Two mechanics worked on a car. The first mechanic worked for 10 hours, and the second mechanic worked for 15 hours. Together they charged a total of 1650 . What was the rate charged per hour by each mechanic if the sum of the two rates was 135 per hour?
Answers
Rates of first and second mechanics are 75 and 60 per hour respectively.
What does an equation mean?A mathematical statement or relation between two or more numeric values, variable and mathematical operations via equal sign is called an Equation.
How to solve equations in Elimination Method?Elimination method is a kind of method to solve simultaneous equations in two variables. In elimination method we compare coefficient of one of two variables and in next step we eliminate that variable using addition or subtraction and form another relation with only one variable and find the value for that one variable and then substituting that value initial equation we can get the value of another variable.
Let the rate of first mechanic and second mechanic be x and y per hour respectively.
Sum of the two rates is 135 per hour. So the suitable equation will be,
x+y = 135 ............... (i)
Since they charged together 1650 after working 10 hours by first and 15 hours by second mechanics. So the suitable equation this time will be,
10x+15y = 1650
5(2x+3y) = 1650
2x+3y = 1650/5
2x+3y = 330 ............... (ii)
Multiplying 2 with equation (i) and then subtracting it from equation (ii) we get,
(2x+3y) - 2(x+y) = 330 - 2*135
2x+3y-2x-2y = 330-270
y = 60
Substituting y=60 in equation (i) we get,
x+60 = 135
x = 135-60 = 75
Hence, the rates charged per hour are 75 and 60 per hour respectively.
To know more about Elimination Method visit:
https://brainly.com/question/25427192
#SPJ1
Related Questions
1. ALUMMUNI PLC. Produces three models of tractors: Metakeb, Mewesson, Metekem Each unit of Metakeb, Mewesson and Metekem requires the following amounts of time in minumtes in each of the indicated departments.
Machining dep't
Inspection dep't
(in minutes)
(in minutes)
(in minutes)
Metakeb
1200
2400
600
Mewesson
1800
1200
3000
Metekem
3000
Assembly dep't
2400
1200
Suppose the total time available per month in machining, assembly and inspection departments are 1050, 1160 and 830 hours respectively.
Required:
Determine the number of units of each product to be produced in a month to use up all the available resources (use Gaussaian method)
Answers
The company should produce 5 units of Metakeb tractors, 8 units of Mewesson tractors, and 16 units of Metekem tractors in a month to use up all the available resources.
What is equations ?
An equation is a mathematical statement that shows that two expressions are equal. It consists of two expressions separated by an equals sign. For example, 3x + 5 = 14 is an equation that states that the sum of 3 times x and 5 is equal to 14.
Let x, y, and z be the number of units of Metakeb, Mewesson, and Metekem tractors produced, respectively.
Then we have the following system of equations based on the time required in each department:
\(1200x + 1800y + 3000z &\leq 63000 \\&&\text{(machining department)}\2400x + 1200y + 1200z &\leq 69600 \\&&\text{(assembly department)}\600x + 3000y &\leq 49800 \\&&\text{(inspection department)}\)
Converting the hours to minutes, we get:
Machining department: 1050 hours = 63,000 minutes
Assembly department: 1160 hours = 69,600 minutes
Inspection department: 830 hours = 49,800 minutes
We can rewrite the system of equations as follows:
\(20x + 30y + 50z &\leq 1050 &&\text{(machining department)}\\ \\10x + 5y + 5z &\leq 580 &&\text{(assembly department)}\\ \\x + 5y &\leq 83 &&\text{(inspection department)}\\ \\\)
To use Gaussian elimination to solve the system of equations, we first write the augmented matrix:
\(\begin{bmatrix}20 & 30 & 50 & | & 1050 \\10 & 5 & 5 & | & 580 \\1 & 5 & 0 & | & 83\end{bmatrix}\)
Performing row operations to obtain row echelon form:
\(\begin{bmatrix}20 & 30 & 50 & | & 1050 \\0 & -55 & -95 & | & -790 \\0 & 1 & -5/4 & | & -1.35\end{bmatrix}\)
Next, perform additional row operations to get the matrix in reduced row echelon form:
\(\begin{bmatrix}20 & 30 & 50 & | & 1050 \\0 & 1 & 19/55 & | & -14.36 \\0 & 0 & 367/55 & | & 105.36\end{bmatrix}\)
Finally, solve for the variables:
\(z &= \frac{105.36}{367/55} = 16 \\ \\y + \frac{19}{55}z &= 14.36 \\ \\y + \frac{19}{55}(16) &= 14.36 \\ \\y &= 8 \\ \\20x + 30y + 50z &= 1050 \\ \\20x + 30(8) + 50(16) &= 1050 \\ \\20x &= 90 \\ \\x &= 4.5\)
Therefore, the company should produce 4.5 units of Metakeb tractors, 8 units of Mewesson tractors, and 16 units of Metekem tractors in a month to use up all the available resources. Since we cannot produce fractional units, we can round up the values of x and y to obtain a feasible production plan of 5 units of Metakeb tractors and 8 units of Mewesson tractors.
To know more about equations visit :
https://brainly.com/question/22688504
#SPJ1
20 students each rolled dice 5 times each to measure the median. Here is the data in the picture attached. What can we infer from this graph by looking at the median data?
A. when rolling a dice multiple times, the median is less likely to fall between numbers 1 and 6.
B. it's not possible to tell the likelihood of where the median is going to be when measuring probability since rolling dice is completely randomized, etc.
C. some other response

Answers
Considering the given graph, we infer that while rolling a dice is random, the median values do exhibit some patterns that can be analyzed and used to make predictions about the expected values.
What is the information from the graph?Looking at the median data from the graph, we can infer that option A is not true, as the median appears to be equally likely to fall between any two numbers from 1 to 6. However, we can also infer that option B is not entirely accurate.
While it is true that rolling a dice is random and each roll is independent, we can observe patterns in the median data.
For example, the median values are more likely to be closer to 3.5, which is the theoretical median value of a fair six-sided dice. This suggests that the more times the dice is rolled, the more likely the median value will approach the expected value.
We can also observe that the spread of the median values tends to decrease as the number of rolls increases, indicating that more rolls lead to more consistent results.
Learn more about probability at: https://brainly.com/question/24756209
#SPJ1
suppose Amaya wants to bake cookies that require 4.5 cups of flour for a recipe that makes 4 dozen cookies. how much flour would Amaya need to make 126 cookies?
Answers
Answer:
11.8125 cups of flour
Step-by-step explanation:
4 dozen = 48 cookies
126/48=2.625 batches of 48 cookies
2.625 x 4.5 = 11.8125 cups of flour
The head of maintenance at XYZ Rent-A-Car believes that the mean number of miles between services is 4959 miles, with a standard deviation of 448 miles. If he is correct, what is the probability that the mean of a sample of 43 cars would differ from the population mean by less than 111 miles
Answers
Answer:
0.8948 = 89.48% probability that the mean of a sample of 43 cars would differ from the population mean by less than 111 miles
Step-by-step explanation:
To solve this question, we need to understand the normal probability distribution and the central limit theorem.
Normal Probability Distribution
Problems of normal distributions can be solved using the z-score formula.
In a set with mean \(\mu\) and standard deviation \(\sigma\), the z-score of a measure X is given by:
\(Z = \frac{X - \mu}{\sigma}\)
The Z-score measures how many standard deviations the measure is from the mean. After finding the Z-score, we look at the z-score table and find the p-value associated with this z-score. This p-value is the probability that the value of the measure is smaller than X, that is, the percentile of X. Subtracting 1 by the p-value, we get the probability that the value of the measure is greater than X.
Central Limit Theorem
The Central Limit Theorem establishes that, for a normally distributed random variable X, with mean \(\mu\) and standard deviation \(\sigma\), the sampling distribution of the sample means with size n can be approximated to a normal distribution with mean \(\mu\) and standard deviation \(s = \frac{\sigma}{\sqrt{n}}\).
For a skewed variable, the Central Limit Theorem can also be applied, as long as n is at least 30.
The mean number of miles between services is 4959 miles, with a standard deviation of 448 miles
This means that \(\mu = 4959, \sigma = 448\)
Sample of 43:
This means that \(n = 43, s = \frac{448}{\sqrt{43}}\)
What is the probability that the mean of a sample of 43 cars would differ from the population mean by less than 111 miles?
p-value of Z when X = 4959 + 111 = 5070 subtracted by the p-value of Z when X = 4959 - 111 = 4848, that is, probability the sample mean is between these two values.
X = 5070
\(Z = \frac{X - \mu}{\sigma}\)
By the Central Limit Theorem
\(Z = \frac{X - \mu}{s}\)
\(Z = \frac{5070 - 4959}{\frac{448}{\sqrt{43}}}\)
\(Z = 1.62\)
\(Z = 1.62\) has a p-value of 0.9474
X = 4848
\(Z = \frac{X - \mu}{s}\)
\(Z = \frac{4848 - 4959}{\frac{448}{\sqrt{43}}}\)
\(Z = -1.62\)
\(Z = -1.62\) has a p-value of 0.0526
0.9474 - 0.0526 = 0.8948
0.8948 = 89.48% probability that the mean of a sample of 43 cars would differ from the population mean by less than 111 miles
1 cat + 20 dogs + 60 men + 5 shark=
Answers
Answer:
Step-by-step explanation:
1 cat + 20 dogs + 60 men + 5 shark= 86
Answer:
86 baby sharks
Step-by-step explanation:
A fish tank has 8 goldfish, 6 tetras, 5 snails, and 2 platies. What is the ratio of tetras to platies?
A. 6/2
B. 2/8
C. 2/6
D. 8/6
Answers
A. 6/2
Eric's father works two part-time jobs, one in the morning and one in the afternoon, and works a total of 40 hours each 5-day workweek. If his schedule is the same each day, and he works 3 hours each morning, how many hours does Eric’s father work each afternoon
does Eric's father work each afternoon?
Answers
Answer:
5
Step-by-step explanation:
ok so first you divide 40 hour by the 5 day work week it = 8 so take away the hours from the morning 8 take away 3 and that = 5 so there is your answer I'm not sure if I did it right but I think I did it just makes sense.
The expression you wrote for part b represents how the jacks and balls can be split among Harold and his friends. What does each number in the expression represent?

Answers
Answer:
6 represents the number of people among which the balls and jacks will be split.
4 represents the number of balls.
10 represents the number of jacks.
Step-by-step explanation:
This is the PLATO answer, hope it helps :)
Answer:
6
Step-by-step explanation:
Referring to the figure, what is the slope of the
line? pls quick

Answers
Answer:
26) 70
27) 12.86 sec
28) 350 feet
Step-by-step explanation:
26) Given points A(0, 0) and B(16, 1120)
slope m = \(\frac{y_2-y_1}{x_2-x_1}\)
\(=\frac{1120-0}{16-0}\\ \\=\frac{1120}{16}\\ \\=70\)
slope = 70
27) eq of a line is :
y = mx + c
y = 70x + c
Since the line passes through A(0,0) substituting the points in the equation,
0 = 70(0) + c
⇒ c = 0
eq of line: y = 70x
given distance is 900 ft
y = distance and x = time
⇒ y = 900
sun in eq,
900 = 70x
⇒ x = 900/70
⇒ x = 12.86
28) time is 5 sec
⇒ x = 5
sub in eq
y = 70(5)
⇒ y = 350
You make $12 an hour, plus time and a half for each hour over 40 hours that you work in a week. How many hours do yo need to work to earn $606?
Answers
Answer:
47 hours
Step-by-step explanation:
[606 -(12×40)]/(1½×12) =
(606-480)/(18) =
126/18 = 7
the total working hours = 40+7 = 47 hours
Answer:
47 hours
Step-by-step explanation:
First, we do 12 times 40, the answer we get is 480 dollars.
Then, we do 606 minus 480, the answer we get is 126.
Next, we do 126 divided by 18, and we get 7
Therefore, the total hours is 47 hours.
consider the sequences 31,35,39,43 ,then which of the following is the first terms of the sequences greater than 312
Answers
Answer:
316 ls the next term greater than 312. in the sequence you are adding 4 to the preceding term
What is the equation of the function represented in the table above?
plz help I really need it please :(
please no link anser

Answers
Take 2 points
(1,10)(2,12)\(\\ \sf\longmapsto m=\dfrac{12-10}{2-1}=2\)
\(\\ \sf\longmapsto y=mx+b\)
\(\\ \sf\longmapsto 10=2(1)+b\)
\(\\ \sf\longmapsto b=8\)
Function:-
\(\\ \sf\longmapsto f(x)=2x+8\)
A circular path 4 feet wide has an inner diameter of 250 feet. How much farther is it around the outer edge of the path than around the inner edge? Round to nearest hundredth. Use 3.14 for π.
The outer edge of the path is ??? feet farther around than the inner edge.
Answers
Answer:
25.13
Step-by-step explanation:
the inner circle is 1570.8 and the outer is 1595.93
Hope this helps! :)
Have a great weekend!! :)
Answer:
50.24 ft
Step-by-step explanation:
diameter = 250 ----- radius = 125 (radius of the smaller circle)
125+4 = 129 ( radius of the bigger circle)
129-125 = 4
4² x pi = 16x 3.14 = 50.24
An extremely large sink hole has opened up in a field just outside of the city limits. It is difficult to measure across the sink hole without falling in so you use congruent triangles. You have one piece of rope that is 50 ft. long and another that is 70 ft. long. You pick a point A on one side of the sink hole and B on the other side. You tie a rope to each spot and pull the rope out diagonally back away from the sink hole so that the other ends of the two ropes meet at point C. Then you recreate the same triangle by using the distance from AC and BC and creating new segments CE and CD. The distance DE is 52.2 ft.
a. What type of triangles have you created?
b. How do you know the triangles are congruent?
c. How far across is the sink hole?
d. What is the perimeter of the triangle ABC?

Answers
A) The type of triangles are congruent triangles
B) By the use of SAS Congruency Postulate
C) The distance across for the sink hole is: 52.2 ft
D) The perimeter of triangle ABC is: 172.2 feet.
How to solve congruent triangles?A) Congruent triangles are defined as the triangles created because of the phrasing "you recreate the same triangle" mentioned in the instructions. Congruent triangles are basically identical carbon copies of each other.
B) If we knew the measure of angle ACB, and then mad use of it to form angle ECD, then we would have enough information to know that triangle ACB was congruent to triangle ECD. Therefore, it would be useful to do the SAS (side angle side) congruence rule.
C) We know that:
AB = ED = 52.2
AB is the distance across the sink hole. Thus, it is 52.2 feet
D) AB = 52.2
BC = 70
AC = 50
Thus:
Perimeter of triangle ABC = AB + BC + AC
Perimeter of triangle ABC = 52.2 + 70 + 50
Perimeter of triangle ABC = 122.2 + 50
Perimeter of triangle ABC = 172.2
The perimeter of triangle ABC is 172.2 feet.
Read more about Congruent Triangles at: https://brainly.com/question/1675117
#SPJ1
Answer:
Step-by-step expA) The type of triangles are congruent triangles
B) By the use of SAS Congruency Postulate
C) The distance across for the sink hole is: 52.2 ft
D) The perimeter of triangle ABC is: 172.2 feet.
How to solve congruent triangles?
A) Congruent triangles are defined as the triangles created because of the phrasing "you recreate the same triangle" mentioned in the instructions. Congruent triangles are basically identical carbon copies of each other.
B) If we knew the measure of angle ACB, and then mad use of it to form angle ECD, then we would have enough information to know that triangle ACB was congruent to triangle ECD. Therefore, it would be useful to do the SAS (side angle side) congruence rule.
C) We know that:
AB = ED = 52.2
AB is the distance across the sink hole. Thus, it is 52.2 feet
D) AB = 52.2
BC = 70
AC = 50
Thus:
Perimeter of triangle ABC = AB + BC + AC
Perimeter of triangle ABC = 52.2 + 70 + 50
Perimeter of triangle ABC = 122.2 + 50
Perimeter of triangle ABC = 172.2
The perimeter of triangle ABC is 172.2 feet.
lanation:
How many different ways can 4 rolls of film be selected from 50 rolls? (Permutation and combination question)
Answers
Answer:
Number of ways to chose rolls = 5,527,200
Step-by-step explanation:
Given:
Total number of films rolls (n) = 50
Selected number of films rolls (r) = 4
Find:
Number of ways to chose rolls.
Computation.
Permutation problem.
Number of ways to chose rolls = n! / (n! - r!)
Number of ways to chose rolls = 50! / (50! - 4!)
Number of ways to chose rolls = 50! / (46!)
Number of ways to chose rolls = 50 × 49 × 48 × 47
Number of ways to chose rolls = 5,527,200
The darkness of the print is measured quantitatively using an index. If the index is greater than or
equal to 2.0 then the darkness is acceptable. Anything less than 2.0 means the print is too light and
not acceptable. Assume that the machines print at an average darkness of 2.2 with a standard
deviation of 0.20.
(a) What percentage of printing jobs will be acceptable? (4)
(b) If the mean cannot be adjusted, but the standard deviation can, what must be the new standard
deviation such that a minimum of 95% of jobs will be acceptable?
Answers
84.13% of the printing jobs will be acceptable.
The new standard deviation required to achieve a minimum of 95% of jobs acceptable is 0.121.
The darkness of the print is measured quantitatively using an index. If the index is greater than or equal to 2.0 then the darkness is acceptable. Anything less than 2.0 means the print is too light and not acceptable. The machines print at an average darkness of 2.2 with a standard deviation of 0.20.
The mean of the darkness of the print is µ = 2.2 and the standard deviation is σ = 0.20.Therefore, the z-score can be calculated as; `z = (x - µ) / σ`.The index required for acceptable prints is 2.0. Thus, the percentage of prints that are acceptable can be calculated as follows;P(X ≥ 2.0) = P((X - µ)/σ ≥ (2.0 - 2.2) / 0.20)P(Z ≥ -1) = 1 - P(Z < -1)Using the standard normal table, P(Z < -1) = 0.1587P(Z ≥ -1) = 1 - 0.1587= 0.8413.
To find the new standard deviation, we can use the z-score formula.z = (x - µ) / σz = (2.0 - 2.2) / σz = -1Therefore, P(X ≥ 2.0) = 0.95P(Z ≥ -1) = 0.95P(Z < -1) = 0.05Using the standard normal table, the z-score value of -1.645 corresponds to a cumulative probability of 0.05. Hence,z = (2.0 - 2.2) / σ = -1.645σ = (2.0 - 2.2) / -1.645= 0.121.
for such more question on deviation
https://brainly.com/question/475676
#SPJ8
Find the solution of 5/2x - 7 = 3/4x + 14
What is equal to x?
Answers
Answer:
Ameliorate retort, pertaining to preceding albeit contingent interrogate proximates subject to x = 12.
Step-by-step explanation:
Availing, exploiting, albeit maneuvering the Mathematic convention, Order of Operations (OO), the contiguous albeit proximate equation may ascertain as the preeminent imminent:
Groups: Not identified.
Exponents: Not identified, disparaging ₁.
Multiplication: Derived, pertaining to subtraction, 4 · (7/4x) = 4 · 21 7x = 84
Division: Derived, pertaining to multiplication, 7x = 84 ⇒ x = 12
Addition: Aggregate constants ⇒ 5/2x = 3/4x +21
Subtraction: Abate algebraic groups ⇒ 7/4x = 21
An arrow is shot at an angle 32 degree from the horizontal. If it's height is increasing at 200 km/hr, how fast is the arrow itself going?
Answers
Check the picture below.
Bearing in mind that the angle is not changing all the wihle, namely is constant
\(tan(32^o)=\cfrac{y}{x}\implies x = \cfrac{y}{tan(32^o)}\implies x = \cfrac{1}{tan(32^o)}y \\\\\\ \stackrel{\textit{taking the derivative on bot sides}}{\cfrac{dx}{dt}=\cfrac{1}{tan(32^o)}\cfrac{dy}{dt}}\implies \cfrac{dx}{dt}=\cfrac{1}{tan(32^o)}200\implies \cfrac{dx}{dt}\approx 320.07~\frac{km}{hr}\)

Question 16 of 25Which of the following is an example of discrete data?A. The weights of students in a classB. Average daily temperatures in a cityC. The wing length of a birdD. The number of goals scored by each member of a soccer teamSUBMIT
Answers
Answer
D. The number of goals scored by each member of a soccer team.
Explanation
Discrete data is information that can only take certain values. That is discrete data are data that can be counted and involves integers. In order words, only a limited number of values can be assigned to the data.
Examples of discrete data are variables that are numeric, non-negative numbers, countable, and finite.
Therefore, an example of discrete data that is finite and countable is: D. The number of goals scored by each member of a soccer team.
what is the property of 3x(5x7)=(3x5)7
Answers
The property you are referring to is called the associative property of multiplication. According to this property, when multiplying three numbers, the grouping of the numbers does not affect the result. In other words, you can change the grouping of the factors without changing the product.
In the equation you provided: 3x(5x7) = (3x5)7
The associative property allows us to group the factors in different ways without changing the result. So, whether we multiply 5 and 7 first, or multiply 3 and 5 first, the final product will be the same.
What is | -8|
—16
-8
8
16
Answers
Answer:
8
Step-by-step explanation:
scince it has the lines that mean that it will always be positive.
Answer:
8
Step-by-step explanation:
Have a nice day!
The point P=(1/2,y)lies on the unit circle shown below. What is the value of y in simplest form?
Answers
The value of y in simplest form for the point P = (1/2, y) lying on the unit circle is y = ± √(3)/2.
To find the value of y in simplest form for the point P = (1/2, y) lying on the unit circle, we can use the equation of the unit circle, which states that for any point (x, y) on the unit circle, the following equation holds: x^2 + y^2 = 1.
Plugging in the coordinates of the point P = (1/2, y), we get:
(1/2)^2 + y^2 = 1
1/4 + y^2 = 1
y^2 = 1 - 1/4
y^2 = 3/4.
To simplify y^2 = 3/4, we take the square root of both sides:y = ± √(3/4).
Now, we need to simplify √(3/4). Since 3 and 4 share a common factor of 1, we can simplify further: y = ± √(3/4) = ± √(3)/√(4) = ± √(3)/2.
for more search question point
https://brainly.com/question/28162977
#SPJ8
consider the system of linear equations
consider the system of linear equations
6x+2y – z=4
X +5y+z=3
2x+y+4z=27
A, solve the system by
I. Gassian elimination method,
II. LU- decomposition method
III. Gauss- Jacobi method,and
IV. Gauss-seidel method,
Answers
I. The solution to the system of equations using Gaussian elimination is x = 1, y = -1, and z = 2.
II. For the LU-decomposition method, we need to have a square coefficient matrix, which is not the case in the given system. Therefore, we cannot directly apply the LU-decomposition method.
III. For this method to converge, the coefficient matrix must be diagonally dominant, which is not the case in the given system. Therefore, the Gauss-Jacobi method cannot be directly applied either.
IV. It requires the coefficient matrix to be diagonally dominant, which is not satisfied in the given system. Hence, the Gauss-Seidel method cannot be directly used.
I. Gaussian Elimination Method:
To solve the system of linear equations using Gaussian elimination, we perform row operations to reduce the system into upper triangular form. The augmented matrix for the given system is:
| 6 2 -1 | 4 |
| 1 5 1 | 3 |
| 2 1 4 |27 |
We can start by eliminating the coefficients below the first element in the first column. To do this, we multiply the first row by a suitable factor and subtract it from the second and third rows to eliminate the x coefficient below the first row. Then, we proceed to eliminate the x coefficient below the second row, and so on.
After performing the necessary row operations, we obtain the following reduced row-echelon form:
| 6 2 -1 | 4 |
| 0 4 2 | -1 |
| 0 0 3 | 6 |
From this form, we can easily back-substitute to find the values of x, y, and z. We have z = 2, y = -1, and x = 1.
II. LU-Decomposition Method:
LU-decomposition is a method that decomposes a square matrix into a product of two matrices, L and U, where L is lower triangular and U is upper triangular.
III. Gauss-Jacobi Method:
The Gauss-Jacobi method is an iterative numerical method to solve systems of linear equations.
IV. Gauss-Seidel Method:
Similar to the Gauss-Jacobi method, the Gauss-Seidel method is an iterative method for solving linear systems.
Therefore, out of the four methods mentioned, only the Gaussian elimination method can be used to solve the given system of linear equations.
For more such questions on solution
https://brainly.com/question/24644930
#SPJ8
Help me this is time sensitive so please help
⭐️⭐️⭐️⭐️⭐️

Answers
The probability of rolling a total of 9 is 1/9.
The probability of rolling a total of 10 is 1/12.
The probability of rolling a total of 11 is 1/18.
We have,
To calculate the probabilities of rolling specific totals with a pair of dice, we need to consider all possible combinations of numbers on each die.
Probability of rolling a total of 9:
There are four combinations that can result in a total of 9: (3, 6), (4, 5), (5, 4), and (6, 3).
Since each die has six sides, there are 6 * 6 = 36 equally likely outcomes when rolling two dice.
The probability of rolling a total of 9 is 4/36, which can be simplified to 1/9.
Probability of rolling a total of 10:
There are three combinations that can result in a total of 10: (4, 6), (5, 5), and (6, 4).
The probability of rolling a total of 10 is 3/36, which can be simplified to 1/12.
Probability of rolling a total of 11:
There are two combinations that can result in a total of 11: (5, 6) and (6, 5).
The probability of rolling a total of 11 is 2/36, which can be simplified to 1/18.
Thus,
The probability of rolling a total of 9 is 1/9.
The probability of rolling a total of 10 is 1/12.
The probability of rolling a total of 11 is 1/18.
Learn more about probability here:
https://brainly.com/question/14099682
#SPJ1
Find the value of x such that the line containing (1,2) and (5,3) is perpendicular to the line containing (x,4) and (3,0)
Answers
The value of x that makes the line containing (1,2) and (5,3) perpendicular to the line containing (x,4) and (3,0) is x = 2.
To determine the value of x such that the line containing (1,2) and (5,3) is perpendicular to the line containing (x,4) and (3,0), we need to find the slope of both lines and apply the concept of perpendicular lines.
The slope of a line can be found using the formula:
slope = (change in y) / (change in x)
For the line containing (1,2) and (5,3), the slope is:
slope1 = (3 - 2) / (5 - 1) = 1 / 4
To find the slope of the line containing (x,4) and (3,0), we use the same formula:
slope2 = (0 - 4) / (3 - x) = -4 / (3 - x)
Perpendicular lines have slopes that are negative reciprocals of each other. In other words, if the slope of one line is m, then the slope of a line perpendicular to it is -1/m.
So, we can set up the equation:
-1 / (1/4) = -4 / (3 - x)
Simplifying this equation:
-4 = -4 / (3 - x)
To remove the fraction, we can multiply both sides by (3 - x):
-4(3 - x) = -4
Expanding and simplifying:
-12 + 4x = -4
Adding 12 to both sides:
4x = 8
Dividing both sides by 4:
x = 2
For more such questions on line.
https://brainly.com/question/280216
#SPJ8
A student compiled a list of daily low temperatures (in °F) that she observed at her college last semester. The
coldest temperature for any day was 31°F, but she mistakenly recorded this as 13º. How will this error affect the
following summary statistics?
a) The mean will
be higher
and the median will
b) The standard deviation will be lower.
be higher.
be lower
not be affected.
Answers
If l || m, find the value of each missing variable(s).
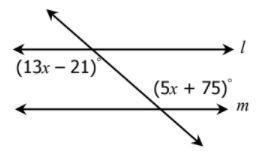
Answers
Answer:
12
Step-by-step explanation:
13x-21=5x+75
8x=96
x=96/8=12
Answer:
x=12
Step-by-step explanation:
Since the 2 lines are parallel we can use the rules of transversals. These 2 angels are alternate side interior. This means that they are congruent and have the same measurement. Because they have the same measurement, we can set them equal to each other and solve for x.
First, set the expressions equal to each other13x-21 = 5x+75
Next, add 21 to both sides13x = 5x+96
Then, subtract 5x from both sides8x = 96
Finally, divide both sides by 8x=12
A commercial jet can fly 1,320 miles in 3 hours with a tailwind but only 1,170 miles in 3 hours into a headwind. Find the speed of the jet in still air and the speed of the wind.
Answers
Answer:
Jet: 415 mph
Wind: 25 mph
Step-by-step explanation:
If x is the speed of the jet in still air, and y is the speed of the wind, then:
x + y = 1320 / 3 = 440
x − y = 1170 / 3 = 390
Solve the system of equations with substitution or elimination. Using elimination:
2x = 830
x = 415
y = 25
Determine which translations would map Figure
W onto Figure X
Answers
The sequence of steps that translates figure W to figure X are -
It is first shifted by the rule (x, y) → (x, y - 3)The image is then reflected across the y - axis.The image is then reflected across the x - axis.Refer to the image attached. It shows figure W and figure X. We can write the sequence of steps for the given translation as -
It is first shifted by the rule (x, y) → (x, y - 3)The image is then reflected across the y - axis.The image is then reflected across the x - axis.To solve more questions on translations, visit the link-
https://brainly.com/question/11805053
#SPJ1

A Realtor claims that no more than half of the homes he sells are sold for less than the asking price. When reviewing a random sample of 14 sales over the past year, he found that actually 10 were sold below the asking price.
Required:
a. The assumption of normality is justified.
b. Calculate a p-value for the observed sample outcome, using the normal distribution.
c. At the 0.05 level of significance in a right-tailed test, is the proportion of homes sold for less than the asking price greater than 50%?