Determine the equation of the line that passes through the points (-2,3) and (4,15)
Answers
We are required to find the equation of a straight line passing through two given points .
First we can calculate the gradient / slope
\(\begin{gathered} x_1=-2 \\ y_1=3 \\ x_2=4 \\ y_2=15 \end{gathered}\)Calculating slope(m) below
\(m=\frac{y_2-y_1}{x_2-x_1}\)\(\begin{gathered} m=\frac{15-3}{4-(-2)} \\ m=\frac{12}{6} \\ m=2 \end{gathered}\)Using the formula y = mx + c at the point any of the two given points .... we can use ( 4 , 15 ) ... we have
\(\begin{gathered} y=2x+c \\ x=4,y=15 \\ 15=2(4)+c \\ 15=8+c \\ c=7 \end{gathered}\)The required straight line equation will be obtained using y=mx+c as;
\(y=2x+7\)Here is a graph of the line
Hence the equation of the line is y=2x + 7

Related Questions
Please help this is Geometry

Answers
Answer:
solution given:
volume of lower one[V1]=l×b×h=1.5×0.5×0.5=0.378m³
volume of upper one [V2]=l×b×h=1×0.5×(0.75-0.5)=
0.125m³
total volume=V1+V2=0.378m³+0.125m³=0.503m³
0.503m³ is your answer
Solve for x. 7.5x/11=4.8/5
Answers
The value of the variable is 1. 40
How to determine the valuewe need to know that algebraic expressions are described as expressions that are made up of term, variables, constants, factors and coefficients.
these algebraic expressions are also made up of arithmetic operations.
These arithmetic operations are listed as;
BracketDivisionAdditionSubtractionMultiplicationParenthesesFrom the information given, we have that;
7.5x/11=4.8/5
cross multiply the values, we get;
7.5x(5) = 4.8(11)
multiply the values
37. 5x = 52. 8
Divide both sides by the coefficient of x, we get;
x = 1. 40
Learn about algebraic expressions at: https://brainly.com/question/4541471
#SPJ1
Joy saved up her money and bought a model train. Each train car is of a foot long. When
it's put together, the whole train is 4 feet long. How many cars are in the train?
Write your answer as a fraction or a whole number.
Submit
Yo
Answers
The number of cars used to make a train of length 4 feet is 4 cars.
What is Unitary Method?The unitary technique involves first determining the value of a single unit, followed by the value of the necessary number of units.
For example, Let's say Ram spends 36 Rs. for a dozen (12) bananas.
12 bananas will set you back 36 Rs. 1 banana costs 36 x 12 = 3 Rupees.
As a result, one banana costs three rupees. Let's say we need to calculate the price of 15 bananas.
This may be done as follows: 15 bananas cost 3 rupees each; 15 units cost 45 rupees.
Given:
Each train car is of 1 foot long.
and, When it's put together, the whole train is 4 feet long.
So, the number of cars needed is
= 4/ 1
= 4 cars
So, That makes 1 +1 + 1 +1 = 4 feet = length of Train.
Learn more about Unitary Method here:
https://brainly.com/question/22056199
#SPJ9
PLS HELP FOR 40 POINTS I HAVE AN EXAM!!!
You need to select a pair of socks from the draw and then a t-shirt. You have eight different colors of sock pairs and ten different color t-shirts. If you pull one from each draw, what are the possible combinations of socks and t-shirt that you will get?
Answers
Answer:
8
Step-by-step explanation:
You only have 8 socks and its a combination of both socks and t-shirts. Hope this helps!
Answer:
80
Step-by-step explanation:
10*8=80
Lisa and Susan are driving to college together. They look at a map to find out how far they have to drive. On the map, Lisa measures the distance to be 4.5 inches. How many miles do they have to drive if the map scale is 1 in. = 35 mi?
Answers
Answer:157.5
Step-by-step explanation:becuase mutilpy 4.5x35 that equals 157.5
Determine the quadratic inequality that produces the graph below.

Answers
Explanation
Since the line is a solid line it’s an inequality ≥ or ≤ so we can eliminate < or > answers #2 and #3
The shaded area is to the outside of the parabola so we know y is ≥ greater than or equal to the line so this eliminates #4 that is ≤ less than or equal to sign
So our correct answer is #1.
y ≥ -x^2 + x + 6

Can someone please help, ty!!
(Will mark brainliest)

Answers
Might be wrong
Answer:
The slope is 4/5 (blue) because if you go up 4 and right 5 from the dot at (-1,-2), you would be at the next dot which is at (4,2)
Step-by-step explanation:
Hope this helps and pls do mark me brainliest if you can:)
The temperature fell 3.25 degrees every hour. Find the change in temperature after 5 hours
Answers
Answer:
The temperature would fall by 16.25°
Step-by-step explanation:
If it's 3.25 every hour and there are 5 hours total just multiply. 3.25 x 5 = 16.25.
complete the partial two-way frequency table below that shows the extracurricular activities of high school students. Based on the data in the table, how many students do not play an instrument
Answers
According to the information, the missing number from the box is number 40.
How to find the missing number in the box?To find the missing number of the table we must take into account different elements. In particular we must look at the totals and the columns and rows in which the empty space is. Once we identify the totals we can subtract the other values from the total and find the missing number in the table.
According to the above we can infer that the missing number is 40.
Learn more about numbers in: https://brainly.com/question/24908711
#SPJ1

homework help on ratios Triangle CDE is similar to triangle GHK and the similarity ratio is 1/16. If DE=20, find the length of HK.
Answers
The similarity ratio expresses the ratio between one side of the first triangle and its corresponding side on the second triangle. To find HK use this information.
\(\begin{gathered} \frac{1}{16}=\frac{20}{HK} \\ HK=20\cdot16 \\ HK=320 \end{gathered}\)The length of HK is 320.
How do you multiply fractions? Please explain step by step to get marked
Answers
Answer: 10/3
Step-by-step explanation
2/3x5
2/3x5/1
Now goahead and multiply the top and bottom numbers
6x +6 = -10 + 8x
please solve it fast
Answers
Answer:
x = 8
General Formulas and Concepts:
Pre-Algebra
Order of Operations: BPEMDAS
Brackets Parenthesis Exponents Multiplication Division Addition Subtraction Left to RightEquality Properties
Multiplication Property of Equality Division Property of Equality Addition Property of Equality Subtraction Property of EqualityAlgebra I
Terms/CoefficientsStep-by-step explanation:
Step 1: Define
6x + 6 = -10 + 8x
Step 2: Solve for x
[Subtraction Property of Equality] Subtract 6x on both sides: 6 = -10 + 2x[Addition Property of Equality] Add 10 on both sides: 16 = 2x[Division Property of Equality] Divide 2 on both sides: 8 = xRearrange/Rewrite: x = 8Step 3: Check
Plug in x into the original equation to verify it's a solution.
Substitute in x: 6(8) + 6 = -10 + 8(8)Multiply: 48 + 6 = -10 + 64Add: 54 = -10 + 64Add: 54 = 54Here we see that 54 does indeed equal 54.
∴ x = 8 is the solution to the equation.
Find the value of n for which the division of x^2n-1 by x+3 leave remainder of -80.
Answers
The value of 'n' for which the division of x^(2n-1) by x + 3 leaves a remainder of -80 is n = 1.
To find the value of 'n' for which the division of x^(2n-1) by x + 3 leaves a remainder of -80, we can use polynomial long division. Let's perform the division step by step:
Write the dividend and divisor in polynomial long division format:
_________________________
x + 3 │ x^(2n-1) + 0x^(2n-2) + 0x^(2n-3) + ...
Divide the leading term of the dividend (x^(2n-1)) by the leading term of the divisor (x). The result is x^(2n-1)/x = x^(2n-2).
Multiply the divisor (x + 3) by the quotient obtained in the previous step (x^(2n-2)). The result is x^(2n-2) * (x + 3) = x^(2n-1) + 3x^(2n-2).
Subtract the result obtained in step 3 from the original dividend:
x^(2n-1) + 0x^(2n-2) + 0x^(2n-3) + ... - (x^(2n-1) + 3x^(2n-2)) = -3x^(2n-2) + 0x^(2n-3) + ...
Bring down the next term of the dividend (which is 0x^(2n-3)) and repeat steps 2-4 until the remainder is constant.
Since we are given that the remainder is -80, we can set the remainder equal to -80 and solve for 'n'.
-3x^(2n-2) + 0x^(2n-3) + ... = -80
Since the remainder is constant (-80), it means that all the terms with x have been canceled out in the division process. Therefore, we can deduce that the highest power of x in the divisor (x + 3) is 0.
This implies that x^(2n-2) = 0, and for any value of 'n', the exponent 2n-2 should be equal to zero. Solving this equation:
2n-2 = 0
2n = 2
n = 1
Therefore, the value of 'n' for which the division of x^(2n-1) by x + 3 leaves a remainder of -80 is n = 1.
for such more question on remainder
https://brainly.com/question/825875
#SPJ8
What Pythagorean triple is made using the Pythagorean identity and the following numbers?
x = 2, y = 1
1. 3, 4, 5
2. 4, 1, 25
3. 2, 1, 2
4. 4, 1, root 5.
Answers
The Pythagorean triples here are; 3, 4, 5. Option 1
What is a Pythagorean triple?We define a Pythagorean triple as a^2+b^2 = c^2 where a, b and c are the three positive integers. Given that we can find the Pythagorean triple from; (x2 - y2)2 + (2xy)2 = (x2 + y2)2
Where; x = 2, y = 1
Substituting values have;
(2^2 - 1^2)^2 + (2*2*1)^2 = (2^2 + 1^2)^2
This results to;
3^2 + 4^2 = 5^2
9 + 16 = 25
25=25
Therefore the Pythagorean triples here are; 3, 4, 5. Option 1
Learn more about Pythagorean triples:https://brainly.com/question/15190643
#SPJ1
An AP has first term as 3 and Common difference of 2 how many terms are needed to make the sum to 99
Answers
Answer:
9
Step-by-step explanation:
The \(n\)term is \(2n+1\).
\(S_n=\frac{3+2n+1}{2}(n)=99 \\ \\ \frac{n(2n+4)}{2}=99 \\ \\ n(n+2)=99 \\ \\ n^2+2n-99=0 \\ \\ (n+11)(n-9)=0 \\ \\ n=9 \text{ } (n>0)\)
The number of terms that needed to make the sum to 99 is 9
The first term of the arithmetic progression = 3
The common difference = 2
The sum of n term is = (n/2) [2a+(n-1)d]
Where a is the initial term
d is the common difference
Substitute the values in the equation
(n/2) [2(3)+(n-1)2] = 99
(n/2) [6 + 2n - 2] = 99
(n/2)[4+2n] = 99
n(2 + n) = 99
2n + \(n^2\) = 99
\(n^2\) + 2n - 99 = 0
Split the terms
\(n^2\) - 9n +11n - 99 =0
n(n -9) + 11(n - 9) = 0
(n + 11)(n - 9) = 0
n = -11 or 9
Since n cannot be a negative number, therefore n = 9
Hence, the number of terms that needed to make the sum to 99 is 9
Learn more about sum of n terms here
brainly.com/question/11688485
#SPJ1
Mr. Cho received a container of fresh eggs. He sold 1/3
of the eggs in the
morning and sold 320 eggs in the afternoon. At the end of the day, he
found that 1/4
of the eggs were not sold. How many eggs did he receive in
the beginning?
Answers
Mr. Cho received 3840 eggs in the beginning.
Let's assume that Mr. Cho received x eggs in the beginning.
In the morning, he sold 1/3 of the eggs, so he had 2/3 of the eggs left. Therefore, he had 2/3 x eggs left after the morning sales.
In the afternoon, he sold 320 eggs, so he had 2/3 × x - 320 eggs left at the end of the afternoon.
At the end of the day, he found that 1/4 of the eggs were not sold, which means that he had 3/4 of the eggs left. Therefore, we have:
3/4 × x = 2/3 × x - 320
Multiplying both sides by 12, we get:
9x = 8x - 3840
Simplifying, we get:
x = 3840
Therefore, Mr. Cho received 3840 eggs in the beginning.
for such more question on eggs
https://brainly.com/question/25863198
#SPJ11
The diagonal of a square table is 3.5 feet.
What is the length of a side of the table? Round to the nearest tenth.
Answers
Answer:
easy it 12.3
Step-by-step explanation:
just took the quiz
The graphs are the shame shape but what is the equation of the red line?
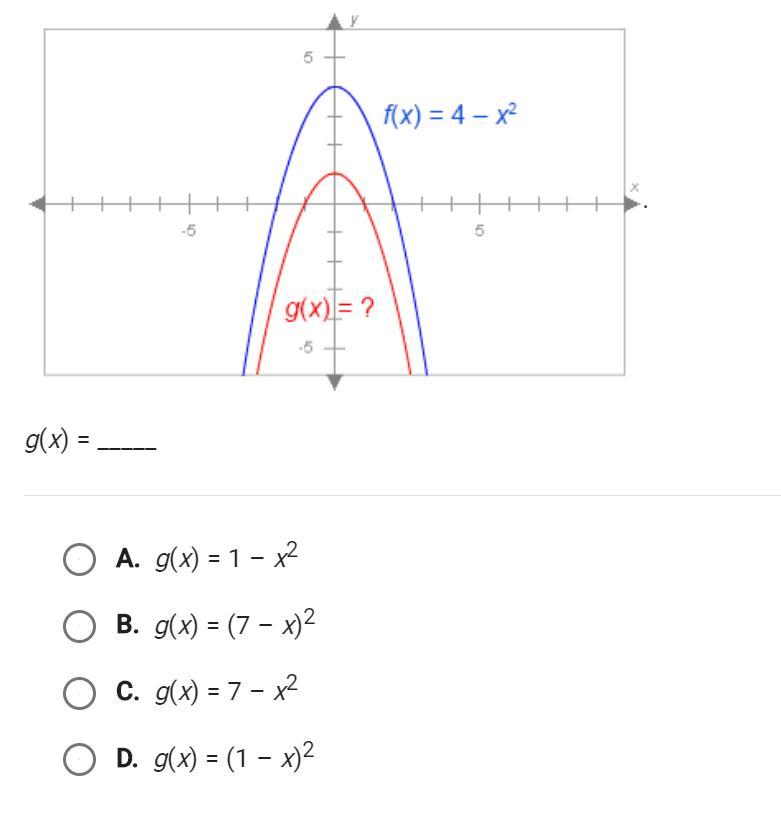
Answers
Answer:
A
Step-by-step explanation:
Since the shape and vertex x coordinate of both graphs are the same, the only difference is the vertical displacement. Since the blue graph is 4 above the origin and the red graph is 1 above the origin, its graph is:
\(g(x)=1-x^2\)
Hence, the correct answer is choice A. Hope this helps!
Please use the following for the next 6 questions. Suppose that the average weekly earnings for employees in general automotive repair shops is $450, and that the population standard deviation for the earnings for such employees is $50. A sample of 100 such employees is selected at random.
1) What is the probability distribution of the average weekly earnings for employees in general automotive repair shops?
2) Find the probability that the average weekly earnings is less than $445.
3) Find the probability that the average weekly earnings is exactly equal to $445.
4) Find the probability that the average weekly earnings is between $445 and $455.
5) In answering the previous 3 questions, did you have to make any assumptions about the population distribution?
6) Now assume that the weekly earnings for employees in all general automotive repair shops is normally distributed, obtain the probability that a given employee will earn more than $480 in a given week.
Answers
1) The probability distribution of the average weekly earnings for employees in general automotive repair shops is the sampling distribution of the sample mean. According to the Central Limit Theorem, if the sample size is large enough, the sampling distribution of the sample mean is approximately normal, with a mean equal to the population mean and a standard deviation equal to the population standard deviation divided by the square root of the sample size.
2) To find the probability that the average weekly earnings is less than $445, we can standardize the sample mean and use a z-table. The z-score for $445 is calculated as follows: z = (445 - 450) / (50 / sqrt(100)) = -1. Using a z-table, we find that the probability that the average weekly earnings is less than $445 is approximately 0.1587.
3) Since we are dealing with a continuous distribution, the probability that the average weekly earnings is exactly equal to any specific value is zero.
4) To find the probability that the average weekly earnings is between $445 and $455, we can subtract the probability that it is less than $445 from the probability that it is less than $455. The z-score for $455 is calculated as follows: z = (455 - 450) / (50 / sqrt(100)) = 1. Using a z-table, we find that the probability that the average weekly earnings is less than $455 is approximately 0.8413. Therefore, the probability that it is between $445 and $455 is approximately 0.8413 - 0.1587 = 0.6826.
5) In answering questions 2-4, we made an assumption about the population distribution based on the Central Limit Theorem. We assumed that since our sample size was large enough (n=100), our sampling distribution would be approximately normal.
6) If we assume that weekly earnings for employees in all general automotive repair shops are normally distributed with a mean of $450 and a standard deviation of $50, then we can calculate the z-score for an employee earning more than $480 in a given week as follows: z = (480 - 450) / 50 = 0.6. Using a z-table, we find that the probability that an employee will earn more than $480 in a given week is approximately 1 - 0.7257 = 0.2743.
What is the sum of 43 and z is equal to 72.98.
Answers
Answer:
x + 43 = 72.98
- 43 - 43
x = 29.98
Step-by-step explanation:
Estimate 4 1/3 x 2 3/4
Answers
Answer:
11 11/12
Step-by-step explanation:
Answer:
≈ 12
Step-by-step explanation:
To estimate, we'll round these mixed numbers to the nearest whole number, then multiply. Let's start with 4 1/3. 4 1/3 is closest to 4, since 1/3 is smaller than 1/2. Then, we can look at 2 3/4. This mixed number is closest to 3, since 3/4 is greater than 1/2.
Now, the two numbers we have are 4 and 3. What is 4 × 3? Well, that's pretty simple - the answer is 12. So, 4 1/3 × 2 3/4 ≈ 12. Hopefully that's helpful! :)
6. Which of the following equations represents the data in the table?
Х
-4
0
4
8
y
5
2
-1
-4
O A. y = x + 2
O B. y = -x + 2
oc. y = x + 8
OD. y = -
3 + 8
M

Answers
Answer:
Step-by-step explanation:
6. Which of the following equations represents the data in the table?
Х
-4
0
4
8
y
5
2
-1
-4
O A. y = x + 2
O B. y = -x + 2
oc. y = x + 8
OD. y = -
3 + 8
M
help plzzzzzzzzzzzzzzzzzzzzz
A jar contains 20 yellow jellybeans, 20 orange jellybeans, 20 red jellybeans and 20 green jellybeans.
Required:
a. In how many ways can you put all the jellybeans in a row?
b. How many ways are there to select a handful of 20 jellybeans?
c. How many ways are there to select a handful of 20 jellybeans that contains at least 3 red?
d. How many ways are there to select a handful of 20 jellybeans that contains at least 3 red and at most 2 orange?
Answers
Answer:
A)
\(\left \{ {{80} \atop {20}} \} * \left \{ {{60} \atop {20}} \} * \left \{ {{40} \atop {20}} \} * \left \{ {{20} \atop {20}} \}\)
B)
\(\left \{ {{20+4-1} \atop {4-1}} \} = \left \{ {{23} \atop {3}} \}\)
C)
= \(\left \{ {{17+4+1} \atop {4-1}} \} = \left \{ {{20} \atop {3}} \}\)
D)
= \(\left \{ {{20} \atop {3}} \} - \left \{ {{17} \atop {3}} \}\)
Step-by-step explanation:
A) How many ways can you put all Jellybeans in a row
Total number of Jellybeans = 80
The first jellybeans = 20 yellow , second is 20 orange jellybeans , third is 20 red jellybeans , fourth is 20 green jellybeans
Therefore the number of ways the Jellybeans can be put in a row is :
\(\left \{ {{80} \atop {20}} \} * \left \{ {{60} \atop {20}} \} * \left \{ {{40} \atop {20}} \} * \left \{ {{20} \atop {20}} \}\)
B) How many ways are there to select a handful of 20 jellybeans
lets assume:
yellow jellybeans = a , orange jellybeans = b , red jellybeans = c , green jellybeans = d
a + b + c + d = 20
This is the number Non-negative integer solutions
= \(\left \{ {{20+4-1} \atop {4-1}} \} = \left \{ {{23} \atop {3}} \}\)
C) This is also the number of Non-negative integer solutions but in this case the value of C ≥ 3
hence the number of ways to select a handful of 20 jellybeans that contains at least 3 red
= \(\left \{ {{17+4+1} \atop {4-1}} \} = \left \{ {{20} \atop {3}} \}\)
D) In this case the value of C ≥ 3 and B ≤ 2
Hence the number of ways to select a handful of 20 jellybeans that contains at least 3 red and at most 2 orange
= \(\left \{ {{20} \atop {3}} \} - \left \{ {{17} \atop {3}} \}\)
At a gas station, 50% of the customers use regular gas, 30% use mid-grade gas and 20% use premium gas. Of those customers using regular gas, only 30% fill their tanks. Of those customers using mid-grade gas, 60% fill their tanks, whereas of those using premium, 50% fill their tanks. What is the probability that the next customer will request mid-grade gas and fill the tank
Answers
Answer:
The probability that the next customer will request mid-grade gas and fill the tank is 0.1800
Step-by-step explanation:
In order to calculate the probability that the next customer will request mid-grade gas and fill the tank we would have to make the following calculation:
probability that the next customer will request mid-grade gas and fill the tank= percentage of the people using mid-grade gas* percentage of the people using mid-grade gas that fill their tanks
probability that the next customer will request mid-grade gas and fill the tank= 30%*60%
probability that the next customer will request mid-grade gas and fill the tank= 0.1800
The probability that the next customer will request mid-grade gas and fill the tank is 0.1800
if a student is selected at random, find the probability the student is a junior. round to the nearest whole percent! i’m not good at probability so an explaination would be appreciated!!!

Answers
Answer:
the answer is 40%.
Step-by-step explanation:
probability= total number of juniors all over total number.
that is , 8
20
2
5
rounding it the nearest whole percent is
2 × 100
5
40%.
The probability that the selected student is a junior is 27%.
What is the probability of an event?The probability of an event is the ratio of the number of outcomes favorable to the event to the total number of outcomes for the event. This represents the chance for the event to take place.
If A is the event, n is the number of outcomes favorable to the event and S is the total number of outcomes, then the probability of the event A is given as:
P(A) = n/S.
How do we solve the given question?We are given a two-way table showing the number of freshmen, sophomores, juniors, and seniors in the class with respect to their gender.
We are asked to find the probability of selecting a junior student while picking a random student from the class.
Let the event of selecting a junior student from the class be A.
Number of favorable outcomes to event A (n) = 2 + 6 = 8 (junior males + junior females)
Total number of outcomes (students in the class) (S) = 4 + 6 + 2 + 2 + 3 + 4 + 6 + 3 = 30.
∴ The probability that the selected student is a junior is given by:
P(A) = n/S = 8/30
or, P(A) = (8/30)*100% = 26.67% = 27% (rounding to the nearest whole percent).
∴ The probability that the selected student is a junior is 27%.
Learn more about the probability of an event at
https://brainly.com/question/7965468
#SPJ2
Two towns are 1150 miles apart. A group of hikers starts from each town and walks down the trail toward each other. They meet after a total hiking time of 220 hours. If one group travels 1 1/2 mile per hour slower than the other group, find the rate of each group.
The rate of the slower group is?
The rate of the faster group is?
Answers
The rate of each group was 1.86 mph and 3.36 mph.
Given that the two towns are 1150 miles apart.
A group of hikers from each town meet after a total hiking time of 220 hours.
One group travels 1 1/2 mile per hour slower than the other group,
We need to find the rate of each group,
So, let use consider, distance travelled by one group = x miles,
Therefore, second group travelled = 1150 - x miles.
Rate of first group = y mph
Rate of second group = y - 3/2 mph
Rate = distance / time
So,
y = x / 220
x = 220y.............(i)
Similarly,
y-3/2 = 1150 - x / 220
220y - 330 = 1150 - x
Using eq (i)
220y - 330 = 1150 - 220y
440y = 1480
y = 3.36
y - 3/2 = 1.86
Hence the rate of each group was 1.86 mph and 3.36 mph.
Learn more about speed click;
https://brainly.com/question/17661499
#SPJ1
The table represents a linear function. Find the missing values to complete the table.
A) x = 1, y=17/3
B) x= 1, y = 21/3
C) x = 5, y = 17/3
D) x = 5, y = 21/3

Answers
The value of missing x = 5
The value of missing y = 17 / 3
How to find the Slope ?The standard line equation, y = mx + b, or the slope formula, m = (y2 - y1)/(x2 - x1), can both be used to find the slope, m.
According to the given information
Let two points be \((x_{1} , y_{1} )\) and \((x_{2} , y_{2} )\) are ( 3 , 8 ) and ( 6 , 15 )
Slope of the two points = \(\frac{y_{2}- y_{1} }{x_{2} -x_{1} }\)
= \(\frac{15-8}{6-3}\)
= 7 / 3
Let the equation passing through points ( 3 , 8 ) be
\(( y - y_{1}) = m(x - x_{1})\)
y - 8 = 7/3( x - 3 )
3y - 24 = 7x - 21
3y = 7x - 21 + 24
3y = 7x + 3
When x = 2
3y = 14 + 3
y = 17 / 3
When y = 38/5
114/3 = 7x + 3
7x = 114/3 - 3
21x = 114 - 9
x = 5
So
The value of missing x = 5
The value of missing y = 17 / 3
To know more about Slope
https://brainly.com/question/6328621
#SPJ1
Help please!!!! 8. 9. And 10.

Answers
Answer:
9 is 3rd -- 8 is last -- 10 is first --
Step-by-step explanation:
***If this answer Helped you out please press the heart Icon, also It would be nice if you made me brainliest. Enjoy Your Day!***
The residuals for data set A and data set B were calculated and plotted on
separate residual plots. If the residuals for data set A form a pattern and the
residuals for data set B do not form a pattern, what can be concluded?
Answers
Answer:
The answer is A linear model is a good fit for data set b but not data set a
Step-by-step explanation:
It can be concluded that data set A is linear, and data set B is not linear.
What is regression line?A regression line is an estimate of the line that describes the true, but unknown, linear relationship between the two variables. If [Y] is the dependent variable and [X] is the independent variable, the [Y] on [X] regression line equation is represented as follows:[Y] = a + b[X] + ɛ
Given is that residuals for data set A and data set B were calculated and plotted on separate residual plots.
If the residuals for data set [A] form a pattern and the residuals for data set [B] do not form a pattern than it can be concluded that data set A is linear, and data set B is not linear.
To solve more questions on regression line, visit the link below -
brainly.com/question/7656407
#SPJ2
could u please help me with 15, 16 and 17?? thank you:)

Answers
Given:
15)
\(\begin{gathered} f(x)=x^3+4 \\ g(x)=3x^2+7 \\ g(f(x))=g(x^3+4) \\ g(f(x))=3(x^3+4)^2+7 \\ g(f(-1))=3((-1)^3+4)^2+7 \\ g(f(-1))=3(-1+4)^2+7 \\ g(f(-1))=3(3)^2+7 \\ g(f(-1))=34 \end{gathered}\)Answer: option b) 34
16)
\(\begin{gathered} f(x)=x^2+3x+2 \\ g(x)=2x^3+1 \\ (f\circ g)(x)=f(g(x)) \\ (f\circ g)(x)=f(2x^3+1) \\ (f\circ g)(x)=(2x^3+1)^2+3(2x^3+1)+2 \\ (f\circ g)(-1)=(2(-1)^3+1)^2+3(2(-1)^3+1)+2 \\ (f\circ g)(-1)=(-2+1)^2+3(-2+1)+2 \\ (f\circ g)(-1)=1-3+2 \\ (f\circ g)(-1)=0 \end{gathered}\)Answer: option c) 0
17)
\(\begin{gathered} f(x)=x^2+x+1 \\ g(x)=x+3 \\ f(g(x))=f(x+3) \\ f(g(x))=(x+3)^2+(x+3)+1 \\ f(g(x))=x^2+6x+9+x+4 \\ f(g(x))=x^2+7x+13 \end{gathered}\)Answer: option d)