Which could be used to solve this equation? 3 and one-fifth n = 9 Subtract 3 and one-fifth from both sides of the equation. 3 and one-fifth minus 3 and one-fifth n = 9 3 and one-fifth Add 3 and one-fifth to both sides of the equation. 9 3 and one-fifth = 12 and one-fifth.
Answers
To solve the equation 3 and one-fifth n = 9, we can use the method of subtracting or adding the same value to both sides of the equation to isolate the variable.
In this case, we can subtract 3 and one-fifth from both sides or add 3 and one-fifth to both sides of the equation.
To solve the equation 3 and one-fifth n = 9, we can subtract 3 and one-fifth from both sides of the equation, which gives us:
3 and one-fifth n - 3 and one-fifth = 9 - 3 and one-fifth.
Simplifying the left side of the equation, we get:
n = 9 - 3 and one-fifth.
Alternatively, we can add 3 and one-fifth to both sides of the equation, which gives us:
3 and one-fifth n + 3 and one-fifth = 9 + 3 and one-fifth.
Simplifying the left side of the equation, we get:
n = 9 + 3 and one-fifth.
In either case, we have isolated the variable n and obtained the solution by either subtracting or adding the same value to both sides of the equation.
To learn more about variable click here:
brainly.com/question/15078630
#SPJ11
Related Questions
the random variable x is the number of occurrences of an event over an interval of ten minutes. it can be assumed that the probability of an occurrence is the same in any two time periods of an equal length. it is known that the mean number of occurrences in ten minutes is 5.3. the probability that there are less than 3 occurrences is
Answers
According to the Poisson distribution formula, we find out that the probability of less than 3 occurrences for the given random variable is 0.1016.
It is given to us that -
The random variable x is the number of occurrences of an event over an interval of ten minutes
It can be assumed that the probability of an occurrence is the same in any two time periods of an equal length
The mean number of occurrences in ten minutes is 5.3
We have to find out the probability that there are less than 3 occurrences in ten minutes.
Firstly, we can determine that the given random variable x follows Poisson distribution.
This is because Poisson distribution is referred to as a discrete probability distribution which helps us to determine the probability of a certain number of events that occur within a specific time interval.
Here, we see that the random variable x provides the number of occurrences of an event with a specific time interval of ten minutes. So, x follows Poisson distribution.
We know the formula for Poisson distribution is given as -
p(x) = {[e^(-λ)] * (λ^x)}/x! ---- (1)
where,
λ = mean number of occurrences within given time interval
x = number of occurrences
From the given information, we can say that -
λ = 5.3 and x = 3
Substituting these values of λ and x in equation (1), we have
p(x) = {[e^(-λ)] * (λ^x)}/x!
\(= > p(x)=\frac{(e^{-5.3} )*(5.3^{3} )}{3!} \\= > p(x) = 0.1016\)
Thus, by applying Poisson distribution formula, we find out that the probability of less than 3 occurrences for the given random variable is 0.1016.
To learn more about Poisson distribution visit https://brainly.com/question/17280826
#SPJ4
i always get it wrong.

Answers
The situations represented by 25/9 are "Melanie equally shares 25 meters of paper to create 9 banners" and "Joe makes 9 equal servings from a 25 ounce bag of peanuts".
What is an expression?Expression in mathematics is combination of variables with the use of operations and given rules. It can be in the form of equation, numbers etc.
We have the expression 25/9.
That means 25 divided in 9 equal parts.
Now the expression 1,
Melanie equally shares 25 meters of paper to create 9 banners.
We have to divide 25 meters of paper into 9 equal banners.
The above expression can be written as a mathematical term,
and that is 25/9.
Now the expression 2,
Quill gives away 9 baseball cards from a pack of 25.
In mathematical terms; 25 - 9.
Now the expression 3,
George invites 25 kids and 9 adults to his birthday party.
That means, the total 25 + 9 = 34 people
In mathematical terms : 25 + 9
Now the expression 4,
Becca creates 9 rows with 25 buttons each.
That means, each row has 25 buttons.
So the total 9 rows have 9 x 25 buttons.
In mathematical terms : 9 x 25
Now the expression 5,
Joe makes 9 equal servings from a 25 ounce bag of peanuts.
That means 25 ounce bag of peanuts equally divided into 9 parts.
In mathematical terms : 25/9
Therefore, expression 1 : Melanie equally shares 25 meters of paper to create 9 banners and expression 5: Joe makes 9 equal servings from a 25 ounce bag of peanuts, represented by 25/9.
To learn more about the expression;
brainly.com/question/24242989
#SPJ1
evaluate the iterated integral. /4 0 5 0 y cos(x) dy dx
Answers
The value of the iterated integral /4 0 5 0 y cos(x) dy dx is 12.25sin(4). This means that the integral represents the signed volume of the region bounded by the xy-plane
To evaluate the iterated integral /4 0 5 0 y cos(x) dy dx, we first need to integrate with respect to y, treating x as a constant. The antiderivative of y with respect to y is (1/2)y^2, so we have:
∫cos(x)y dy = (1/2)cos(x)y^2
Next, we evaluate this expression at the limits of integration for y, which are 0 and 5. This gives us:
(1/2)cos(x)(5)^2 - (1/2)cos(x)(0)^2
= (1/2)cos(x)(25 - 0)
= (1/2)cos(x)(25)
Now, we need to integrate this expression with respect to x, treating (1/2)cos(x)(25) as a constant. The antiderivative of cos(x) with respect to x is sin(x), so we have:
∫(1/2)cos(x)(25) dx = (1/2)(25)sin(x)
Finally, we evaluate this expression at the limits of integration for x, which are 0 and 4. This gives us:
(1/2)(25)sin(4) - (1/2)(25)sin(0)
= (1/2)(25)sin(4)
= 12.25sin(4)
Therefore, the value of the iterated integral /4 0 5 0 y cos(x) dy dx is 12.25sin(4). This means that the integral represents the signed volume of the region bounded by the xy-plane, the curve y = 0, the curve y = 5, and the surface z = y cos(x) over the rectangular region R = [0,4] x [0,5].
Learn more on iterated integral here:
https://brainly.com/question/29632155
#SPJ11
how do we forecast using data that has seasonality?
How can we control volatility in various time series models?
What is a simple moving average method?
Answers
To forecast using data that has seasonality, one commonly used method is seasonal decomposition of time series (STL). This method separates the time series data into three components: trend, seasonality, and residuals. By isolating the seasonal component, you can forecast future values by extrapolating the pattern observed in previous seasons.
Another approach is the use of seasonal autoregressive integrated moving average (SARIMA) models. SARIMA models are an extension of ARIMA models that incorporate seasonal patterns. These models capture both the trend and seasonality in the data and can be used to make forecasts.
To control volatility in various time series models, a common technique is to use a volatility model, such as the generalized autoregressive conditional heteroskedasticity (GARCH) model. This model estimates the volatility of the time series by incorporating past volatility and squared residuals. By modeling and forecasting the volatility, you can better understand and manage the potential fluctuations in the time series data.
A simple moving average method is a technique used to smooth out fluctuations and identify trends in time series data. It involves calculating the average of a fixed number of data points, often referred to as the window size or period. As new data becomes available, the oldest data point in the window is dropped, and the newest data point is included in the calculation. This process is repeated for each subsequent data point. The resulting moving average values can provide insights into the overall trend of the data, helping to identify patterns or changes over time.
Learn more about seasonal decomposition of time here:-
https://brainly.com/question/28334787
#SPJ11
Some help please? I’m lost

Answers
Answer:
1/2
Step-by-step explanation:
When you find the sine of an angle, it is the opposite side / hypotenuse. This means that the hypotenuse of this triangle is 2 and the side opposite to 60° is √3. This means that the side adjacent to 60° is 1 (Pythagorean Theorem). When you find the cosine of an angle, it is the adjacent side / hypotenuse, which in this case will be 1 / 2. Hope this helps!
Answer:
1/2.
Step-by-step explanation:
Sine = opposite side / hypotenuse.
The side opposite 60 degrees has length√3 and the hypotenuse = 2.
By Pythagoras the side adjacent to the angle 60 = √( 2^2 - 3)
= 1 unit.
So cos 60 = adjacent / hypotenuse = 1/2.
If U is the set of the numbers on a 6-sided die and B is the set of numbers on a 6-sided die that are greater than 3, find B
Answers
The calculated elements in the set B is {4, 5, 6}
Finding the elements in the set BFrom the question, we have the following parameters that can be used in our computation:
U is the set of the numbers on a 6-sided die B is the set of numbers on a 6-sided die that are greater than 3The above means that
U = {1, 2, 3, 4, 5, 6}
The numbers greater than 3 in the above set are
Elements = 4, 5 and 6
This means that the elements in the set B is {4, 5, 6}
Read more about sets at
https://brainly.com/question/24713052
#SPJ1
a food manufacturer recently developed a new preservative to extend the shelf life of its perishable food items. It makes two batches of the perishable items. The preservative is added only to the second batch. After some time, researcher compares the shelf life of the batches. which method is the researcher applying?
Answers
Answer:
The researcher is using the Experiment method
Step-by-step explanation:
Plato
Answer:
Experiment
Step-by-step explanation:
PLATO/Edmentum
I GOT IT RIGHT!!!
I think it’s a but don’t want to get it wrong

Answers
Based on the transformation of the given parent function f(x), the function g(x) is: C. g(x) = ∛(x) + 2
What is a translation?In Mathematics, the translation of a geometric figure to the right is a type of transformation which simply means adding a digit to the value on the x-coordinate (x-axis) of the pre-image while translating a geometric figure up is a type of transformation that simply means adding a digit to the value on the y-coordinate (y-axis) of the pre-image.
Translating the parent function f(x) = ∛(x) two (2) units up, would produce an image of function f(x):
g(x) = f(x) + 2
g(x) = ∛(x) + 2
In this context, we can reasonably infer and logically deduce that the parent function f(x) was shifted 2 units up to produce function g(x).
Read more on translation here: brainly.com/question/20720324
#SPJ1
A company has 10 men qualified to run a machine that requires 3 operators at a time. find how many groups of 3 operators are possible?
Answers
The number of possible groups or combination of three men can be formed from 10 is 120.
According to the given question.
Total number of men in a company, n = 10.
Number of men to be selected, r = 3
As we know that, What is a combination in math?
Image result for what is combination
A combination is a mathematical technique that determines the number of possible arrangements in a collection of items where the order of the selection does not matter.
Therefore, the number of possible groups or combination of three men can be formed from 10
= \(^{10} C_{3}\)
= 10!/ 3!7!
= 10(9)(8)/3(2)
= 5 × 3 × 8
= 120
The number of possible groups or combination of three men can be formed from 10 is 120.
Find out more information about number of possible groups or combination here:
https://brainly.com/question/3854382
#SPJ4
2. [3pts] In the context of a simple linear regression model y = β0 + estimated by least squares with n observations, show that the LS estimator of βˆ0 is equal to the sample mean of the dependent variable.
3. [4pts] In the context of a simple linear regression model y = β0 + β1x + estimated by least squares with n observations, show that the LS estimator of βˆ1 is βˆ 1 = sample covariance between x and y sample variance ofx Most of the following questions require a proof. Please make sure you mention the assumptions used in your proofs.
Answers
The LS estimator of βˆ0 in a simple linear regression model is equal to the sample mean of the dependent variable, while the LS estimator of βˆ1 is equal to the sample covariance between x and y divided by the sample variance of x.
1. LS estimator of βˆ0:
In a simple linear regression model, the equation is y = β0 + ε, where y represents the dependent variable, β0 is the intercept, and ε is the error term. The LS estimator of βˆ0 is obtained by minimizing the sum of squared residuals (SSR). The SSR is defined as the sum of the squared differences between the observed values of y and the predicted values of y based on the model.
When we minimize the SSR, we differentiate it with respect to β0 and set the derivative equal to zero. This leads to the condition that the LS estimator of βˆ0 is equal to the value of β0 that minimizes the SSR. The value of β0 that minimizes the SSR is the one that makes the sum of the residuals equal to zero.
Since the residuals represent the differences between the observed values of y and the predicted values of y, the sum of the residuals can be interpreted as the sum of the deviations of the observed values from the predicted values. When the sum of the residuals is zero, it implies that the sum of the deviations is also zero.
Therefore, the LS estimator of βˆ0 is equal to the sample mean of the dependent variable, as the sample mean represents the average value of the dependent variable and minimizes the deviations from the predicted values.
2. LS estimator of βˆ1:
In a simple linear regression model, the equation is y = β0 + β1x + ε, where y represents the dependent variable, x represents the independent variable, β0 is the intercept, β1 is the slope, and ε is the error term. The LS estimator of βˆ1 is obtained by minimizing the SSR, similar to the LS estimator of βˆ0.
To derive the LS estimator of βˆ1, we differentiate the SSR with respect to β1 and set the derivative equal to zero. This leads to the condition that the LS estimator of βˆ1 is equal to the value of β1 that minimizes the SSR. The value of β1 that minimizes the SSR is the one that makes the sum of the products of the residuals and the corresponding values of x equal to zero.
The product of the residuals and the values of x represents the covariance between x and y, as it measures the joint variability between the two variables. The sum of these products being zero implies that the covariance between x and y is zero.
The sample covariance between x and y divided by the sample variance of x is an unbiased estimator of the population slope β1. Hence, the LS estimator of βˆ1 is given by the sample covariance between x and y divided by the sample variance of x.
Learn more about estimator
brainly.com/question/30876115
#SPJ11
Determine the inverse.
g(x)=7(x-8)² +3
for 8 ≤ x < ♾️
Answers
The inverse of the given function could be; y = √x / 7 + 8
What is inverse of a function?Suppose that the given function is \(f:X\rightarrow Y\)
Then, if function 'f' is one-to-one and onto function (a needed condition for inverses to exist), then, the inverse of the considered function is
\(f^{-1}: Y \rightarrow X\)
This simply means, inverse of 'f' is undo operator, that takes back the effect of 'f'
We have been given the function as g(x)=7(x-8)² +3 for 8 ≤ x < ∝
Function that can reverse into another function is known as an inverse function or anti function to find y when x is swapped with another variable, we must first determine the inverse function.
On the other hand, the inverse function and the original function have interchangeable domains and ranges.
y = 7(x-8)²
(x-8)² = y/7
x - 8 = y / 7
x = √y / 7 + 8
Or
y = √x / 7 + 8
Learn more about inverse function here:
https://brainly.com/question/19425567
#SPJ1
Five eighths of the members of a soccer team ride the bus. If there are 24 members on the soccer team, how many members ride the bus? Explain how to use equivalent fractions to solve.
How many members ride the bus?
Answers
Answer:
15
Step-by-step explanation:
"of" means times (×)
\(\frac{5}{8} \times\frac{24}{1}\)
Multiply across:
\(5\times 24=120\)
\(8\times 1= 8\)
\(\frac{120}{8}\)
Divide:
\(120\div8=15\)
Therefore, 15 people on the soccer ride the bus.
The equation that represents the canned goods order is 24x + 64y = 384.
x = number of minutes for fruit cans
y = number of minutes for vegetable cans
Explain how to calculate the x- and y-intercepts.
Answers
Answer:
intercept
16,0
0,6
Step-by-step explanation:
Answer:
Substitute 0 for the x-value in the equation and solve for y. The result is y = 6, so the y-intercept is at (0, 6). Next, substitute 0 for the y-value in the equation and solve for x. The result is x = 16, so the x-intercept is at (16, 0).
Step-by-step explanation:
I did the assignment on edge its 8 grade math
A bolt manufacturer is very concerned about the consistency with which his machines produce bolts. The bolts should be 0.2 centimeters in diameter. The variance of the bolts should be 0.025. A random sample of 15 bolts has an average diameter of 0.21 cm with a standard deviation of 0.1587. Can the manufacturer conclude that the bolts vary by more than the required variance at α=0.01 level? Step 1 of 5: State the hypotheses in terms of the standard deviation. Round the standard deviation to four decimal places when necessary. A bolt manufacturer is very concerned about the consistency with which his machines produce bolts. The bolts should be 0.2 centimeters in diameter. The variance of the bolts should be 0.025. A random sample of 15 bolts has an average diameter of 0.21 cm with a standard deviation of 0.1587. Can the manufacturer conclude that the bolts vary by more than the required variance at α=0.01 level? Step 2 of 5: Determine the critical value(s) of the test statistic. If the test is twotailed, separate the values with a comma. Round your answer to three decimal places. A bolt manufacturer is very concerned about the consistency with which his machines produce boits. The bolts should be 0.2 centimeters in diameter. The variance of the boits should be 0.025. A random sample of 15 bolts has an average diameter of 0.21 cm with a standard deviation of 0.1587. Can the manufacturer conclude that the bolts vary by more than the required variance at α=0.01 level?
Answers
To determine if the bolts vary by more than the required variance, we can conduct a hypothesis test. The null hypothesis (H₀) states that the variance of the bolts is equal to or less than the required variance (σ² ≤ 0.025), while the alternative hypothesis (H₁) states that the variance is greater than the required variance (σ² > 0.025).
Next, we need to determine the critical value(s) of the test statistic. Since we are testing for variance, we will use the chi-square distribution. For a one-tailed test with α = 0.01 and 14 degrees of freedom (n-1), the critical value is 27.488.
Now, we can compare the test statistic to the critical value. The test statistic is calculated as (n-1) * s² / σ², where n is the sample size (15), s² is the sample variance (0.1587²), and σ² is the required variance (0.025).
If the test statistic is greater than the critical value, we reject the null hypothesis and conclude that the bolts vary by more than the required variance. Otherwise, we fail to reject the null hypothesis.
To learn more about Hypothesis test - brainly.com/question/29996729
#SPJ11
To determine if the bolts vary by more than the required variance, we can conduct a hypothesis test. The null hypothesis (H₀) states that the variance of the bolts is equal to or less than the required variance (σ² ≤ 0.025), while the alternative hypothesis (H₁) states that the variance is greater than the required variance (σ² > 0.025).
Next, we need to determine the critical value(s) of the test statistic. Since we are testing for variance, we will use the chi-square distribution. For a one-tailed test with α = 0.01 and 14 degrees of freedom (n-1), the critical value is 27.488.
Now, we can compare the test statistic to the critical value. The test statistic is calculated as (n-1) * s² / σ², where n is the sample size (15), s² is the sample variance (0.1587²), and σ² is the required variance (0.025).
If the test statistic is greater than the critical value, we reject the null hypothesis and conclude that the bolts vary by more than the required variance. Otherwise, we fail to reject the null hypothesis.
To learn more about Hypothesis test - brainly.com/question/29996729
#SPJ11
John and Mary had Php 384 altogether. After John gave Php 36 to Mary, Mary had 3 times as much money as John. How much money did Mary had at first?
Answers
John initially had Php 132 and Mary initially had Php 252 (384 - 132).
Let's start by setting up the equations to solve for this problem.
Let x be the amount of money John had initially.
Then, Mary had (384 - x) initially.
After John gave Php 36 to Mary, John had (x - 36) left and Mary had (384 - x + 36) = (420 - x).
We are told that Mary had three times as much money as John after this transaction, so we can write:
3(x - 36) = 420 - x
Simplifying this equation gives:
3x - 108 = 420 - x
4x = 528
x = 132
Therefore, John initially had Php 132 and Mary initially had Php 252 (384 - 132).
Answer: Mary had Php 252 at first.
Learn more about initially from
https://brainly.com/question/31041139
#SPJ11
Compute the value: 5+ 6+ 7+ 8+9+...+200 52. (4) Consider the sequence (bi) defined as follows: b₁-4, and b=3b4-1 for k>1. Find the term bio.
Answers
The calculated value of the tenth term, b₁₀ of the sequence is 78732
How to calculate the tenth term, b₁₀ of the sequenceFrom the question, we have the following parameters that can be used in our computation:
b₁ = -4
bₙ = 3bₙ₋₁
The above means that
We multiply the current term by 4 to get the next term
So, we have
b₂ = 3 * 4 = 12
b₃ = 3 * 12 = 36
b₄ = 3 * 36 = 108
b₅ = 3 * 108 = 324
b₆ = 3 * 324 = 972
b₇ = 3 * 972 = 2916
b₈ = 3 * 2916 = 8748
b₉ = 3 * 8748 = 26244
b₁₀ = 3 * 26244 = 78732
Hence, the tenth term, b₁₀ of the sequence is 78732
Read more about sequence at
https://brainly.com/question/30499691
#SPJ1
which factors will influence the margin of error when the population standard deviation is unknown? check all that apply.
Answers
We need to know about margin of error to solve the problem. The factors that influence margin of error are confidence level and sample size.
Margin of error is a statistic expressing the amount of random sampling error in the results of a survey. The larger the margin of error, the less confidence one should have that a poll result will reflect the result of a census of the entire population. Margin of error is influenced by three factors, confidence level, sample size and population standard deviation. In absence of population standard deviation, the margin of error is influenced by confidence level and sample size.
Therefore the margin of error is influenced by confidence level and sample size.
Learn more about margin of error here:
https://brainly.com/question/10218601
#SPJ4
the slope of line (-1,1) (2,-5)?
Answers
Answer:
slope = 4/3
Step-by-step explanation:
(-1 , 1) = (x1 , y1)
(2 , 5) = (x2 , y2)
slope (m) = y2 - y1/x2 - x1
=5 - 1/2 - (-1)
=4 /2+1
=4/3
what are the next 2 sequences to 5, 1, 6, 7, 13, 21, 34,
Answers
Answer:
55,89
Step-by-step explanation:
Here we see a sequence where the pattern is achieved by adding each new term to the term before to get the next term
5+1=6
1+6=7
6+7=13 etc
So for the next two terms: 21+34=55
55+34=89
Answer:
5, 1, 6, 7, 13, 21, 34, 51, 77
Solution:
Next possible number in the given sequence I. -4,1,8 is 17
Next possible number in the given sequence II. 5,6,13 is 26
☆ The next 2 number for given series -4,5,1,6,8,13 are 17,26
☆The next 2 number for given series 5,1,6,7,13,21,34 are 51,77
what is equalivliant to 4a + (–6b) – 3a + 2b
Answers
Answer: a-4b
Step-by-step explanation:
Combine like terms
4a-6b-3a+2b
1a-6b+2b
a-6b+2b
a-4b
Calculus Vector Application Handout An airplane is traveling at a speed of 724 km/hr at a direction of 30°. The wind is blowing from the west at 32 km/hr. Find the resultant speed and true course of the plane.
Answers
The resultant speed of the plane is approximately 742.82 km/hr, and the true course of the plane is approximately 179.55°.
To find the resultant speed and true course of the plane, we need to consider the velocity vectors of the plane and the wind.
Let's represent the velocity of the plane as vector P and the velocity of the wind as vector W.
Given:
Speed of the plane = 724 km/hr
Direction of the plane = 30°
Speed of the wind = 32 km/hr
First, we need to convert the given speeds and direction into their corresponding vector form.
The velocity vector of the plane P can be represented as:
P = 724(cosθ, sinθ)
where θ is the direction of the plane in radians. To convert the given angle from degrees to radians, we use the formula: radians = degrees * π / 180.
So, θ = 30° * π / 180 = π / 6 radians.
Substituting the values, we have:
P = 724(cos(π/6), sin(π/6))
P = 724(√3/2, 1/2)
The velocity vector of the wind W is given as:
W = 32(-1, 0) (since the wind is blowing from the west)
Now, to find the resultant velocity vector R, we add the vectors P and W:
R = P + W
R = 724(√3/2, 1/2) + 32(-1, 0)
R = (362√3 - 32, 362/2)
The magnitude of the resultant velocity vector R represents the resultant speed of the plane, and the direction of the vector represents the true course of the plane.
To find the magnitude (resultant speed) of R, we use the formula:
Magnitude of R = √(R_x^2 + R_y^2)
Substituting the values, we have:
Magnitude of R = √((362√3 - 32)^2 + (362/2)^2)
Magnitude of R ≈ 742.82 km/hr
To find the direction (true course) of R, we use the formula:
Direction of R = tan^(-1)(R_y / R_x)
Substituting the values, we have:
Direction of R = tan^(-1)((362/2) / (362√3 - 32))
Direction of R ≈ 179.55°
Therefore, the resultant speed of the plane is approximately 742.82 km/hr, and the true course of the plane is approximately 179.55°.
To learn more about resultant speed
https://brainly.com/question/148987
#SPJ11
Benny's arcade has five video game machines. The average time between failures (i.e. the average time between jobs) is 34 hours. The maintenance engineer can repair a machine in about 13 hours. Assume the failure time and repair times are both exponentially distributed. What is the average time in HOURS from when a machine breaks until it is fixed?
Answers
The average time in hours from when a machine breaks until it is fixed is approximately 11.3 hours.
Given,
Benny's arcade has five video game machines
The average time between failures = 34 hours
The maintenance engineer can repair a machine in about = 13 hours
The failure time and repair times are both exponentially distributed
The formula for the mean of an exponential distribution is mean = 1/λ where λ is the rate parameter of the distribution.
In this problem, the rate parameter of both the failure time and repair time is the reciprocal of their respective averages. Therefore,
λ_f = 1/34λ_r = 1/13
Now, let's find the average time from when a machine breaks until it is fixed using the fact that the sum of two independent exponential distributions with rate parameters λ1 and λ2 is itself an exponential distribution with rate parameter λ1+λ2.
So, the rate parameter for the time from when a machine breaks until it is fixed is λ_f+λ_r = 1/34+1/13
= 0.0885 hours⁻¹ (approximately)
Hence, the average time from when a machine breaks until it is fixed is mean = 1/λ= 1/0.0885 ≈ 11.3 hours (approximately).
Therefore, the average time in hours from when a machine breaks until it is fixed is approximately 11.3 hours.
To know more about average visit:
https://brainly.com/question/897199
#SPJ11
How many terms are in the expression 3x+y−23−5
Answers
Answer:
There are 4 terms
Step-by-step explanation:
“Terms are single numbers, variables, or the product of a number and variables. Examples of terms: 9 a 9a 9a. y y y.”
Hi can anyone please help me with this problem, I’d really appreciate it
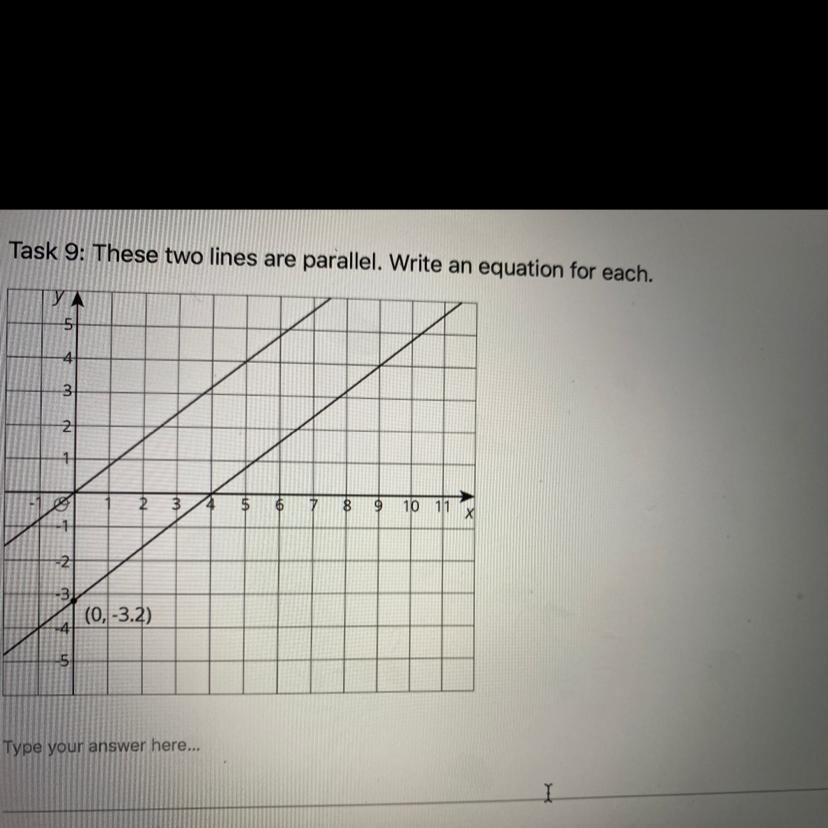
Answers
We can start this equation off by noticing the fact that this is a linear equation, which we can write in the form of: \(mx+b\). We can plug in our x values and y values given. I am going to start on the second line:
We plug in the given point: \((0,-3.2)\).
\(-3.2=m(0)+b\\-3.2=b\)
We can also plug in the point \((4,0)\) to get:
\(0 = m(4) -3.2\)
We get \(m = 0.8\)
Therefore, our equation for the SECOND line is:
\(y = 0.8m - 3.2\)
Hope that this helps you do the first line.
Let f(x)= 2 square root of x
If g(x) is the graph of f(x) shifted down 2 units and left 1 units, write a formula for g(x)
Answers
Answer:
g(x)=2\(\sqrt{x+1}\)-2
Step-by-step explanation:
if f(x)=2\(\sqrt{x}\) then g(x) shifted down 2 and left 1 would be g(x)=2\(\sqrt{x+1}\)-2
The following joint probability density function for the random variables Y1 and Y2, which represent the proportions of two components in a somaple from a mixture of insecticide.
f(y1,y2) = { 2, 0 <= y1 <= 1, 0 <= y2 <= 1, 0 <= y1+y2 <=1
{ 0, elsewhere
For the chemicals under considerationm an important quantity is the total proportion Y1 +Y2 found in any sample. Find E(Y1+Y2) and V(Y1+Y2).
Answers
The chemicals under considerationm an important quantity is the total proportion Y1 +Y2 found in any sample.V(Y1 + Y2) = Var(Y1) + Var(Y2) + 2Cov(Y1, Y2) = 1/18 + 1/18 + 2(1/144) = 5/72.
To find E(Y1 + Y2), we first find the marginal distribution of Y1 and Y2 by integrating the joint density function over the other variable as follows:
f1(y1) = ∫f(y1,y2)dy2 = ∫2dy2 from 0 to 1-y1 = 2(1-y1) for 0 ≤ y1 ≤ 1
f2(y2) = ∫f(y1,y2)dy1 = ∫2dy1 from 0 to 1-y2 = 2(1-y2) for 0 ≤ y2 ≤ 1
Now we can find E(Y1 + Y2) as follows:
E(Y1 + Y2) = ∫∫(y1 + y2)f(y1,y2)dy1dy2
= ∫∫(y1 + y2)2dy1dy2 over the region 0 ≤ y1 ≤ 1-y2, 0 ≤ y2 ≤ 1
E(Y1 + Y2) = ∫0^1∫0^(1-y1) (y1 + y2)2dy2dy1
= ∫0^1[y1(y1/2 + 2/3) + 1/3]dy1
= 7/12
To find V(Y1 + Y2), we can use the fact that V(Y1 + Y2) = V(Y1) + V(Y2) + 2Cov(Y1, Y2), where Cov(Y1, Y2) is the covariance of Y1 and Y2. First, we need to find the variances of Y1 and Y2:
Var(Y1) = E(Y1^2) - [E(Y1)]^2 = ∫∫y1^22dy1dy2 - [∫∫y1f(y1,y2)dy1dy2]^2
= ∫0^1∫0^(1-y1) y1^22dy2dy1 - [∫0^1(2y1-2y1^2)dy1]^2
= 1/18
Var(Y2) = E(Y2^2) - [E(Y2)]^2 = ∫∫y2^22dy1dy2 - [∫∫y2f(y1,y2)dy1dy2]^2
= ∫0^1∫0^(1-y2) y2^22dy1dy2 - [∫0^1(2y2-2y2^2)dy2]^2
= 1/18
Now we need to find the covariance of Y1 and Y2:
Cov(Y1, Y2) = E(Y1Y2) - E(Y1)E(Y2) = ∫∫y1y2f(y1,y2)dy1dy2 - (∫∫y1f(y1,y2)dy1dy2)(∫∫y2f(y1,y2)dy1dy2)
= ∫0^1∫0^(1-y1) 2y1y2dy2dy1 - (7/12)(7/12)
= 1/144
Therefore, V(Y1 + Y2) = Var(Y1) + Var(Y2) + 2Cov(Y1, Y2) = 1/18 + 1/18 + 2(1/144) = 5/72.
Learn more about proportion here:
https://brainly.com/question/31548894
#SPJ11
Write each of the following in scientific notation.
a. 1,000,000 _______
b. .00005 _______
c. 40 thousand _______
d. 7 trillion _______
e. 8 thousandths _______
f. one tenth _______
Answers
The scientific notation of the given numbers are:
a. 1,000,000 - 1 x \(10^{6}\)
b. .00005 - 5 x \(10^{-5}\)
c. 40 thousand- 4 x \(10^{4}\)
d. 7 trillion - 7 x \(10^{12}\)
e. 8 thousandths - 8 x \(10^{-3}\)
f. one-tenth - 1 x \(10^{-1}\)
We are given the following:
- 1,000,000
- 0.00005
- 40 thousand
- 7 trillion
- 8 thousandths
- one tenth
We will write the following above in scientific notation.
What is scientific notation?It is a form of presenting very large numbers in a simpler form.
We have to make the number in a form of multiplication of single-digit and 10 raised to a power of a positive integer.
- 1000000
In scientific notation = 1 x \(10^{6}\)
- 0.00005
In scientific notation = 5 x \(10^{-5}\)
- 40 thousand = 40,000
In scientific notation = 4 x \(10^{4}\)
- 7 trillion
= 7,000,000,000,000
In scientific notation = 7 x \(10^{12}\)
- 8 thousandths
It is in decimal place so we have 0.008.
In scientific notation = 8 x \(10^{-3}\)
-one-tenth
It means one out of 10 parts.
= 1 / 10
= 0.1
In scientific notation = 1 x \(10^{-1}\)
The scientific notation of the given numbers are:
a. 1,000,000 - 1 x \(10^{6}\)
b. .00005 - 5 x \(10^{-5}\)
c. 40 thousand- 4 x \(10^{4}\)
d. 7 trillion - 7 x \(10^{12}\)
e. 8 thousandths - 8 x \(10^{-3}\)
f. one-tenth - 1 x \(10^{-1}\)
Learn more about scientific notation here:
https://brainly.com/question/18073768
#SPJ1
Please look at photo for question, thank you so much <3

Answers
When two triangles are similar the corresponding sides are always in the same ratio.
If triangle MNP is similar to traingle XYZ, the lengths of MNP needs to be in the same ratio with the corresponding sides of triangle XYZ
\(\frac{MN}{XY}=\frac{NP}{YZ}=\frac{MP}{XZ}\)\(\frac{MN}{6}=\frac{MP}{7}=\frac{NP}{9}\)Prove each of the given options lengths to find the one that makes the equation above be true:
Pair corresponding sides ordering its lengths from least XY to greatest YZ
First option:
\(\begin{gathered} \frac{12}{6}=\frac{14}{7}=\frac{20}{9} \\ 2=2=2.22 \end{gathered}\)As the ratios are not the same that cannot be the lengths of MNP
Second option:
\(\begin{gathered} \frac{30}{6}=\frac{35}{7}=\frac{45}{9} \\ 5=5=5 \\ \end{gathered}\)As the ratios are the same that can be the lengths of MNP
Third option:
\(\begin{gathered} \frac{13}{6}=\frac{14}{7}=\frac{16}{9} \\ 2.16=2=1.77 \end{gathered}\)As the ratios are not the same that cannot be the lengths of MNP
Fourth option:
\(\begin{gathered} \frac{3}{6}=\frac{4}{7}=\frac{5}{9} \\ 0.5=0.57=0.55 \end{gathered}\)As the ratios are not the same that cannot be the lengths of MNP
Then, the lengths of MNP could be: 30cm,35cm,45cmWhich ordered pair is a solution of the equation?
y= 4x + 9
Answers
Answer: In order to find this, we simply need to select an x and it will help us find y. Our x for this case will be 1. Now we put that into the equation and solve for the y value.
y = 4x + 9
y = 4(1) + 9
y = 4 + 9
y = 13
This gives us the ordered pair of (1, 13)
Step-by-step explanation:
Answer:
(0,9),(1,13),(2,17)
Step-by-step explanation:
i hope this is correct!! c:
Reconstruction failed to establish racial equality and black freedom. Explain how the rapid industrialization of the United States under the system of "free labor" in the years after the Civil War led to a social crisis by the end of the nineteenth century in which the traditional American values of democracy, equality, and opportunity seemed to be disappearing, and in which class conflict threatened to tear society apart. How did the capitalists and working classes attempt to enhance their own power and interests in their struggle with each other? Why was the working class unable to achieve much, despite valiant efforts? How did the middle-class respond to the struggle between labor and capital as well as the changes that American society underwent during the late nineteenth century?
Answers
The working class was unable to achieve much, despite valiant efforts, due to their lack of solidarity and the capitalist's willingness to use violence against them.The middle class responded to the struggle between labor and capital, as well as the changes that American society underwent during the late nineteenth century, by supporting a variety of social reform movements.
After the Civil War, Reconstruction failed to establish racial equality and black freedom in the United States. The rapid industrialization of the United States under the system of "free labor" in the years following the Civil War contributed to a social crisis by the end of the nineteenth century. This crisis seemed to be causing the disappearance of traditional American values of democracy, equality, and opportunity, and class conflict was threatening to tear society apart.In their struggle against each other, capitalists and working classes attempted to enhance their own power and interests. Capitalists attempted to enhance their power by instituting new labor policies, cutting wages, and lowering working conditions.
The working class was unable to achieve much, despite valiant efforts, due to their lack of solidarity and the capitalist's willingness to use violence against them.The middle class responded to the struggle between labor and capital, as well as the changes that American society underwent during the late nineteenth century, by supporting a variety of social reform movements. They sought to provide relief for the urban poor and to reform politics by promoting women's suffrage and demanding the elimination of political corruption.In conclusion, Reconstruction failed to establish racial equality and black freedom in the United States. The rapid industrialization of the United States under the system of "free labor" in the years following the Civil War contributed to a social crisis by the end of the nineteenth century. Capitalists and working classes attempted to enhance their power and interests in their struggle with each other. The working class was unable to achieve much, despite valiant efforts. The middle class responded to the struggle between labor and capital, as well as the changes that American society underwent during the late nineteenth century, by supporting a variety of social reform movements.
Learn more about American society here:
https://brainly.com/question/12006768
#SPJ11