what is 108 x 0.37 in money
Answers
Related Questions
Let log_b 4= G and log_b9= S. Write the expression in terms of G and S

Answers
S-G is the expression in terms of G and S
What is the expression in terms of G and S?Given: log_b 4 = G, log_b9 = S and log_b 9/4
To write log_b 9/4 in terms of G and S, we need to understand logarithm division rule, which states that:
When you divide two values with the same base is to subtract the exponents. Simply, the rule for division is to subtract the logarithms i.e.
logₓ (a/c) = logₓa - logₓc
Thus, log_b (9/4) = log_b 9 - log_b 4 = S - G
(Note: log_b 4 = G and log_b9= S)
Therefore, the expression log_b (9/4) in terms of G and S is S-G
Learn more about logarithm rules on:
https://brainly.com/question/28596566
#SPJ1
If the nth partial sum of a series n = 1 as it approaches infinity an is sn = 6 - n5^-n, find an and n = 1 as it approaches infinity an.

Answers
By using the partial sum of series is
\(a_{n} = 5^{(-n-1) } - (n+1)5^{(-(n+1))}\)
and summation n=1 to infinity of \(a_{n}\) is =6 .
How to find the nth term?To obtain the formula for the nth term of the series and its total, we must first discover the formula for the series' general term.
The formula for the series' nth partial sum is as follows:
\(sn = 6 - n*5^{-n}\)
The nth term of the series can be obtained by subtracting the (n+1)th and nth partial sums, i.e.,
a = sn+1 - sn
When we substitute the formula for sn, we get:
\(a_{n} = [6 - (n+1)5^{(-(n+1))} ] - [6 - n5^{(-n)} ]\\a_{n} = 5^{(-n-1) } - (n+1)5^{(-(n+1))}\)
As a result, the formula for the series' nth term is:
\(a_{n} = 5^{(-n-1) } - (n+1)5^{(-(n+1))}\)
To calculate the series total, take the limit of the nth partial sum as n approaches infinity:
lim(n->inf) lim(n->inf) sn [6 - n*5^(-n)]
lim(n->inf) [n*5(-n)] = 6
= 6 - 0
= 6
As a result, the series' sum is 6.
As a result, the formula for the nth term in the series is:
\(a_{n} = 5^{(-n-1) } - (n+1)5^{(-(n+1))}\)
And the series' total is:
a = sum(n=1 to inf) = 6
Learn more about the partial series here:
https://brainly.com/question/29166558
#SPJ1
What is the range of the absolute value function below?
Answers
The range of the absolute value function y = |x| is all non-negative numbers, which can be expressed as [0, ∞).
The absolute value function is a type of mathematical function that measures the distance between a given number and zero. It is denoted by two vertical bars surrounding the number or expression.
The range of the absolute value function is the set of all non-negative numbers. This is because the absolute value of any number is always a positive number or zero.
For example, the absolute value of 3 is 3, and the absolute value of -3 is also 3.This means that the output of the function can never be negative and can range from zero to infinity.
To learn more about : range
https://brainly.com/question/30389189
#SPJ8
The range of the absolute value function below is C. f(x) < 1.
How to explain the informationThe domain and range of a function are the components of a function. The domain is the set of all the input values of a function and range is the possible output given by the function. Domain→ Function →Range.
The range of a function is the set of all its outputs. Example: Let us consider the function f: A→ B, where f(x) = 2x and each of A and B = {set of natural numbers}.
The function y=|ax+b| is defined for all real numbers. So, the domain of the absolute value function is the set of all real numbers. The absolute value of a number always results in a non-negative value.
Learn more about range on
https://brainly.com/question/4106049
#SPJ1
What is the range of the absolute value function below?
f(x) > -4
f(x) > -1
f(x) < 1
f(x) < 4
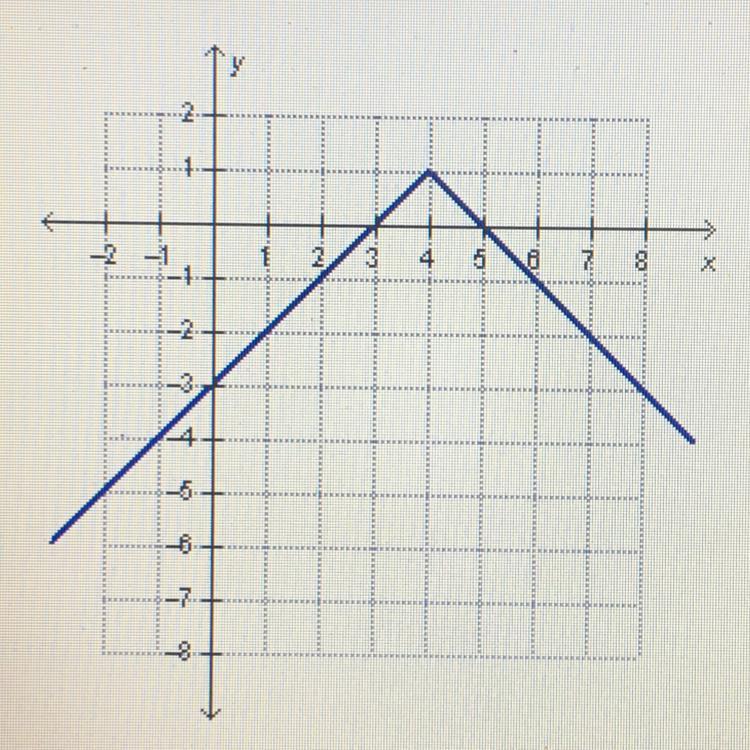
Find the slope of the line containing the points (7,5) and (9,5).
Answers
Answer:
\(\frac{2}{4}\)
Step-by-step explanation:
Remember that in order to find the slope of two points you need to substitute the variables and subtract making sure you keep the 2's and the 1's together.
\(m=\frac{y2-y1}{x2-x1}\)
Plugin your numbers:
\(=\frac{7-5}{9-5}\)
\(7-5=2\)
\(9-5=4\)
\(=\frac{2}{4}\)
Hope this helps.
The area of a circle is 9π m². What is the circumference, in meters? Express answer in terms of π
Answers
Answer:
20.25 pi
Step-by-step explanation:
The circumference of a circle is given by
C = 2 * pi *r
9 pi = 2 * pi *r
Divide each side by 2pi
9pi / 2 pi = 2 pi r / 2 pi
4.5 = r
Now we can find area
A = pi r^2
= pi ( 4.5)^2
20.25 pi
Use the following table to find the probability that a randomly chosen member of the Student Government Board is a graduate student or lives in on-campus housing. Express your answer as a fraction in lowest terms or a decimal rounded to the nearest millionth.
Students on the Student Government Board
On-Campus Housing Off-Campus Housing
Freshman 2 2
Sophomore 2 4
Junior 0 3
Senior 4 2
Graduate Student 2 0
Answers
The probability that a randomly chosen member of the Student Government Board is a graduate student or lives in on-campus housing is 8/25 or 0.32 (rounded to the nearest millionth).
1. Calculate the total number of students on the Student Government Board by summing up the numbers in the table:
Total Students = 2 + 2 + 2 + 4 + 0 + 3 + 4 + 2 = 19
2. Calculate the total number of graduate students on the Student Government Board:
Total Graduate Students = 2 + 0 = 2
3. Calculate the total number of students living in on-campus housing:
Total On-Campus Housing = 2 + 2 + 0 + 4 + 2 = 10
4. Calculate the probability of selecting a graduate student from the Student Government Board by dividing the total number of graduate students by the total number of students:
Probability of Graduate Student = Total Graduate Students / Total Students = 2 / 19
5. Calculate the probability of selecting a student living in on-campus housing by dividing the total number of students in on-campus housing by the total number of students:
Probability of On-Campus Housing = Total On-Campus Housing / Total Students = 10 / 19
6. Calculate the probability that a randomly chosen member of the Student Government Board is a graduate student or lives in on-campus housing by summing up the probabilities from steps 4 and 5:
Probability = Probability of Graduate Student + Probability of On-Campus Housing = 2 / 19 + 10 / 19
7. Simplify the fraction if necessary. In this case, the fraction cannot be simplified further, so the final probability is 2 / 19 + 10 / 19 = 12 / 19.
8. Convert the fraction to a decimal by dividing the numerator by the denominator: 12 / 19 ≈ 0.631578947, which rounds to 0.632 (rounded to the nearest thousandth).
9. Finally, express the probability as a fraction in lowest terms: 12 / 19 is already in lowest terms.
Therefore, the probability that a randomly chosen member of the Student Government Board is a graduate student or lives in on-campus housing is 12/19 or approximately 0.632 (rounded to the nearest thousandth).
For more such questions on probability, click on:
https://brainly.com/question/31048927
#SPJ8
Which is the graph of g(x) = 2^x-1 + 3?
Answers
Answer:
gave no examples.. this is what I got
Step-by-step explanation:

Complete the function table for each equation

Answers
Answer:
y=-32, 0, -16, -4, 16
Step-by-step explanation:
y=4(-6)-8= -32
y=4(2)-8 = 0
y=4(-2)-8 = -16
y=4(1) -8 = -4
y=4(6) -8= 16
For the given equation of the line, the complete table is attached below.
Use the concept of the equation of line defined as:
A line has length but no width, making it a one-dimensional figure. A line is made up of a collection of points that can be stretched indefinitely in opposing directions. Two points in a two-dimensional plane determine it.
The given equation of a line is,
y = 4x - x
To complete the table:
Put x = -6 in this equation,
y = (-4)(-6) - 8
y = 16
Put x = 2 in the equation,
y = (-4)(2) - 8
y = -16
Put x = -2 in the equation,
y = (-4)(-2) - 8
y = 0
Put x = 1 in the equation,
y = (-4)(1) - 8
y = -12
Put x = 6 in the equation,
y = (-4)(6) - 8
y = -32
The complete table is attached below.
To learn more about the equation of line visit:
brainly.com/question/18831322
#SPJ6

A company's human resources department was interested in the average number of years that a person works before retiring. The sample of size 11 follows: Based on the values of the arithmetic mean, median, and mode, what is the most likely shape of the distribution
Answers
Answer:
hello your question is incomplete below is the complete question
A company's human resources department was interested in the average number of years that a person works before retiring. The sample of size 11 follows: 12 16 18 19,21 21, 21,22 24 24 26What is the arithmetic mean, median and mode? what is the most likely shape of the distribution
answer :
i) mean = 20.36
ii) median = 21
iii) mode = 21
iv) The most likely shape is a Bell - shaped curve
Step-by-step explanation:
sample size = 11
values ( observations ) = 12, 16, 18, 19,21,21,21,22,24,24,26
i) mean
= ∑ values / sample size = 224 / 11 = 20.36
ii) median
median is the value of the data located at the center of the string of data
to determine the middle position = ( n+1 / 2 )
where n = sample size
hence position of median value = 11 + 1 / 2 = 12 / 2 = 6
the value at the 6th position = 21 ( median )
iii) mode
mode = most repeated value in the series of data
hence mode value = 21
Evaluate the double integral. 4y2 dA, D is the triangular region with vertices (0, 1), (1, 2), (4, 1)
Answers
Answer:
44/3
Step-by-step explanation:
Let A be the line joining the vertices (0, 1) and (1,2) while B be the equation of the line joining (1, 2) and (4, 1).
The equation of a line joining points \((x_1,y_1) \ and\ (x_2,y_2)\ is:\\\\\)
\(y-y_1=\frac{y_2-y_1}{x_2-x_1} (x-x_1)\)
The equation of line A joining (0, 1) and (1,2) is:
\(y-1=\frac{2-1}{1-0} (x-0)\\\\y-1=x\\\\x=y-1\)
The equation of line D joining (1, 2) and (4,1) is:
\(y-2=\frac{1-2}{4-1} (x-1)\\\\y-2=-\frac{1}{3} (x-1)\\\\3y-6=-x+1\\\\x=-3y+7\)
Therefore the change in y is: 1 ≤ y ≤ 2, while change in x is: y-1 ≤ x ≤ -3y + 7. Hence the double integral is:
\(\int\limits^2_1\int\limits^{-3y+7}_{y-1} {4y^2} \, dx dy\\\\=4\int\limits^2_1\int\limits^{-3y+7}_{y-1} {y^2} \, dx dy\\\\=4\int\limits^2_1y^2dy[x]^{-3y+7}_{y-1} \\\\=4\int\limits^2_1y^2dy(-3y+7-(y-1))\\\\=4\int\limits^2_1y^2dy(-4y+8)\\\\=4\int\limits^2_1(-4y^3+8y^2)dy\\\\=4[-y^4+\frac{8}{3}y^3 ]^2_1\\\\=4(\frac{11}{3} )\\\\=\frac{44}{3 }\)
To answer this question, we need, first get the equations for the lines that enclosed the surface, and integrate according to the limits obtained from these equations give.
The solution is:
A = 44/3 square units
Let´s call points:
P ( 0 , 1 ) Q ( 1 , 2 ) and R ( 4 , 1 )
The equation for the line between, P and R is:
y = 1
The equation for the line between, P and Q is:
Slope-intercept equation is y = m×x + b
The slope m₁ = ( 2 - 1 ) / ( 1 - 0 ) m₁ = 1
and the line passes over the point x = 0 y = 1 ; then
1 = 0 +b b = 1
y = x + 1 ⇒ x = y - 1
The equation for the line between Q and R is:
m₂ = ( 1 - 2 ) / ( 4 - 1) m₂ = - 1/3
y = ( -1/3)× x + b
when x = 1 y = 2
2 = ( - 1/3)×(1) + b
2 + 1/3 = b
b = 7/3
y = - (x/3) + 7/3 ⇒ x = 7 - 3×y
The double integral becomes:
A = 4×∫∫ y² dx dy ⇒ A = 4 ×∫₁² y²dy ∫dx | (y - 1 ) y ( 7 - 3y)
A = 4×∫₁² y²dy × x | ( y - 1 ) y ( 7 - 3y)
A = 4 ×∫₁² y²dy × [ 7 - 3×y - ( y - 1 )]
A = 4 ×∫₁² y²dy × (8 - 4×y ) ⇒ A = 4 ×∫₁² (8×y² - 4×y³ ) dy
A = 4 × [ (8/3)×y³ - y⁴ | ₁²
A = 4 × [ 64/3 - 16 - (8/3) + 1 ]
A = 4 × ( 56/3 - 15 )
A = 4 × ( 56 - 45 /3)
A = 4 × 11/3
A = 44/3 square units
Related Link :brainly.com/question/9825328

3 yellow marbles to 2 blue marbles, bag has 18 marbles, how many yellow marbles are in the bag?
Answers
Answer:
16 yellow marbles in total, because you subtract 2 from the 18 because then you just get the yellow marbles.
Sheila had some money in her
wallet. After she spent $12, she
had $9 left. (Use m as a
variable.)
Answers
a=3
Check Answer: 12-3=9
What is 5,382.619 rounded to the nearest 10,000
Answers
Answer:
10,00
Step-by-step explanation:
if its 50,000 its closest to 10,000
I need help with this problem

Answers
Answer:
please see answers below
Step-by-step explanation:
In a right-angled triangle, a ² + b ² = c ².
angles in a triangle add up to 180°.
Sine rule: a/SIN A = b/SIN B = c/SIN C
angle C = 90° (little square means 90).
if angle A is 30, then angle B must be 180 - 90 - 30 = 60°.
using the Sine rule:
a/Sin A = c/Sin C
multiply both sides by Sin A:
a = (c Sin A) / Sin C
= (12 Sin 30) / Sin 90
= 6.
so a = 6.
Using Pythagoras (In a right-angled triangle, a ² + b ² = c ²),
c² = a² + b²
b² = c² - a²
= 12² - 6²
= 144 - 36
= 108
so b² = 108
b = √108
angle B is correct
Please help me with this -w-

Answers
Answer: 250
Step-by-step explanation: You can round down 1014 to 1000, adn 1000 divided by 4 is 250.
Answer:
253.5 but round to 250
Step-by-step explanation:
1,014 /4=253.5
what is 11/2 times 1/3 as a fraction
Answers
Answer:
11/2 times 1/3 is 11/6
Step-by-step explanation:
11/2 times 1/3,
We have to multiply the numerators and denominators,
\((11/2)(1/3) = (11*1)/(2*3) = 11/6\\11/6\)
hence we get 11/6
In an apple harvest, for every 3 green apples there are 5 red apples. If the total number of
green apples harvested is 75, which of the following is the number of red apples harvested?
Answers
Answer:
125 red apples
Step-by-step explanation: 3x25=75 and 5X25=125
Please mark brainliest
You roll a 6-sided die.
What is P(not divisor of 6)?
Write your answer as a fraction or whole number.
Answers
1/3
Step-by-step explanation:The notation P(x) is used to describe the probability of event x occurring.
Divisors
Before we can do the probability question, we need to find the divisors of 6. Divisors are numbers that can evenly divide another number. The factors and divisors of a number are the same. So, 6 has 4 divisors:
1, 2, 3, and 6All of these values are on a 6-sided dice. So, in this probability question, there are 4 unsuccessful outcomes.
Probability
The probability of a simple event is the number of successful outcomes divided by the number of possible outcomes. In this situation, there are 6 possible outcomes, each side of the dice. Four of these outcomes do not satisfy the given condition (not a divisor of 6). Thus, there are 2 successful outcomes.
\(\frac{2}{6} =\frac{1}{3}\)The probability of rolling a number that is not a divisor of 6 is 1/3.
Solve –2(3x – 1) = 20 for x
Answers
Answer:
x = -3
Step-by-step explanation:
Distribution
3x(-2) -1(-2) = 20
-6x +2 = 20
Subtraction Property of Equality
-6x + (2 - 2) = (20 - 2)
-6x = 18
Division Property of Equality
(-6x/-6) = (18/-6)
x = -3
To check just plug in x
-6x = 18
x = 3
Which ratio represents the number of vowels to total letters in the word JACKSONVILLE?
Answers
Step-by-step explanation:
4:12 there are four vowels and 12 letters?
178.8 divided by 24 please help
Answers
Answer:
7.45
hope that answers ur question :D
what is the solution to 2x + 5 = 6x – 5? Show your work.
Answers
2x+5=6x−5 2x+5-5=6x - 5 - 5
Please answer for 20

Answers
Answer:
Step-by-step explanation:
im on the same one
sorry i couldent help
U
1
2.
3
4.
LO
What is the highest common factor (HCF) of 72 and 96?
1
Answers
Answer:
HCF of 72 and 96 is 24
Step-by-step explanation:
What is the highest common factor (HCF) of 72 and 96
HCF
Highest Common factor(HCF) is the biggest number that is divisible by both numbers.
First we will write prime factors of both 72 and 96
72: 2x2x2x3x3
96: 2x2x2x2x2x3
Now, writing the common factors: 2x2x2x3
Multiplying them we get: 24
So, HCF of 72 and 96 is 24
Select all the correct answers.
Which expressions are equivalent to the given expression.
y^-8y^3x^0x^-2
Answers
Answer:
\(x^{-2}y^{-5}\)
Step-by-step explanation:
The options are not given; however, the question can still be solved
Given
\(y^{-8}y^3x^0x^-2\)
Required
Simplify
Start by rewriting the expression
\(y^{-8} * y^3 * x^0 * x^{-2}\)
Apply the following laws of indices: \(a^x * a^y = a^{x+y}\)
This gives:
\(y^{-8+3} * x^{0-2}\)
Evaluate the exponents
\(y^{-5} * x^{-2}\)
\(x^{-2}y^{-5}\)
Hence, \(x^{-2}y^{-5}\) is equivalent to \(y^{-8}y^3x^0x^-2\)
Answer:
The answer is- (Drumroll)
Step-by-step explanation:
The answers are \(x^-2y^-5\), and \(1/x^2y^5\)
HELP!!!!!!!!!!!!!!! IT'S TIMED!!!!!!!!!!!!!!!!
Dr. Ellison says that the equation y = 17x + 1 has a solution of (1,18).
Is Dr. Ellison right or wrong?
a. HE'S RIGHT
B. he's wrong
c. you need more information
Answers
Answer:
The answer is A
Step-by-step explanation:
There are two solutions.
Slope = 17
y - intercept = (0,1)
The two solutions is
(0,1) and (1,18)
So he is right
Answer:
Step-by-step explanation:
a
Simplifying products and quotients of powers
7^2•7^8/7^4=7^a/7^4=7^b
A=
b=

Answers
Answer:
a=10
b=6
Step-by-step explanation:
add 2 to 8, to get a, then subtract 4 from 10 to get b
Answer:
10 and 6
Step-by-step explanation:

Diana can earn money for the tickets she sells. Which of the following statements describes the variables in this situation correctly?
The amount of money earned is the independent variable because it affects the number of tickets sold.
The amount of money earned is the dependent variable because it affects the number of tickets sold.
The number of tickets sold is the independent variable because it affects the amount of money earned.
The number of tickets sold is the dependent variable because it affects the amount of money earned.
Answers
Answer:
Option 3 is the correct answer.
Answer: The number of tickets sold is the independent variable because it affects the amount of money earned.
Step-by-step explanation: I just took test got 100%
The number of tickets sold is the independent variable because it affects the amount of money earned.
Which of the following represents the series? –13 + (–7) + (–1) + 5 + 11
Answers
Answer:
Adding +6 in turn
Step-by-step explanation:
-13+6=(-7)
(-7)+6=(-1)
(-1)+6=5
5+6=11
11+6=17
17+6=23
23+6=29
29+6=35
........... And so on
if it helped uh please mark me a brainliest :))Find the 97th of the arithmetic sequence 25, 29, 33, ...
Answers
Answer:
434
Step-by-step explanation:
hello :
note : the n th term of an arithmetic sequence is :
An =A1+(n-1)d ....a common difference is : d and A1 the first term
in this exercice : d = 33-29 =29-25= 4 A1 = 25
when : n =97 you have the 97rd term A97
so : A97 =25 +(97-1)(4)
A97 = 434