Answers
The diagram of the Gateway Arch on the coordinate plane, analyzed using quadratic equations indicates;
1. The vertex point is (50, 630)
2. The solution point are; (20, 0), and (80, 0)
3. Vertex form; f(x) = -0.7·(x - 50)² + 630
4. Factored form; f(x) = -0.7·(x - 20)·(x - 80)
What is a quadratic equation?A quadratic equation is an equation of the form f(x) = a·x² + b·x + c
1. The vertex obtained from the graphical diagram of the Gateway Arch indicates that the point corresponding to the vertex point is; (50, 9 × 70 = 630)
The vertex point is; (50, 630)
2. The solution are the points the curve of the Gateway intersects the x-axis, which are points where the y-axis values are zero, therefore;
The solutions are; (20, 0), and (80, 0)
3. The vertex form of a quadratic equation is; f(x) = a·(x - h)² + k
Where;
(h, k) = The coordinates of the vertex
Therefore;
(h, k) = (50, 630)
f(20) = 0 = a·(20 - 50)² + 630
a·(20 - 50)² = -630
a = -630/((20 - 50)²) = -630/900 = -7/10
a = -7/10 = -0.7
The vertex form quadratic equation is therefore; f(x) = -0.7·(x - 50)² + 630
4. The factored form of a quadratic equation is; f(x) = a·(x - r₁)·(x - r₂)
r₁ = 20, and r₂ = 80, a obtained from the vertex is; a = -0.7
The factored form is therefore; f(x) = -0.7·(x - 20)·(x - 80)
Learn more on the factored form of a quadratic equation here: https://brainly.com/question/25094938
#SPJ1
Related Questions
A 13km stretch of road needs repairs. Workers can repair 3 1/2 km of road per week.
How many weeks will it take to repair this stretch of road?
Answers
The computation shows that the number of weeks that it take to repair this stretch of road will be 4 weeks.
How to compute the number of weeks?From the information given, we are told that a thirteen kilometers stretch of road needs repairs and that the workers can repair three and half kilometers of road per week.
The question is simply for us to calculate the number or weeks that it will take to be able to repair the stretch of road.
Therefore, based on the information given, it should be noted that to get the number of weeks that will be used to repair the road, we simply have to divide the total distance or the road by the distance that is repaired every week.
Therefore, the number of weeks that it will take to be able to repair this stretch of road will be:
= 13/3.5
= 3.7
= 4 weeks approximately
Therefore, based on the information given, the number of weeks that's required is 4 weeks.
Learn more about computations on:
brainly.com/question/4658834
#SPJ1
shareholders. Calculate the amount of dividend r of dividend received by Bishwant. Curriculum Development Centre. Sanothimi. Bhaktanur 65 Vedanta Excel in Mathematics - Booeceived by Mrs. Rai. b) A Business Company sold 2,500 shares at Rs 1,200 per share. Bishwant bought 450 shares. If the company earned a net profit of Rs 39,00.000 in a year and it announced to distribute 18% dividend from the net profit to its shareholders, find the amount
Answers
3900000×18% = 702000
702000÷2500 = 280.8 per share dividend
450×280.8 = 126360
8x-2y
10 xy
(if x = 4 and y=-7) what is this evaluation
Answers
Answer: 2xy • (4x - 5y)
Step-by-step explanation:
STEP 1:
Equation at the end of step 1
((8 • (x2)) • y) - (2•5xy2)
STEP 2:
Equation at the end of step 2:
(23x2 • y) - (2•5xy2)
STEP 3:
STEP 4:
Pulling out like terms
4.1 Pull out like factors :
8x2y - 10xy2 = 2xy • (4x - 5y)
Final result :
2xy • (4x - 5y)
Looking for answer key to Gina Wilson All Things Algebra Unit 3 Parallel and Perpendicular Lines Homework 5 Slopes of lines; Parallel and Perpendicular Lines
Answers
It's important to work through the problems yourself to improve your understanding and problem-solving skills.
For the unit 3 homework on parallel and perpendicular lines, you should have learned about the slope of lines, how to find the slope of a line given two points, and the relationship between parallel and perpendicular lines.
To find the slope of a line given two points, you can use the slope formula:
m = (y2 - y1) / (x2 - x1)
where (x1, y1) and (x2, y2) are the coordinates of the two points on the line.
To determine if two lines are parallel, you can compare their slopes. Parallel lines have the same slope.
To determine if two lines are perpendicular, you can use the fact that the product of their slopes is -1. That is, if m1 and m2 are the slopes of two lines, and m1 * m2 = -1, then the lines are perpendicular.
It's important to practice working through problems using these concepts and formulas to develop your skills and understanding. Additionally, you can seek assistance from your teacher or tutor if you have specific questions or concerns.
To know more about slope of a line click here:
brainly.com/question/14511992
#SPJ4
Help me fast plsssssssssss

Answers
Answer:
PR= 4.2 AC= 18
Step-by-step explanation:
1)divide by same sides
QP/GF
3/5=0.6
in order to get PR
7(GH) *0.6 =4.2
BA/ML
30/50=0.6
in order to get AC
30(LN) * 0.6= 18
What is the solution to the system of equations?
y
+3
X = -2
15
o (-2, 3),
(2)
(-2공
ㅇ(-2, )
0

Answers
Answer:
d
Step-by-step explanation:
How to solve this question? Adding algebraic fractions

Answers
Answer:
it should be 2/12 and 9/11
Step-by-step explanation:
Find the side length of a cube with a volume of 141 f3 If necessary, round your answer to the nearest tenth.
Answers
The side length of the cube is 5.6 feet (rounded to the nearest tenth).
We can calculate the side length of a cube with a volume of 141 cubic feet using the formula for cube volume , which is \(V = s^3\), where V is the volume and s is the side length.
We can calculate s by taking the cube root of both sides of the equation:
\(s = (V)^{(1/3)\)
Substituting V = 141, we get:
\(s = (141)^{(1/3)\)
By using a calculator to evaluate this expression, we may determine:
s ≈ 5.6
As a result, the cube's side length is roughly 5.6 feet (rounded to the closest tenth). This indicates that if we increase the side length by three, it will become longer. (\(s^3\)), we will get the volume of the cube, which is 141 cubic feet.
for such more question on length
https://brainly.com/question/20339811
#SPJ11
Which is the sum of and 3/10 and 1/3
Answers
Step-by-step explanation:
sum=addition
3/10 +1/3
find the LCM of 10 and 3 Which is 30
9+10/30=19/30
I hope it helps ☺️
select all ordered pairs that are solutions of inequalities graphed aboveA. (-4, 2)B. (-1, -11)C. (4, 2)D. (0, 0)E. (-4, -2)

Answers
The solution must be inside the area in green
we have the next points
A. (-4, 2) point in yellow
B. (-1, -11) point in green
C. (4, 2) point in purple
D. (0, 0) point in red
E. (-4, -2) point in blue
as we can see the only points that are a solution
B. (-1, -11)
C. (4, 2)
D. (0, 0)

Solve by elimination.
4x + 9y
28
- 4x - y = -28
0.(-7,0)
(6,0)
O (7,0)
O (-6,0)
Answers
Answer:
(7,0)
Step-by-step explanation:
By Multiplying 4 and 7 (aka x), it will equal 28.
Multiplying 9 and y, (aka 0) , it will equal to 0
4(7)+9(0)=28
multiplying -4 by 7 will equal to -28 ,minus y which is 0, total will be -28
What is the solution to |x-2| + 3 > 17?
Ox<-12 or x > 16
Ox<-14 or x>7
O-12
O-14
Answers
Answer:
x<-12 or x>16
Step-by-step explanation:
Answer:
|x - 2| + 3 > 17
|x - 2| > 14
x - 2 < -14 or x - 2 > 14
x < -12 or x > 16
What is 420 in exponential form
Answers
Answer:
420 = 4.2 x 100 = 4.2 × 102
Step-by-step explanation:
Answer = 4.2x10^2
What is one way to add up 3-digit numbers?
Answers
Answer:
do it like step by step down below
Step-by-step explanation:
123
+ 240
+ 300
= 663
With lining them up
Step-by-step explanation:
Say you have 357 + 872
You would position your numbers so that they line up then you add the numbers that line up with each other, if your number is 10 or more you need to carry the first didgit over above the next row if your number was 14 you would carry the 1 over above the next row then you would add that number to the multiplicated 2 other numbers.
357
+ 872
_________
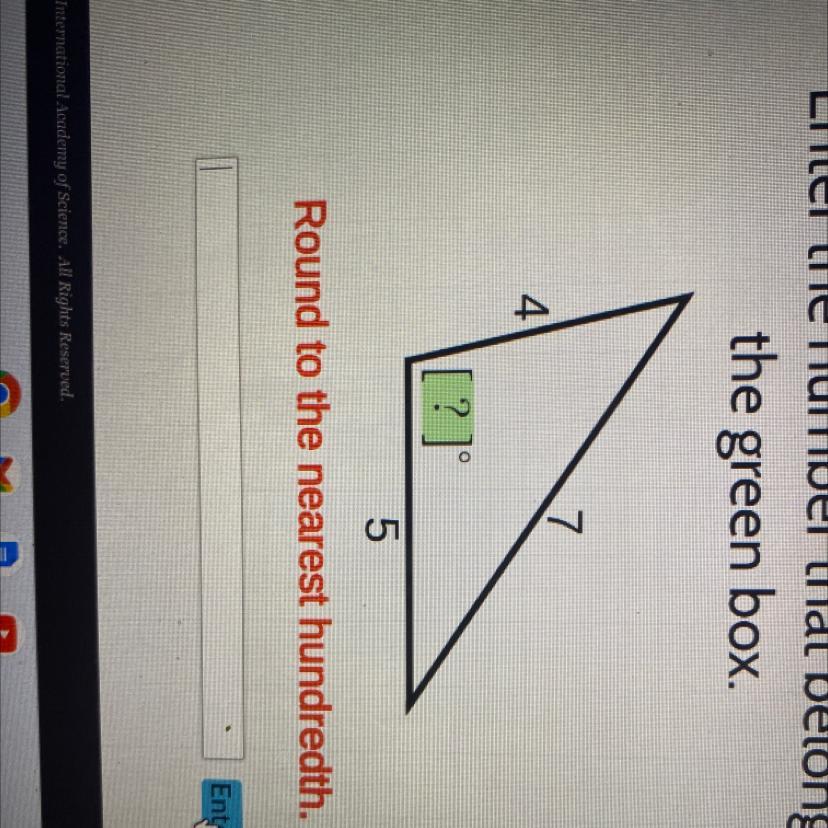
Enter the number that belongs in the green box
Answers
The angle between the sides measuring 4 and 5 in the obtuse triangle is approximately 101.54 degrees.
To find the measure of the angle between the sides measuring 4 and 5 in an obtuse triangle with side lengths 4, 5, and 7, we can use the Law of Cosines. The Law of Cosines states that in a triangle with side lengths a, b, and c, and an angle opposite to side c, the following equation holds:
\(c^2 = a^2 + b^2 - 2ab*cos(C)\)
In this case, we have side lengths a = 4, b = 5, and c = 7. We want to find the angle C, which is opposite to side c. Substituting these values into the Law of Cosines, we get:
\(7^2 = 4^2 + 5^2\)- 2(4)(5)*cos(C)
49 = 16 + 25 - 40*cos(C)
49 = 41 - 40*cos(C)
40*cos(C) = 41 - 49
40*cos(C) = -8
cos(C) = -8/40
cos(C) = -0.2
To find the measure of angle C, we can take the inverse cosine (arccos) of -0.2:
C = arccos(-0.2)
Using a calculator, we find that C ≈ 101.54 degrees.
Therefore, the measure of the angle between the sides measuring 4 and 5 in the obtuse triangle is approximately 101.54 degrees.
For more such information on: angle
https://brainly.com/question/25716982
#SPJ8
What is the equation of the line that is perpendicular to the given line and passes through the point (5, 3)?
4x – 5y = 5
Answers
Answer:
Step-by-step explanation:
-5y = -4x + 5
5y = 4x - 5
y = 4/5x - 1
perp. -5/4
y - 3 = -5/4(x - 5)
y - 3 = -5/4x + 25/4
y - 12/4 = -5/4x + 25/4
y = -5/4x + 37/4
The probability of winning a two-spot Keno game is 0.0601. A win occurs whenever both of the numbers picked by the player are pulled out of the hopper. What is the
probability of losing this game? Round to four decimal places. Put a leading zero on your answer; for example 0.0601.
Answers
The probability of losing the game is 0.9399. So, the answer is 0.9399.
The probability of losing the game is 0.9399. To determine the probability of losing, we must subtract the probability of winning from 1.
The probability of losing is equal to the complement of the probability of winning, and the complement of an event is the probability of the event not occurring.
Therefore, we will calculate the probability of the event not winning.
We know that the probability of winning a two-spot Keno game is 0.0601.
This means that the probability of not winning is 1 - 0.0601 = 0.9399.
Therefore, the probability of losing the game is 0.9399. So, the answer is 0.9399.
For more such questions on probability
https://brainly.com/question/25839839
#SPJ8
Please please look at the picture and answer the question thank you for your help

Answers
Answer:
20 for both
Step-by-step explanation:
hope this helps
Solve for x and graph the answer on a number line
2
Answers
The solution to the system of inequalities in interval notation is [-2, 1].
How to solve the system of inequalities?Based on the information provided in the image below, we have the following system of inequalities;
-12 < 3x - 6
-3 ≥ 3x - 6
By adding 6 to both sides of the equation (inequality), we have;
-12 < 3x - 6
-12 + 6 < 3x - 6 + 6
-6 < 3x
-2 < x
x > -2 (flip)
-3 ≥ 3x - 6
-3 + 6 ≥ 3x - 6 + 6
3 ≥ 3x
1 ≥ x
x ≤ 1
Therefore, the solution to the system of inequalities is given by:
-2 < x ≤ 1
In interval notation, we have [-2, 1].
Read more on inequality here: https://brainly.com/question/30665021
#SPJ1
Missing information:
The question is incomplete and the complete question is shown in the attached picture.


Evaluate the line integral ∫CF⋅dr, where F(x,y,z)=−xi−2yj−zk and C is given by the vector function r(t)=⟨sint,cost,t⟩, 0≤t≤3π/2.
Answers
Answer:
\(\mathbf { \int _ c Fdr= - \dfrac{9 \pi ^2}{8} - \dfrac{3}{2} }\)
Step-by-step explanation:
GIven that:
\(r(t) = (sin \ t, cos \ t, t)\)
\(r' (t) = (cos \ t, -sin \ \ t, 1)\)
\(F(x, y, z) = ( -x, -2y , - z)\)
\(F(r(t)) = ( sin \ t , - 2 cos \ t, - 1 t)\)
\(F(r(t)) \times r'(t) = (sin \ t, - 2 \ cos \ t , -1 \ t)( cos \ t , - sin \ t , 1 )\)
\(= sin t \ cos t + 2 \ sin t \ cos t - 1t\)
\(= 3sint \ cost - 1 t\)
\(\int _ c Fdr = \int ^b_a f(r(t)) \times r'(t) \ dt\)
\(= \int^{3 \pi/2}_0 \ [3 sin \ t \ cos \ t - 1 \ t ] \ dt\)
\(= 3 \int ^{3 \pi/2} _0 \ cos \ t ( sin \ t \ dt ) - 1 \int ^{3 \pi/2}_0 \ (t) \ dt\)
Let cos t = u &
sint dt = du
\(= 3 \int ^{3 \pi/2_} } _0 \ udu - 1 \int ^{3 \pi/2}_0 \ (t) \ dt\)
\(= 3 [ \dfrac{u^2}{2}]^{3 \pi/2}_0 - 1 [ \dfrac{t^2}{2}]^{3 \pi/2}_0\)
\(= \dfrac{3}{2} [ cos ^2 \ t ] ^{3 \pi/2} _0- \dfrac{1}{2} ( \dfrac{ 3 \pi }{2 })^2 - 0^2\)
\(= \dfrac{3}{2} [ cos ^2 \ \dfrac{3 \pi}{2} - cos ^2 \ 0 ] - \dfrac{1}{2}( \dfrac{9 \pi^2}{4})\)
\(= \dfrac{3}{2} ( 0 -1 ) - \dfrac{9 \pi^2}{8}\)
\(= - \dfrac{3}{2} - \dfrac{9 \pi ^2}{8}\)
\(\mathbf { \int _ c Fdr= - \dfrac{9 \pi ^2}{8} - \dfrac{3}{2} }\)
To answer this question, we apply:
∫CF×dr = ∫ c F (r(t)) × dr
Solution is:
( 1/2) - (9/8)×π
We know r(t) = sint i + cost j + t k
Then dr = ( cost i - sint j + k ) dt
And F ( x , y , z ) = -x i - 2y j - z k
Then F ( r(t)) = - sint i - 2 × cost j - t × k
And F ( r(t)) × dr = (- sint×cost + 2 ×sint ×cost - t ) dt
∫F (r(t)) × dr = ∫ (- sint×cost + 2 ×sint ×cost - t ) dt
Integration limits 0≤ t ≤ ( 3/2 ) π
∫ (- sint×cost + 2 ×sint ×cost - t ) dt = ∫ ( sint ×cost - t ) dt
∫ sint ×cost × dt - ∫ t × dt
∫F (r(t)) × dr = (1/2) sin²t - ( 1/2) × t² | 0 y (3/2) π
∫F (r(t)) × dr = (1/2)× ( -1)² - 0 - ( 9/8 ) × π - 0
∫F (r(t)) × dr = ( 1/2) - (9/8)×π
Related Link :https://brainly.com/question/3645828
please help and answer ASAP please will mark Brainlest
What is the value of x?
Enter your answer in the box.
x =
°

Answers
Answer:
80 degrees
Step-by-step explanation:
The sum of the angles in a triangle is 180 degrees so we can create the equation 70+30+x=180. This solves out to 80 so x=80 degrees.
Can someone please let me know what the answer is?

Answers
Answer:
D
Step-by-step explanation:
I'm not 100% sure but since when you remove the square roots on a and b, you have to add it to X
Answer:
B
Step-by-step explanation:
\(\dfrac{\sqrt{a}}{\sqrt{b}}=x\\\\Take \ square \ both \ sides,\\\\(\dfrac{\sqrt{a}}{\sqrt{b}})^{2}=x^{2}\\\\x^{2}=\dfrac{a}{b}\)
2x-9
X =
X +5
Use the triangle shown above to solve for x.
A

Answers
The value of x is 14.
We know in an isosceles triangle an isosceles polygon is a polygon with at least two sides of equal length.
We have two sides measured 2x-9 and x+ 5.
From the figure the length of sides are equal then
2x-9 = x+ 5
2x- 9- x = 5
x-9 = 5
Add 9 to both sides of the equation:
x - 9 + 9 = 5 + 9
x= 14
Thus, the value of x is 14.
Learn more about Equation here:
https://brainly.com/question/29538993
#SPJ1
model the unknown number problem by writing an algebraic expression. Then complete the statements
juan doubled his workout time
Answers
Measures of Variation, Standard Deviation, Population Variance, Population. Can someone help?

Answers
To solve this problem one must be aware of the concepts of standard deviation and variance of raw data.
There is a direct formulae to calculate the variance of the data,
Variance = \(σ^{2} =\frac{Sigma(xi-m)^{2}}{n}\)
Now here we need to verify ∑x and ∑\(x^{2}\).
∑x = 21+19+15+32+27
= 114
and ∑\(x^{2}\) = \(21^{2} +19^{2} +15^{2} +32^{2} +27^{2}\)
= 441+361+225+1024+729
= 2780
So, the given relation is true.
Now the variance of the given data by putting the values in the formulae is equal to 45.2.
And Standard deviation=\(\sqrt{Variance}\)
= \(\sqrt{45.2}\)
= 6.72
And for population α²=36.16 and deviation=6.01.
To learn more about the deviation and variance visit the link:
https://brainly.com/question/16555520
#SPJ9
What is 4 & 1/2+1/8? *

Answers
Answer:
4 5/8
Step-by-step explanation:
Rewriting our equation with parts separated
=4+12+18
Solving the fraction parts
12+18=?
Find the LCD of 1/2 and 1/8 and rewrite to solve with the equivalent fractions.
LCD = 8
48+18=58
Combining the whole and fraction parts
4+58=458
24,783 is invested, part at 15% and the rest 9%. If the interest earned from the amount invested at 15% exceeds the interest earned from the amount invested at 9% by $894.09, how much is invested at each rate?
Answers
If 24,783 is invested, part at 15% and the rest 9%. If the interest earned from the amount invested at 15% exceeds the interest earned from the amount invested at 9% by $894.09: 51,960 is invested at 15%, and 22,823 is invested at 9%.
How to find the amount invested?Let's x represent the amount invested at 15%
Let the amount invested at 9% = 24,783 - x.
Interest earned at 15% is 0.15 * x
Interest earned at 9% is 0.09 * (24,783 - x)
We can set up the equation: 0.15x = 0.09 * 24,783 - 0.09x + 894.09
Solving for x:
0.06x = 24,783 * 0.09 + 894.09
0.06x = 2223.47 + 894.09
0.06x = 3117.56
x = 51,960
So,
(24,783 - x)
24,783 - 51,960
= 22,823
Therefore 51,960 is invested at 15%, and 22,823 is invested at 9%.
Learn more about amount invested here:https://brainly.com/question/25300925
#SPJ1
Walking100
a) How many students ride in a tricycle, motorcycle and car going to their school
b) How many students ride in both a motorcycle and a tricycle?
c) How many students ride in both a motorcycle and a car?
d) How many students ride in both a car and tricycle?
e.)How many students go to school
in a car only;
in a motorcycle only;
30
Answers
Complete question :
The Venn diagram relating to the question can be found in the picture attached below :
Answer:
A.) 15 ; b.) 17 ; c.) 20 ; d.) 19 ; e.) 55 ; 67; 76 ;100 ; F.) 369
Step-by-step explanation:
Let :
Cars = C ; Motorcycle = M ; Tricycle = T ; Walking = W
a) How many students ride in a tricycle, motorcycle and car going to their school
Intersection of the 3 modes;
(C n M n T) = 15 ; it is the number which sits in between all the three circles.
B.) How many students ride in both a motorcycle and a tricycle?
(M n T) = 17 ; number in the middle of both circles representing motorcycle and tricycle
C.) How many students ride in both a motorcycle and a car?
(M n C) = 20 ; number in the middle of both circles representing motorcycle and Car
D) How many students ride in both a car and tricycle?
(C n T) = 19 ; number in the middle of both circles representing Car and tricycle
e.)How many students go to school
in a car only = 55
in a motorcycle only = 67
Tricycle only = 76
Walking = 100
F.) How many Grade Seven students of Koronadal National Comprehensive High School are there in all?
(100 + 67 + 76 + 55 + 19 + 20 + 17 + 15) = 369

a machinist is making a gear that will pull a chain at 60 ft/min when rotating at 40 rev/min. What should the radius of the gear be? Answer in inches.
Answers
The radius of the gear should be approximately 2.8644 inches.It is important to note that when making calculations involving units, we need to make sure that all units are consistent and convert them when necessary. In this case, we converted the answer from feet to inches to match the given unit.
To find the radius of the gear that will pull a chain at 60 ft/min when rotating at 40 rev/min, we can use the formula:
chain speed = 2 x pi x radius x rotational speed
where pi is a mathematical constant approximately equal to 3.14.
We know that the chain speed is 60 ft/min and the rotational speed is 40 rev/min, so we can substitute those values into the formula:
60 = 2 x 3.14 x radius x 40
Simplifying the equation, we get:
radius = 60 / (2 x 3.14 x 40)
radius = 0.2387 ft
To convert feet to inches, we can multiply the answer by 12:
radius = 0.2387 x 12 = 2.8644 inches.
For such more questions on Radius:
https://brainly.com/question/24375372
#SPJ11
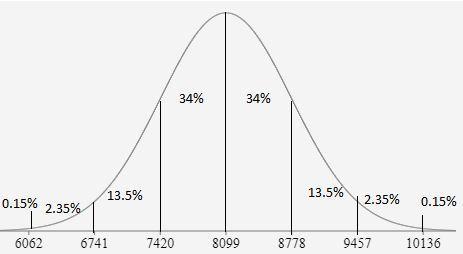
Lightbulbs. A company produces lightbulbs. We know that the lifetimes (in hours) of lightbulbs follow a bell-shaped (symmetric and unimodal) distribution with a mean of 7,161 hours and a standard deviation of 564 hours. Use the Empirical Rule (68-95-99.7 rule) to answer the following question: The shortest lived 2.5% of the lightbulbs burn out before how many hours
Answers
Answer:
Please find the complete question and its solution in the attached file.
Step-by-step explanation:
Shortest had survived after 6741 hours \(2.5\%\) of the lights burnt.
\(\to 0.15\% + 2.35\% = 2.50\%\)