This question refers to the population growth problem in section 3.9 of the lecture notes. Suppose that bacteria growth is modelled by the DE given in the notes. Suppose that the number of bacteria is observed to double after 4 days, and the estimated carrying capacity is 19 times the initial population. What is the estimated population, as a multiple of the initial population, after 18 days? (For example an answer of 3.5 would indicate a population 3.5 times the initial population). Give the answer accurate to 2 decimal places. Number
Answers
The given differential equation is,dP/dt = kP (1 - P/19) Where k is the constant of proportionality and P is the population at any time t.
Let P0 be the initial population. Then, the given statement that the number of bacteria is observed to double after 4 days can be written as,P(4) = 2P0So, P0 = P(4)/2 = 500
Now, the carrying capacity is 19 times the initial population, which is 19P0 = 19 × 500 = 9500. So, P cannot exceed 9500.As the initial population is P0, and the doubling time is 4 days, the time required for P to become 8P0 is 3 × 4 = 12 days. Since P cannot exceed 9500, the population after 18 days would have stabilised to 19P0 or 9500 (whichever is less).Now we need to estimate P(18). At t = 18, the population is given by,P(18) = 19P0 / [1 + (18/5) * e^(-k*18)]Since P0 = 500, we have to estimate the value of k.
To find k, use P(4) = 2P0 and P(12) = 8P0 to get two equations in k.
Substituting P0 = 500 and solving, we get,k = 0.26622 approx 0.27Putting this in P(18), we get,P(18) = 19*500 / [1 + (18/5) * e^(-0.27*18)]P(18) ≈ 5638.76Thus, the estimated population as a multiple of the initial population after 18 days is 5638.76 / 500 ≈ 11.28 (accurate to two decimal places).Hence, the required answer is 11.28.
#SPJ11
https://brainly.com/question/32643516
Related Questions
If
sales are $802,000, variable costs are 65% of sales, and income
from operations is $267,000, what is the contribution margin ratio?
a. 35% b. 65% c. 61% d. 39%
please show calculations
Answers
Answer:
a. 35%
Step-by-step explanation:
Sales = $802,000
Variable costs = 65% of sales = 0.65 x $802,000 = $521,300
Income from operations = $267,000
Contribution Margin = Sales - Variable Costs
= $802,000 - $521,300 = $280,700
Contribution Margin Ratio = (Contribution Margin / Sales) x 100
= ($280,700 / $802,000) x 100
= 35%
Therefore, the contribution margin ratio is approximately 35.02%.
Hello struggling with this question. I need to know what are intervals in math. Please answer the question with the image.

Answers
Answer:
b
Step-by-step explanation:
When you see things that move at a non linear rate, it means that it is not a straight line. Automatically, you can eliminate A and D because the lines there between those numbers are straight. Now we have two non linear lines left and there is only one that is decreasing (going down and it is the line between -2 and 0.
Hope this helped :)
I need help with this problem

Answers
Find dy/dx for the function y=3x^0

Answers
Answer:0
Step-by-step explanation:
So this is an exponential equation. And the dy/dx is impossible because anything time 0 is 0. So there is no Rise over run, most commonly known as your slope.
Option. A ) 0 is correct
It is given that,y = 3x⁰ = 3
we have asked to differentiate the function y with respect to x.
for constants, the slope is always zero and the graph looks like a line parallel to the x-axis
\(dy/dx\) = 0
therefore, the differentiation of y=3 is 0.
know more about the "slope of a line" here :
brainly.com/question/27877215
Dry ice is solid carbon dioxide. It’s temperature is about -98°C. how much less is this temperature than room temperature, which is about 20°C
Answers
To find how much less the temperature of dry ice is than room temperature, we need to subtract the temperature of dry ice from the temperature of room temperature.
The formula is:difference = room temperature - dry ice temperature
We can plug in the given values:
difference = 20°C - (-98°C)
We can simplify the expression by adding 98°C to both sides:
difference + 98°C = 20°C + 98°C
difference = 118°C
Therefore, the temperature of dry ice is 118°C less than room temperature.
At work, Amy receives $22.25 per hours for up to 40 hours per week. Any time beyond that is paid at a rate of 37.80 per hour . If she revived $1.173.50 in her paycheck , how much time did she work that week
Answers
Answer:
Amy worked 47 1/2 (47.5) hours
Step-by-step explanation:
(22.25) (40) = $890
1,173.50 - 890 = $ 283.50
283.50/37.80 = 7 1/2 hours
A box contains red, purple and green beads in the ratio 4:6:7 There are 1428 more green beads than red beads.
How many green beads are in the box?
Answers
Answer:
3332 green beads
Step-by-step explanation:
There are 1428 more green beads than red beads.
There are 3,332 greed beads in the box
Given :
A box contains red, purple and green beads in the ratio 4:6:7
From the given ratio,
number of red beads = 4x
number of purple beads= 6x
number of green beads= 7x
There are 1428 more green beads than red beads.
green beads =1428 + red beads
\(7x=1428+4x\\3x=1428\\x=476\)
we know that number of green beads= 7x
number of green beads= \(7(476)=3332\)
There are 3,332 greed beads in the box
learn more : brainly.com/question/20318626
Order these numbers from least to greatest.
46,012; 45,987; 459,870; 4,987
A. 45,987; 459,870; 46,012; 4,987
B. 4,987; 46,012; 45,987; 459,870
C. 46,012; 45, 987; 4, 987; 459,870
D. 4,987; 45,987; 46,012; 459,870
Answers
Which of the following are other names for the Fundamental Theorems of Calculus? The Fundamental Theorem of Calculus and the Integral Evaluation Theorem The Fundamental Theorem of Calculus The Fundamental Theorem of Calculus, Part One I and Part II
Answers
The other names for the Fundamental Theorems of Calculus are the Integral Evaluation Theorem and the Fundamental Theorem of Calculus, Part One and Part Two.
The Fundamental Theorem of Calculus is a significant concept in calculus that connects integration and differentiation. It essentially states that integration and differentiation are inverse operations of each other. The theorem has two parts: Part One and Part Two.
Part One of the Fundamental Theorem of Calculus states that if a function f(x) is continuous on the interval [a,b], then the definite integral of f(x) from a to b can be evaluated using an antiderivative of f(x) at the endpoints a and b.
Part Two of the Fundamental Theorem of Calculus, also known as the Integral Evaluation Theorem, extends the concept of Part One by stating that if F(x) is an antiderivative of f(x), then the definite integral of f(x) from a to b can be evaluated as the difference between the antiderivative evaluated at the endpoints a and b. This theorem is often used to evaluate definite integrals.
Therefore, the other names for the Fundamental Theorems of Calculus are the Integral Evaluation Theorem and the Fundamental Theorem of Calculus, Part One and Part Two.
These theorems are essential tools in calculus and are used to solve a wide range of problems in many areas of mathematics and science. Understanding and applying these theorems can help to simplify complex problems and enable accurate calculations of integrals.
To learn more about Fundamental Theorems of Calculus, refer here:
https://brainly.com/question/30761130#
#SPJ11
when the level of confidence and sample standard deviation remain the same, a confidence interval for a population mean based on a sample of n = 100 will be a. wider than, b. narrower than, or c. equal to a confidence interval for a population mean based on a sample of n = 50.
Answers
This is because as the sample size increases, the confidence interval becomes more precise and thus narrower.
When the level of confidence and sample standard deviation remains the same, a confidence interval for a population mean based on a sample of n = 100 will be narrower than a confidence interval for a population mean based on a sample of n = 50. This is because larger sample sizes typically result in more precise estimates of the population mean, leading to a smaller margin of error and therefore a narrower confidence interval.
This is because as the sample size increases, the confidence interval becomes more precise and thus narrower.
LEARN MORE ABOUT confidence interval
https://brainly.com/question/24131141
#SPJ11
How could you use the function y = sin 2x to find the zeros of y = tan 2x
Answers
To find the zeros of y = tan(2x), we need to solve the equation sin(2x) = 0 to determine the x-values where sin(2x) is zero. These x-values will correspond to the zeros of the function tan(2x).
To find the zeros of the function y = tan(2x), we can utilize the fact that tan(x) is equal to sin(x)/cos(x).
Given y = tan(2x), we can rewrite it as:
y = sin(2x) / cos(2x)
Now, let's consider the numerator of this expression, which is sin(2x). If we set sin(2x) equal to zero, we can determine the values of x that make the numerator zero:
sin(2x) = 0
By solving this equation, we can find the zeros of sin(2x). The solutions will be the values of x for which sin(2x) equals zero.
Similarly, we can look at the denominator of the expression y = sin(2x) / cos(2x), which is cos(2x). If cos(2x) equals zero, the denominator will be zero, which leads to undefined values for y. These points will be vertical asymptotes of the function tan(2x).
To find the zeros of y = tan(2x), we need to solve the equation sin(2x) = 0 to determine the x-values where sin(2x) is zero. These x-values will correspond to the zeros of the function tan(2x).
Learn more about function at https://brainly.com/question/30477134
#SPJ11
Sal's Sandwich Shop sells wraps and sandwiches as part of its lunch specials. The profit on every sandwich is $2, and
last month. The equation 2x + 3y = 1,470 represents Sal's profits last month, where x is the number of sandwich lunch
1. Change the equation to slope-intercept form. Identify the slope and y-intercept of the equation. Be sure to show
2. Describe how you would graph this line using the slope-intercept method. Be sure to write using complete sente
3. Write the equation in function notation. Explain what the graph of the function represents. Be sure to use comples
4. Graph the function. On the graph, make sure to label the intercepts. You may graph your equation by hand on a
5. Suppose Sal's total profit on lunch specials for the next month is $1,593. The profit amounts are the same: $2 for
sentences, explain how the graphs of the functions for the two months are similar and how they are different.
02.03 Key Features of Linear Functions-Option 1 Rubric
Requirements
Student changes equation to slope-intercept form. Student shows all work and identifies the slope and y-intercept of the
Student writes a description, which is clear, precise, and correct, of how to graph the line using the slope-intercept meth
Student changes equation to function notation. Student explains clearly what the graph of the equation represents.
Student graphs the equation and labels the intercepts correctly.
Student writes at least three sentences explaining how the graphs of the two equations are the same and how they are different.
Answers
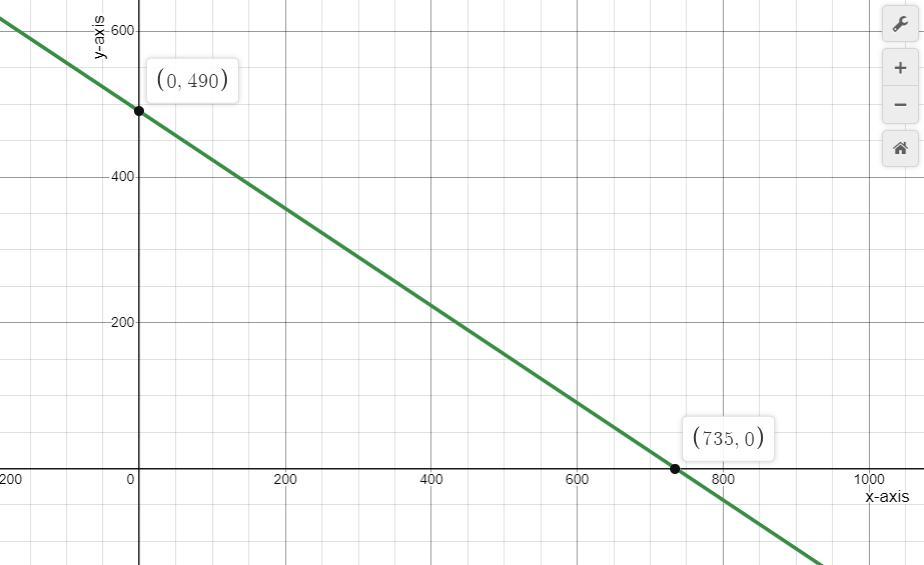
1. The equation to slope-intercept form is y = -2/3(x) + 490. The slope is -2/3 and the y-intercept is 490.
2. You should start at the y-intercept (0, 490) and move right by 3 units and downward by 2 units, and then connect the points.
3. The equation in function notation is f(x) = -2/3(x) + 490. The graph of the function is the rate of change with respect to the number of sandwich lunch sold.
4. A graph of the function with intercepts is shown below.
5. The graphs of the functions for the two months both have the same slope but different y-intercept and x-intercept.
How to change the equation to slope-intercept form?In Mathematics and Geometry, the slope-intercept form of the equation of a straight line is given by this mathematical equation;
y = mx + b
Where:
m represent the slope or rate of change.x and y are the points.b represent the y-intercept or initial value.Based on the information provided above, a linear equation that models Sal's Sandwich Shop's profit is given by;
2x + 3y = 1,470
By subtracting 2x from both sides of the equation and dividing by 3, we have:
2x + 3y - 2x = 1,470 - 2x
y = -2/3(x) + 490
Therefore, the slope is -2/3 and the y-intercept is 490.
Part 2.
In order to graph the equation by using the slope-intercept method, you would start at the y-intercept (0, 490) and move right by 3 units and down by 2 units, and then connect the points.
Part 3.
Next, we would write the equation in function notation as follows;
f(x) = -2/3(x) + 490
where:
f(x) represents the number of wrap lunch sold.x is the number of sandwich lunch sold.The graph represents the rate of change of the function with respect to the number of sandwich lunch sold.
Part 4.
In this context, we would use an online graphing calculator to plot the linear function as shown in the image attached below.
Part 5.
Assuming Sal's total profit on lunch specials for the next month is $1,593 and the profit amounts remain the same, a system of equations to model this situation is given by:
2x + 3y = 1593; y = -2/3(x) + 531.
2x + 3y = 1,470; y = -2/3(x) + 490.
In conclusion, we can logically deduce that the graphs of the functions for the two months both have the same slope but different y-intercept and x-intercept.
Read more on slope-intercept here: brainly.com/question/7889446
#SPJ1
Complete Question:
Sal's Sandwich Shop sells wraps and sandwiches as part of its lunch specials. The profit on every sandwich is $2, and the profit on every wrap is $3. Sal made a profit of $1,470 from lunch specials last month. The equation 2x + 3y = 1,470 represents Sal's profits last month, where x is the number of sandwich lunch specials sold and y is the number of wrap lunch specials sold.
Mr. G eats the same amount of gummy bears each hour. He made a table showing
the number of gummy bears he eats. Write a linear equation in slope-interd zoom in
form to represent the number of gummy bears, g, that Mr. G has eaten at any
time, h, in hours.
Time (hours) X
0
1
2
3
4
Gummy bears eaten Y
0
4
8
12
16
Slope-Intercept Form
y = mx + b
HARIBO
Goldbears

Answers
The equation of the number of gummy bears in the slope-intercept form is g = 4x
How to determine the equation of the line?From the question, we have the following table of values that represents the given parameter that can be used in our computation:
Time (hours) X Gummy bears eaten Y
0 0
1 4
2 8
3 12
4 16
On the table, we have the following point
(x, y) = (0, 0) and (1, 4)
A linear equation is represented as
y = mx + c
Where
c = the y-intercept
i.e. c represents when the value of x is 0
This means that
c = 0
So, we have
y = mx + 0
y = mx
Recall that we have
(1, 4)
This means that
4 = 1 * m
So, we have
m = 4
So, the equation is y = 4x
Read more about linear equation at
https://brainly.com/question/2030026
#SPJ1
The average length of a baby sunfish in the east town hatchery is 2.2 inches with a standard deviation of 0.6 inches. Assume the population is bell shaped. Approximately what percentage of fish have z-scores because 2 and -2?
Answer:
68%
75%
88.9%
95%
99.7%
Answers
In the east town hatchery, around (d) 95% of the fish will have z-scores between 2 and -2.
A z-score is a measure of how far a specific point is away from the mean in terms of standard deviations. A z-score of 2 means that the point is 2 standard deviations above the mean, while a z-score of -2 means that the point is 2 standard deviations below the mean.
In this case, the mean length of a baby sunfish is 2.2 inches and the standard deviation is 0.6 inches. Therefore, a z-score of 2 means that the fish is 2 * 0.6 = 1.2 inches above the mean, while a z-score of -2 means that the fish is 2 * 0.6 = 1.2 inches below the mean.
The 68-95-99.7 rule tells us that approximately:
68% of the fish will have z-scores between -1 and 1.
95% of the fish will have z-scores between -2 and 2.
99.7% of the fish will have z-scores between -3 and 3.
Therefore, approximately (d) 95% of the fish in the east town hatchery will have z-scores between 2 and -2.
To know more about z-score refer here :
https://brainly.com/question/33911012#
#SPJ11
3) Find the equation of the plane Ax+By+Cz=D_through the points P(1, −1,2), Q(−1,0,1) and R(1,−1,1)
Answers
We are given three points, P(1, -1, 2), Q(-1, 0, 1), and R(1, -1, 1), and are asked to find the equation of the plane that passes through these points.
To find the equation of the plane, we can use the point-normal form of a plane, which states that a plane can be defined by a point on the plane and the normal vector perpendicular to the plane. To find the normal vector of the plane, we can use the cross product of two vectors that lie on the plane. Let's take two vectors, PQ and PR, where PQ = Q - P and PR = R - P. We can calculate the cross product of PQ and PR to obtain the normal vector.
PQ = (-1 - 1, 0 - (-1), 1 - 2) = (-2, 1, -1)
PR = (1 - 1, -1 - (-1), 1 - 2) = (0, 0, -1)
Normal vector N = PQ x PR = (-2, 1, -1) x (0, 0, -1) = (1, -2, -2)
Now that we have the normal vector, we can substitute the coordinates of one of the points, let's say P(1, -1, 2), and the normal vector (A, B, C) into the point-normal form equation: A(x - x1) + B(y - y1) + C(z - z1) = 0, where (x1, y1, z1) is the point on the plane.
Substituting the values, we have A(1 - 1) + B(-1 - (-1)) + C(2 - 2) = 0, which simplifies to A(0) + B(0) + C(0) = 0. This implies that A, B, and C are all zero.
Therefore, the equation of the plane passing through the points P(1, -1, 2), Q(-1, 0, 1), and R(1, -1, 1) is 0x + 0y + 0z = D, or simply 0 = D.
To know more about equation click here: brainly.com/question/29657983
#SPJ11
This is Evaluating Functions.Replace the X values with the number in the question ("Find f( )" )


Answers
Given the following function:
f(x) = 3x - 3
Let's determine the value at f(-3),
This means that we will be replacing all x variables of f(x) by -3.
We get,
f(x) = 3x - 3
f(-3) = 3(-3) - 3
= -9 - 3
f(-3) = -12
Therefore, f(-3) = -12
Given the following function:
f(x) = 3x - 3
Let's determine the value at f(-3),
This means that we will be replacing all x variables of f(x) by -3.
We get,
f(x) = 3x - 3
f(-3) = 3(-3) - 3
= -9 - 3
f(-3) = -12
Therefore, f(-3) = -12
the number n can be expressed as the product of four prime numbers, exactly three of which are the same. how many different positive divisors does n have, including n and 1 ?
Answers
Answer:
8
Step-by-step explanation:
Give a prime factorization consisting of two primes, with one of them cubed, you want to know the number of positive integer divisors.
DivisorsThe number of divisors is the product of the exponents of the prime factorization, each increased by 1.
ApplicationYour number is ...
n = (a^3)(b^1)
so the number of positive divisors is ...
(3+1)(1+1) = 4·2 = 8
The number n has 8 different positive divisors.
__
Additional comment
The divisors are ...
1, a, b, a², ab, a³, a²b, a³b
Example: 24 = 2³·3 has divisors ...
1, 2, 3, 4, 6, 8, 12, 24
Which expression is equivalent to startfraction 3 x over x 1 endfractiondivided by x 1?
Answers
The expression that is equivalent to [3/(x + 1)] ÷ 1/(x + 1)] after simplification is gotten as; 3
How to simplify fractions?We want to simplify the expression;
[3/(x + 1)] ÷ 1/(x + 1)]
This fraction expression will be re-expressed as;
[3/(x + 1)] * (x + 1)/1
x + 1 will cancel out to give us the expression as;
[3/(x + 1)] * (x + 1)/1 = 3
Read more about simplification of fractions at; https://brainly.com/question/6109670
Answer:
Step-by-step explanation:
c
Select the correct answer.
Consider the given functions.
A table with values of x and f of x is given along with a non-linear equation g of x equals 2 power x minus 5 and a graph solving the equation
Select the true statement regarding the end behavior of the functions.

Answers
Using limits, it is found that the true statement regarding the end behavior of the function is given by:
B. As the value of x increases, f is the only function to approach 0.
How to find the end behavior of a function?The end behavior of a function is given by the limit of the function as x goes to infinity.
In this problem, we have that:
\(\lim_{x \rightarrow \infty} f(x) = 0\), as we can see from the table, when x increases, y decreases. \(\lim_{x \rightarrow \infty} g(x) = 2^{\infty} = \infty\).\(\lim_{x \rightarrow \infty} h(x) = \infty\), from the graph.Hence statement B is correct.
More can be learned about limits at https://brainly.com/question/22026723
#SPJ1
assuming that if a logical vector z has at least one entry true, which of the function will always be false ? group of answer choices any(!z) all (!z) any(z) all(z)
Answers
If a logical vector z has at least one entry TRUE, the function all(!z) will always be false.
If a logical vector contains only TRUE items, the all() method returns FALSE; otherwise, it returns TRUE.
The any() function, on the other hand, gives a result of TRUE if a logical vector has at least one element that is TRUE and FALSE otherwise.
any(z) will always be TRUE if z contains at least one TRUE element since there is at least one TRUE element. On the other hand, depending on whether all of the components of z are TRUE, all(z) may or may not be TRUE.
any(!z) will always return FALSE since if z has at least one TRUE element, then!z must include at least one FALSE element.
Additionally, all(!z) will always return FALSE if any element is FALSE since if z has at least one TRUE element, then!z must include at least one FALSE element.
Learn more about propositional logic in discrete mathematics here:
https://brainly.com/question/1103576
#SPJ4
Dr black is standing 13 feet from a streetlamp. The lamp is making his shadow 9 feet long. He estimates that the angle of elevation from the tip of his shadow to the top of the streetlamp is 50° to the nearest foot the streetlamp is about
Answers
Dr black is standing 13 feet from a streetlamp. The lamp is making his shadow 9 feet long. He estimates that the angle of elevation from the tip of his shadow to the top of the streetlamp is 50° to the nearest foot the streetlamp is about 26 feet tall.
I hope this helps!
6 boxes of 3-inch incense sticks for $22.75 is a better buy than 8 boxes for $30 and 10 boxes for $38.00
True
False
Answers
Answer:
Step-by-step explanation:
22.75/6 boxes is $3.79 per box
30/8 boxes is $3.75 a box
38/10 boxes is $3.80 a box
68/18=3.70 a box if you combine the last two.
So no, the 6 boxes at $3.79 a box is not a better buy.
It costs $150.00 to rent the bowling alley, plus $8.50 per person. What would be the total
cost to rent the bowling alley for 25 people?
Answers
Answer: $362.50.
8.5 x 25 = 212.5,
212.5 + 150.0 = 362.50
Very simple, I hope this helps!
What does the Angle-Angle Criterion tell you about the relationship between two triangles?The first choose options (all 3 angles, 2 angles) Second choose options (all 3 angles, only 1 angle, 2 angles )The third choose options (congruent, similar)

Answers
the answers are
1) all 3 angles
2) all 3 angles
3) similar
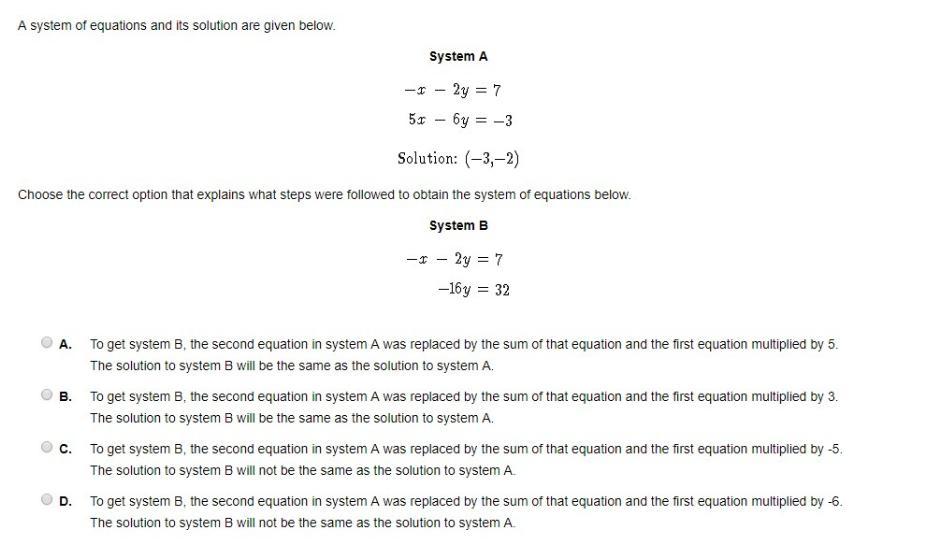
Select the correct answer. A system of equations and its solution are given below. System A Choose the correct option that explains what steps were followed to obtain the system of equations below. System B A. To get system B, the second equation in system A was replaced by the sum of that equation and the first equation multiplied by 3. The solution to system B will be the same as the solution to system A. B. To get system B, the second equation in system A was replaced by the sum of that equation and the first equation multiplied by -5. The solution to system B will not be the same as the solution to system A. C. To get system B, the second equation in system A was replaced by the sum of that equation and the first equation multiplied by 5. The solution to system B will be the same as the solution to system A. D. To get system B, the second equation in system A was replaced by the sum of that equation and the first equation multiplied by -6. The solution to system B will not be the same as the solution to system A.
Answers
The correct option that explains what steps were followed to obtain the system of equations include the following: A. To get system B, the second equation in system A was replaced by the sum of that equation and the first equation multiplied by 5. The solution to system B will be the same as the solution to system A.
How to solve the given system of equations?In order to solve the given system of equations, we would apply the elimination and substitution method. Based on the information provided above, we have the following system of equations:
System A:
-x - 2y = 7 ..........equation 1.
5x - 6y = -3 .......... equation 2.
Next, we would multiply the first equation by 5 as follows
5(-x - 2y) = 5(7)
-5x - 10y = 35 ........... equation 3.
By taking the sum of equation (2) and equation (3), we have:
(5x - 5x) + (-6y - 10y) = 35 - 3
-16y = 32 .......... equation 4.
By replacing the second equation in system A by equation 4, we have system B:
-x - 2y = 7
-16y = 32
y = 32/-16
y = -2.
When y = -2, the value of x is given by;
-x - 2y = 7
x = -7 - 2y
x = -7 - 2(-2)
x = -7 + 4
x = -3
Therefore, the solution to system B is the same as the solution to system A i.e (-3, -2).
Read more on equation here: https://brainly.com/question/10681451
#SPJ1
Missing information:
The question is incomplete and the complete question is shown in the attached picture.
Jada keeps 175 beads in a storage box. She chooses a bead without looking what
color it is, and returns it to the box. She does this severaltimes. The table shows results
Pink - 7
Green - 8
Red - 10
How many pink beads are in Jadas storage box
Answers
There will be 49 pink beads in Jada's storage container.
What is the anticipated outcome?The weighted sum is applied to the expected value in parameter estimation. Informally, the expected value is the simple average of a large number of outcomes that were chosen at random and were calculated independently.
A bundle from Jada holds 175 hair beads. Without even glancing at the beads, she chooses one, notes the color, and puts it back in the box. She speaks more than once in the same way. The table below displays the results. Jada's cousin desires to have pink beads placed in her hair.
The expected value is given below.
E(x) = np
Where n is the number of samples and p is the probability.
The table is given below.
Color Frequency
Pink 7
Green 8
Red 10
Total 25
The probability of getting pink will be given as,
p = 7/25
p = 0.28
Then the expected value of pink will be given as.
E(pink) = 175 x 0.28
E(pink) = 49
Thus, the number of pink beads in Jada's storage box will be 49.
More about the expected value link is given below.
To learn more about 'storage box' refer to
brainly.com/question/13945225
#SPJ1
if the probability of winning a teddy bear at a carnival is 0.89 and 40 turns are taken how many teddy bears will be won
Answers
If probability of winning a teddy bear at a carnival is 0.89 and 40 turns are taken, then he should have won about 35 teddy bears.
The probability of winning a teddy bear at a carnival is = 0.89, then
The probability of not winning a teddy bear is = 1 - 0.89 = 0.11,
We use binomial-distribution to calculate the probability of winning "k" teddy bears in "n" turns,
Where probability of winning (p) = 0.89,
The formula for the binomial distribution is,
⇒ P(k) = C(n,k) × p^k × (1-p)^(n-k),
The probability of winning "k" teddy bears in 40 turns is :
⇒ P(k) = C(40,k) × 0.89^k × 0.11^(40-k),
To find "expected-number" of teddy bears won, we multiply the probability of winning k teddy bears by k, and sum over all possible values of k,
⇒ E(number of teddy bears won) = \(\[ \sum_{k=0}^{40} \]\) k × P(k),
⇒ \(\[ \sum_{k=1}^{40} \]\) k × C(40,k) × 0.89^k × 0.11^(40-k),
On further solving ,
We get,
⇒ E(number of teddy bears won) ≈ 35.6,
Therefore, we expect to win about 35 teddy bears if we take 40 turns .
Learn more about Probability here
https://brainly.com/question/4293666
#SPJ4
a linear function has an x-intercept of −8 − 8 and a y-intercept of 4 4 . which of these is an equation of the linear function?
Answers
So, y = (1/2)(x + 8) is the equation of the linear function.
To write the equation of a linear function with a given x-intercept and y-intercept, we can use the point-slope form of a linear equation:
y - y1 = m(x - x1)
In this case, we are given that the x-intercept is (-8, 0) and the y-intercept is (0, 4). We can use the coordinates of the x-intercept as (x1, y1) and the slope of the line as m to write the equation:
y - 0 = m(x - (-8))
y = m(x + 8)
We can then substitute the y-intercept into this equation to find the value of m:
4 = m(0 + 8)
4 = 8m
m = 1/2
Substituting the value of m back into the equation, we get:
y = (1/2)(x + 8)
This is the equation of the linear function.
To learn more about Linear Function,
visit; brainly.com/question/20286983
#SPJ4
y - y1 = m(x - x1)
y - 0 = m(x - (-8))
y = m(x + 8)
We can then substitute the y-intercept into this equation to find the value of m:
4 = m(0 + 8)
4 = 8m
m = 1/2
Substituting the value of m back into the equation, we get:
y = (1/2)(x + 8)
Please help! Correct answers only please!
A student was conducting a study to determine how many mini chocolate chip cookies would fit in a plastic sealed container. He used random packing to find the following.
Using the collected data above what would be a reasonable rough estimate of a ratio of how many cubic inches per cookie?
A. 2.2 in^3/cookie
B. 0.45 in^3/cookie
C. 17.82 in^3/cookie
D. 23.76 in^3/cookie

Answers
Answer: (A) \(2.2\text{ in}^3/\text{cookie}\) .
Step-by-step explanation:
From the given table , the dimension of the container for the 90cookies = 11 inch x 6 inch x 3 inch
Then, the volume of the container would be \(11\times6\times3= 198 \ in^3\)
Then, the ratio of cubic inches per cookie would be
\(=\dfrac{\text{Volume of container}}{\text{Number of cookies in it}}\\\\=\dfrac{198}{90}\\\\=2.2\text{ in}^3/\text{cookie}\)
So, the the ratio of cubic inches per cookie would be \(2.2\text{ in}^3/\text{cookie}\) .
Hence, the correct option is (A) \(2.2\text{ in}^3/\text{cookie}\) .
I need help, please! ASAP.

Answers
Answer:
Formula for percent change is C = {x_2 - x_1}/{x_1}
C = relative change
x_1 = initial value
x_2 = final value
Step-by-step explanation:
Answer:
<_> daddy
Step-by-step explanation: