Answers
Answer:
x = 0
Step-by-step explanation:
Given the diagram, we know that the left side is the same as -2x + 6 and the right side as -6. We can write an equation and solve for x.
-2x + 6 = 6
~Subtract 6 to both sides
-2x = 0
~Divide -2 to both sides
x = 0
Best of Luck!
Answer:
x=0
Step-by-step explanation:
-2x+6=-6
-2x=0
x=0
Related Questions
What is limit of startfraction 6 minus x over x squared minus 36 endfraction as x approaches 6? negative startfraction 1 over 12 endfraction 0 startfraction 1 over 12 endfraction dne
Answers
Answer:
As x approaches 6:
\( \frac{6 - x}{ {x}^{2} - 36 } = - \frac{x - 6}{(x - 6)(x + 6)} = - \frac{1}{x + 6} = - \frac{1}{6 + 6} = - \frac{1}{12} \)
The limit of the given function as x approaches 6 is -1/12. This is achieved by factoring and revising the original function, and then substituting into the revised function.
Explanation:The student is asking for the limit of the function (6-x) / (x²-36) as x approaches 6. In mathematics, this is a problem of calculus and specifically involves limits. Let's solve this by first factoring the denominator to get (6-x) / ((x-6)(x+6))
By realizing we can revise the numerator as -(x-6), we make it obvious that the limit can be directly computed by substituting x=6 after canceling out the (x-6) terms. The result is -1/12, therefore the limit of the function as x approaches 6 is -1/12.
Learn more about limits here:https://brainly.com/question/37042998
#SPJ11
The diagram shows a circle with center O and radius 42 inches.
A and B are points on the circumference such that arc AB makes an angle of 120°
Calculate the length of the arc AB.
Use 22/7 as an approximation for IT
A 88 inches
B 132 inches
C176 inches
D 264 inches

Answers
1. Fine the missing numbers
A. A worm can move 20cm in one second. It’s speed is what ?
B. An elevator can travel 500m in one minute. It’s speed is what ?
C. A cheetah can run 120km in one hour . It’s speed is what ?
D. Ben runs at a speed of 4m/s. He runs what ? m in one second?
E. A snail moves at a speed of 32cm/min.it moves what cm in one minute?
F. An aeroplane travels at speed of 965km/h. It travels what ? Km in one hour ?
Answers
A. If a worm can move 20cm in one second, its speed is 20cm/s (20cm per second).
B. If an elevator can travel 500m in one minute, its speed is 500m/m (500 meters per minute).
C. If a cheetah can run 120km in one hour, its speed is 120km/h (120 kilometers per hour).
D. If Ben runs at a speed of 4m/s. He runs 4 m in one second.
E. If a snail moves at a speed of 32cm/min, it moves 32 cm in one minute.
F. If an airplane travels at speed of 965km/h. It travels 965 Km in one hour.
What is the speed?The speed refers to the quotient of the total distance and the time.
The speed describes the unit rate at which an object or person moves or operates or is moved or operated.
Thus, the speed can be described as the rate of change of distance with respect to time.
Learn more about the speed at https://brainly.com/question/13943409.
#SPJ1
1. For each of the following, find 2 solutions and 2 non solutions.
a. 2x + 5y = 30
Answers
The following, find 2 solutions and 2 non solutions is \(x=\frac{30-5 y}{2}\)
What is non solutions?
Ideal solutions are those that follow Raoult's law throughout the whole concentration range. A solution is said to as a non-ideal solution if it violates Raoult's law.
\($$2 x+5 y=30$$\)
\(Move $5 y$ to the right side\)
\($$2 x=30-5 y$$\)
\(Divide both sides by 2\)
\($$\frac{2 x}{2}=\frac{30}{2}-\frac{5 y}{2}$$Simplify$$x=\frac{30-5 y}{2}$$\)
Non solutions, in the plural, refer to anything that falls short of offering a fix for a problem. These batteries also lose their charge, rendering them useless for solving the more significant issue of storing and utilizing renewable energy.
To learn more about non solutions visit
https://brainly.com/question/3184550
#SPJ1
Please help my pick A B C OR D

Answers
the ans is (A) . -2x^3 + 8x^2 - 3.
Answer:
ANSWER IS OPTION A OR -3X^3+8X^2-3
HELP HOW DO I SOLVE THIS
Josie wants to buy Internet access. One service provider charges a flat rate of $34.95/month. A second charges $25/month plus 33c/h. For what number of hours per month should Josie choose the flat rate?
Answers
ansewer:
25+.33h=34.95 solving for h gives h=(34.95-25)/.33 =30.15 hours. So if you are going to use more than 30 hours it better to go with the flat rate company.
Step-by-step explanation:
You want to find, h, the number of hours of usage that will make the costs for the two equal so
What is the vertex of the graph of f x )=[ x 5 ]- 6?
Answers
The vertex of the graph of f(x) = |x - 5| - 6 is (5, -6). It lies in the fourth quadrant.
Therefore the answer is (5, -6).
The graph f(x) = |x - 5| - 6 is symmetrical about the axis x = 5.
A vertex of a graph is a node of a graph. For a graph of the form f(x) = a|x - h| + k, the vertex is (h, k). So in this case where f(x) = |x - 5| - 6, a = 1, h = 5 and k = -6. Therefore the vertex of the given graph f(x) is
(5, -6)
--The question is incomplete, answering to the question --
"What is the vertex of the graph of f(x) = |x - 5| - 6?"
To know more on vertex
https://brainly.com/question/28885437
#SPJ4
There are 28 brown ducks swimming in a pond. Twice as many white ducks flew in and landed in the pond. Then half of the ducks flew away. How many ducks remained in the pond?
Answers
Answer:
42 ducks remain in the pond
Step-by-step explanation:
First, find the number of white ducks
28*2=56
Add them to the brown ducks
56+28= 84
Then divide by 2 because half flew away
84/2=42
So 42 ducks remain in the pond. Hope this helps :)
A study was done by an online retail store to determine the rate at which users used its website. A graph of the data that was collected is shown: What can be interpreted from the range of this graph?
The range represents the 54-month time period of the study.
The range represents the 36-month time period of the study.
The range represents the number of users each month for 36 months. The range represents the number of users each month for 54 months.

Answers
Answer:
The correct answer is C.
Step-by-step explanation:
First, we can eliminate A and D since it is obviously only 36 months.
Note that the question asks what can be interpreted from the range of the graph.
The range is the number of users in thousands (per month) (as can be seen from the side title).
While B is technically correct, it wrongly interprets the range. C is the best choice.
The correct answer is C.
A tv has a screen that is 15 in long and has a 25 in long diagonal find the area of the tv screen?

Answers
Answer:
300 in²
Step-by-step explanation:
The TV screen is rectangular in shape.
Thus:
Length of the rectangular screen = 15 in.
Diagonal of the rectangular screen = 25 in.
To find the area of the rectangular screen, we need the length and the width of the screen.
Use pythagorean theorem to find the width as shown below:
\( a^2 + b^2 = c^2 \), where:
a = width = ?
b = 15 in.
c = 25 in. (The hypotenuse/longest side)
Plug in the values into the equation
\( a^2 + 225 = 625 \)
Subtract 225 from each side
\( a^2 = 400 \)
Take the square root of both sides
\( a = 20 \)
width of the screen = 20 in.
Thus,
Area of the screen = length × width
= 15 × 20
Area = 300 in²
Consider the following sequence.
64, -32, 16, -8,...
Hence find the next three terms of the sequence.

Answers
Answer:
4,-2,1
Step-by-step explanation:
Keep dividing the previous term by -1/2
So we get
4,-2,1
What is the present value of $12,200 to be received 4 years from today if the discount rate is 5 percent? Multiple Choice $10,027.51 $7,320.00 $10,459.53 $10,538.82 $10,036.97
Answers
Answer; present value of $12,200 to be received 4 years from today, with a discount rate of 5 percent, is $10,027.51.
The present value of $12,200 to be received 4 years from today can be calculated using the formula for present value. The formula is:
Present Value = Future Value / (1 + Discount Rate)^n
Where:
- Future Value is the amount to be received in the future ($12,200 in this case)
- Discount Rate is the interest rate used to discount future cash flows (5 percent in this case)
- n is the number of periods (4 years in this case)
Plugging in the given values into the formula:
Present Value = $12,200 / (1 + 0.05)^4
Calculating the exponent first:
(1 + 0.05)^4 = 1.05^4 = 1.21550625
Dividing the future value by the calculated exponent:
Present Value = $12,200 / 1.21550625
Calculating the present value:
Present Value = $10,027.51
Therefore, the present value of $12,200 to be received 4 years from today, with a discount rate of 5 percent, is $10,027.51.
Learn more about present value calculations:
https://brainly.com/question/12736329
#SPJ11
Which of the following expressions represents sin x + sin y= + y2? 0 A dy = 2.2-COSI 2y-cosy dz 0 В. dy 2x+cos3 2y+cos y du o dy de 2y-cos y 2.1-COSI O D dy 2.1-cos2 2y-cosy O E dy d 2r+COST 2y+cos y
Answers
- [(2x - cos x) / (2y - cos y)] of the following expressions represents sin x + sin y = x² + y², using the concept of differentiation.
What is differentiation?One method is to determine a function's derivative by differentiation. Finding the instantaneous rate of change of a function depending on one of its variables is a technique known as differentiation in mathematics.
Differentiation refers to the process of calculating a function's derivative. The derivative, when applied to two variables x and y, is the rate at which x changes in relation to y. To calculate the immediate rate of change of quantities, differentiation is used.
sin x + sin y = x² + y²
Differentiating w.r.t x
d/dx (sin x + sin y) = d/dx (x² + y²)
or, cos x + cos y dy/dx = 2x + 2y dy/dx
or, cos y dy/dx - 2y dy/dx = 2x - cos x
or, dy/dx (cos y - 2y) = 2x - cos x
or, dy/dx = (2x - cos x) / (cos y - 2y)
or, dy/dx = - [(2x - cos x) / (2y - cos y)]
To know more about differentiation refer to:
https://brainly.com/question/954654
#SPJ4
Between the ages of 22 and 50, how much money would john spend on his habit if he continues to smoke two packs a day and each pack costs $5 how much will he spend if he smokes at the same rate from 22 to 70
Answers
Between the ages of 22 and 50, John would spend $102,200 on smoking, and between the ages of 22 and 70, he would spend $175,200 on smoking.
1. First, find the number of years John smokes between ages 22 and 50, and between ages 22 and 70:
- For ages 22 to 50: 50 - 22 = 28 years
- For ages 22 to 70: 70 - 22 = 48 years
2. Calculate the number of packs John smokes per year, given that he smokes 2 packs a day:
- 2 packs/day × 365 days/year = 730 packs/year
3. Calculate the cost of smoking per year, given that each pack costs $5:
- 730 packs/year × $5/pack = $3,650/year
4. Calculate the total cost of smoking for both age ranges:
- For ages 22 to 50: 28 years × $3,650/year = $102,200
- For ages 22 to 70: 48 years × $3,650/year = $175,200
If John continues to smoke at the same rate, he would spend $102,200 on smoking between the ages of 22 and 50, and $175,200 if he continues until the age of 70.
To know more about cost visit:
https://brainly.com/question/14566816
#SPJ11
Ten years ago, you could buy a loaf of bread for $1.21. Twenty years ago, you could buy a loaf of bread for $0.92.
Answers
Answer:
$0.29 is the price difference
Step-by-step explanation:
Ten years ago, you could buy a loaf of bread for $1.21. Twenty years ago, you could buy a loaf of bread for $0.92.
ABCD is a trapezium.
10 cm
A
B
7 cm
9 cm
D
С
15 cm
Calculate the area of ABCD.

Answers
Answer:
87.5
Step-by-step explanation:
For what values of a is F = (x² + yz)i + a(y + 2zx)j + (xy+z)k a conservative vector field? For this value of a, find a potential such that F= Vy. (b) A particle is moved from the origin (0, 0)
Answers
(a) For a = 1, the vector field F is conservative, (b) For a = 1, the potential function V such that F = ∇V is: V = (1/3)x³ + xy z + (y²/2 + 2xyz) + xyz + z²/2 + C
To determine the values of a for which the vector field F = (x² + yz)i + a(y + 2zx)j + (xy+z)k is conservative, we need to check if the curl of F is zero. If the curl is zero, then F is conservative.
The curl of a vector field F = P i + Q j + R k is given by the following determinant:
curl(F) = ( ∂R/∂y - ∂Q/∂z ) i + ( ∂P/∂z - ∂R/∂x ) j + ( ∂Q/∂x - ∂P/∂y ) k
The curl of F:
∂R/∂y = 1
∂Q/∂z = a
∂P/∂z = -2ax
∂R/∂x = y
∂Q/∂x = 0
∂P/∂y = 0
Plugging these values into the curl formula, we have:
curl(F) = (1 - a) i + (-2ax) j + y k
For the curl to be zero, each component of the curl must be zero. Therefore, we have the following conditions:
1 - a = 0 (from the i-component)
-2ax = 0 (from the j-component)
y = 0 (from the k-component)
From the first condition, we find that a = 1.
Substituting a = 1 into the second and third conditions, we have:
-2x = 0
y = 0
∴ x = 0 and y = 0.
Therefore, the vector field F is conservative for a=1.
To obtain a potential function V such that F = ∇V, we integrate each component of F with respect to the corresponding variable:
V = ∫(x² + yz) dx = (1/3)x³ + xy z + g(y,z)
V = ∫a(y + 2zx) dy = a(y²/2 + 2xyz) + h(x,z)
V = ∫(xy + z) dz = xyz + z²/2 + k(x,y)
Combining these terms, we have:
V = (1/3)x³ + xy z + a(y²/2 + 2xyz) + xyz + z²/2 + C
Therefore, for a = 1, the potential function V such that F = ∇V is:
V = (1/3)x³ + xy z + (y²/2 + 2xyz) + xyz + z²/2 + C
To know more about potential function refer here:
https://brainly.com/question/28156550#
#SPJ11
On a strange railway line, there is just one infinitely long track, so overtaking is impossible. Any time a train catches up to the one in front of it, they link up to form a single train moving at the speed of the slower train. At first, there are three equally spaced trains, each moving at a different speed.
Answers
In the given scenario, where there is one infinitely long track and overtaking is impossible, the initial situation consists of three equally spaced trains, each moving at a different speed. The trains have the capability to link up when one catches up to the other, resulting in a single train moving at the speed of the slower train.
As the trains move, they will eventually reach a configuration where the fastest train catches up to the middle train. At this point, the fastest train will link up with the middle train, forming a single train moving at the speed of the middle train. The remaining train, which was initially the slowest, continues to move independently at its original speed. Over time, the process continues as the new single train formed by the fastest and middle trains catches up to the remaining train. Once again, they link up, forming a single train moving at the speed of the remaining train. This process repeats until all the trains eventually merge into a single train moving at the speed of the initially slowest train. In summary, on this strange railway line, where trains can only link up and cannot overtake, the initial configuration of three equally spaced trains results in a sequence of mergers where the trains progressively combine to form a single train moving at the speed of the initially slowest train.
Learn more about speed here:
https://brainly.com/question/13263542
#SPJ11
The Jones family was one of the first to come to the U.S. They had 9 children. Assuming that the
probability of a child being a girl is .5, find the probability that the Jones family had:
at least 7 girls?
at most 8 girls?
Answers
Answer:
At least 7 girls: 0.5^7 OR 0.0078125
At least 8 girls: 0.5^8 OR 0.00390625
Answer quickly please I really need this answer right now
A bag contains:
• 5 red marbles
• 6 blue marbles
• 3 green marbles
• 2 yellow marbles
• 4 purple marbles
A student draws a random marble from the bag, records its color, and returns
it to the bag. The student makes a total of 60 draws. What is a reasonable
prediction for the number of times a green marble will be drawn?
O A. 18
O B. 10
Ос. 9
O D. 12
Answers
Answer:
Answer Choice A (8, eight)
Step-by-step explanation:
To begin, add all of the marbles together. When finished, the answer should be 20. Because the variable 20 equals 100, you divide 4 by 100. You will get 0.04 as a result. This multiplied by 20 equals 8. Finally, the answer is A.
-[-/7.14 Points] DETAILS LARPCALC11 6.4.036. Find the angle (in radians) between the vectors. (Round your answer to two decimal places.) u = 4i - 6j v = 5i + 4j 0 =
4. [-/7.14 Points] DETAILS LARPCAL
Answers
The angle (in radians) between vectors u and v is approximately 1.66 radians, rounded to two decimal places.
To find the angle between two vectors, we can use the dot product formula and the magnitudes of the vectors. Let's calculate the angle between vectors u and v:
Given:
u = 4i - 6j
v = 5i + 4j
Step 1: Calculate the dot product of u and v.
The dot product of two vectors u and v is given by:
u · v = |u| |v| cosθ
where |u| and |v| are the magnitudes of vectors u and v, respectively, and θ is the angle between them.
To calculate the dot product, we multiply the corresponding components of u and v and sum them:
u · v = (4 * 5) + (-6 * 4) = 20 - 24 = -4
Step 2: Calculate the magnitudes of vectors u and v.
The magnitude of a vector is given by:
|u| = √(u₁² + u₂²)
For vector u:
|u| = √((4)² + (-6)²) = √(16 + 36) = √52 ≈ 7.21
For vector v:
|v| = √((5)² + (4)²) = √(25 + 16) = √41 ≈ 6.40
Step 3: Calculate the angle θ.
Using the dot product formula mentioned earlier, we have:
-4 = (7.21)(6.40)cosθ
Solving for cosθ:
cosθ = -4 / (7.21 * 6.40) ≈ -0.086
To find θ, we can take the inverse cosine (arccos) of cosθ:
θ ≈ arccos(-0.086) ≈ 1.66 radians
Know more about vectors here:
https://brainly.com/question/24256726
#SPJ11
determine the equation of the circle graphed below.
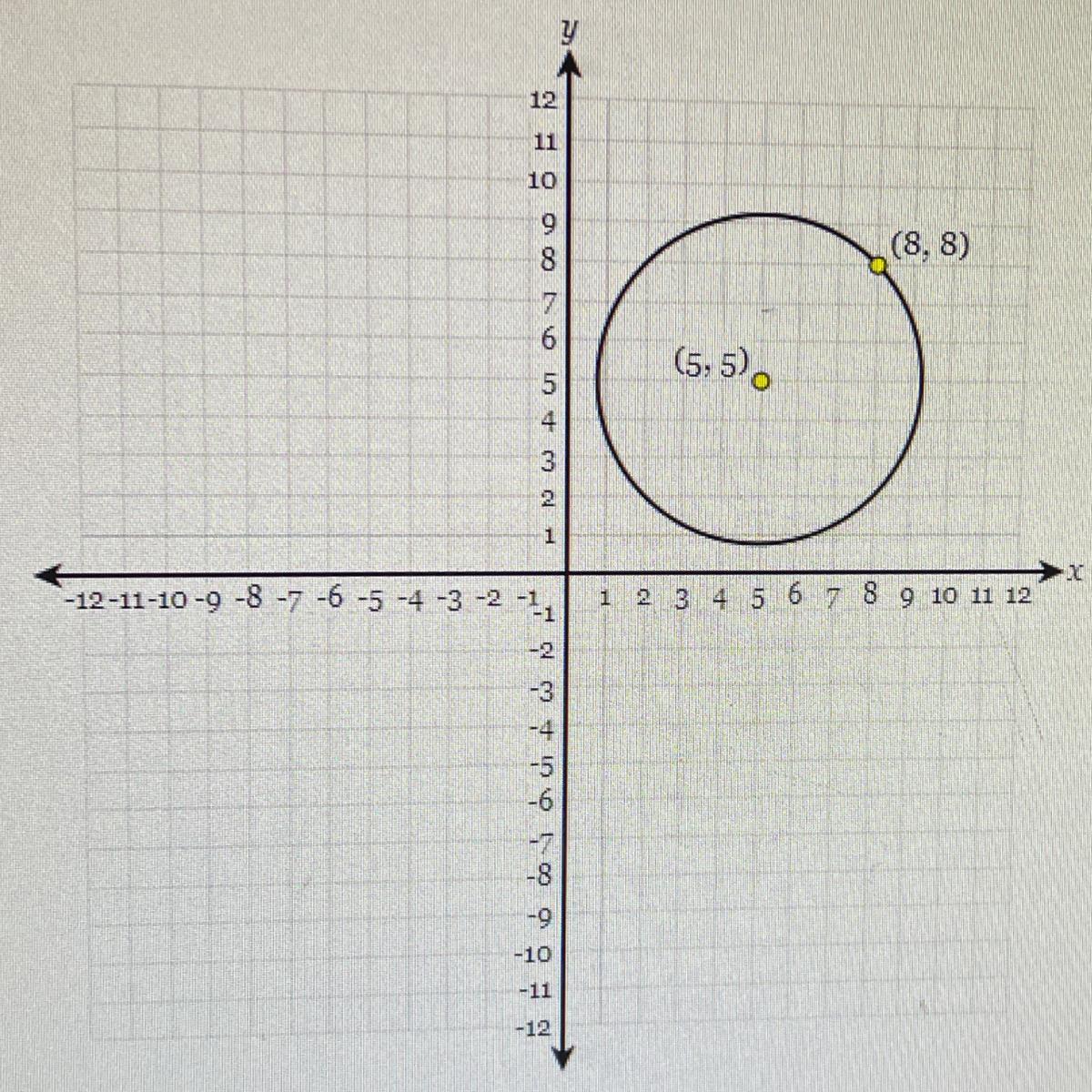
Answers
Answer:
(x - 5)² + (y - 5)² = 18
Step-by-step explanation:
The equation of a circle in standard form is
(x - h)² + (y - k)² = r²
where (h, k) are the coordinates of the centre and r is the radius
Here (h, k ) = (5, 5) , then
(x - 5)² + (y - 5)² = r²
r is the distance from the centre to a point on the circle
Calculate r using the distance formula
r = \(\sqrt{(x_{2}-x_{1})^2+(y_{2}-y_{1})^2 }\)
with (x₁, y₁ ) = (5, 5) and (x₂, y₂ ) = (8, 8)
r = \(\sqrt{(8-5)^2+(8-5)^2}\)
= \(\sqrt{3^2+3^2}\)
= \(\sqrt{9+9}\)
= \(\sqrt{18}\) ⇒ r² = (\(\sqrt{18}\) )² = 18
Then
(x - 5)² + (y - 5)² = 18 ← equation of circle
Two variables are (always | sometimes | never) correlated.
Answers
always
Write an equation in point-slope form for each pair of points. (4,6) and (10,30)
Answers
The equation of point slope form of (4,6) and (10,30) is -4x + y + 10 = 0.
How to find the equation of point slope form?A straight line is represented using its slope and a point on the line using point slope form. In other words, the point slope form is used to determine the equation of a line whose slope is "m" and passes through the point (x1, y1).
given that
the coordinates points are (4, 6) and (10, 30)
(x1,y1) = (4,6)
(x2, y2) = (10,30)
The formula of point-slope is
(y - y1) = m(x - x1)
Here,
x1 = 4 and y1 = 6 but we don't know the value of
m.
now,find the value of m using the following formula
\( m = \frac{(y2 - y1)}{(x2 - x1)}\)
now, substitute the values, we get
\(m = \frac{(30 - 6)}{(10 - 4)}\)
\(m = \frac{24}{6}\)
m = 4
so, the value of m is 4.
substitute these values into the point-slope formula
(y - 6) = 4(x - 4)
y-6 = 4x - 16
y = 4x -16 + 6
y = 4x - 10
-4x + y + 10 =0
Hence, the equation of point slope form of (4,6) and (10,30) is -4x + y + 10 = 0.
Learn more about point-slope from here:
https://brainly.com/question/24907633
#SPJ4
1. A group of friends share a large popcorn at the movies. It costs $4.95 to buy the first bag and $0.65 for each refill.
If they spent $6.90, how many times did the group of friends refill their popcorn?
Answers
% .65
ANSWER- 3 times
helpppp anyoneee please!!

Answers
Answer:x=6
Step-by-step explanation:
So if y is 0, that’s 8(y) which is 0. That means the equation is 2x=12, which means x is 6
My comments don’t work! But it’s (6,0)
y−5=2/3(x+4) what is the slope of this equation please.
Answers
Answer:
2/3
Step-by-step explanation:
The equation is in point slope form
\(y-y_{1} =m(x-x_{1} )\)
m is the slope, which is 2/3
Answer:
Slope is 2/3
Step-by-step explanation:
Point-slope formula is in the form
y - y1 = m(x - x1)
y - 5 = 2/3(x + 4)
Your equation has the
(x1, y1) and slope, m filled in.
The m is 2/3.
The slope is 2/3.
What type of conic section is defined by the equation?

Answers
so in the template above for a conic, the defining components are the coefficients A and C
If either A=0 or C=0, then the equation is a parabola
if A=C, then we have a Circle
if either A or C is negative, and A≠C, then we have a hyperbola
if A and C are both positive or both negative, and A≠C, then we have an ellipse.
the median of $20, x, 15, 30, 25$ is $0.4$ less than the mean. if $x$ is a whole number, what is the sum of all possible values of $x$?
Answers
The sum of all possible values of x is 19.4
The term median is referred by putting the numbers in increasing order and then taking the middle number if n is odd. where as If n is even then the median is the sum of two middle numbers divided by 2.
Here we have given that the median of 20, x, 15, 30, 25 is 0.4 less than the mean and we need to find the value of x.
As per the given question we know that x is the whole number
And the given sequence is written as,
=> 20, x , 15, 30, 25
Here we also know that the median is less that the mean by 0.4.
In order to find the median, we have to arrange the given data,
=> 15, x, 20, 25, 30.
Then the value of x is calculated as,
=> 20 - 0.4
=> 19.4
To know more about Median here.
https://brainly.com/question/28060453
#SPJ4
i need help !! asap please !!!

Answers
a=62°
B=62°
C=62°
D=62°
