PLEASE HELP QUICK!! TEST IS TIMED!! Terri wrote the equation using slope-intercept form for the line that passes through the points (4, 6) and (–2, 3).
Step 1: m = StartFraction 4 minus (negative 2) Over 6 minus 3 EndFraction = StartFraction 6 Over 3 EndFraction = 2
Step 2: 3 = 2 (negative 2) + b
Step 3: 3 = negative 4 + b. 7 = b.
Step 4: y = 2 x + 7
Which best describes Terri’s first error?
In step 1, the slope of the line should be One-half.
In step 2, she should have substituted the point (4, 6).
In step 3, she should have subtracted 4 from both sides of the equation.
In step 4, the m and b values should be switched.
Answers
From the calculation, you can see that Terri's slope should have been one-half instead of 2. In step 1, the slope of the line should be One-half.
How to find the equation of a line?The standard equation of a line is expressed as y = mx + b
where
m is the slopeb is the y-interceptGiven the coordinate points (4,6) and (-2, 3)
Determine the slope
Slope = 3-6/-2-4
Slope = -3/-6
Slope = 1/2
From the calculation, you can see that Terri's slope should have been one-half instead of 2
Learn more on equation of a line here: https://brainly.com/question/13763238
#SPJ1
Related Questions
What value of b^2 is needed for there to be exactly one real solution of a quadratic equation? Explain.
Answers
Answer:
4ac
Step-by-step explanation:
Assuming you're referring to the equation \(ax^2+bx+c=0\).
Since the discriminant \(D=b^2-4ac\) has to be equal to 0 in order for there to be exactly one real solution, then we have the following:
\(0=b^2-4ac\\b^2=4ac\)
Therefore, b² needs to be the same value as 4ac.
The population on a certain island increased from 1500 in 2000 to 1577 in 2001 a. Determine the growth rate b. Write a general equation for the popolation p(t) c. Estimate the population in 2010 d. How many years will it take for the population to double?
Answers
Therefore, it will take approximately 13.7 years for the population to double.
a. To determine the growth rate, you need to calculate the percentage increase in population. The formula for growth rate is:
Growth Rate = (New Value - Old Value) / Old Value * 100
Using the given values, we have:
Growth Rate = (1577 - 1500) / 1500 * 100
Growth Rate = 77 / 1500 * 100
Growth Rate ≈ 5.13%
b. To write a general equation for the population, you can use the formula:
p(t) = p(0) * (1 + r/100)^t
where p(t) is the population at time t, p(0) is the initial population, r is the growth rate, and t is the number of years.
c. To estimate the population in 2010, we need to find the population at time t = 2010 - 2000 = 10 years. Using the general equation from part b, and substituting the given values:
p(10) = 1500 * (1 + 5.13/100)^10
p(10) ≈ 1500 * (1.0513)^10
p(10) ≈ 1500 * 1.6436
p(10) ≈ 2465.4
Therefore, the estimated population in 2010 is approximately 2465.
d. To find out how many years it will take for the population to double, we need to solve the equation:
2 * p(0) = p(0) * (1 + r/100)^t
Simplifying the equation, we have:
2 = (1 + r/100)^t
Taking the logarithm of both sides, we get:
log(2) = t * log(1 + r/100)
Finally, solving for t, we have:
t = log(2) / log(1 + r/100)
Substituting the growth rate from part a, we have:
t = log(2) / log(1 + 5.13/100)
t ≈ log(2) / log(1.0513)
t ≈ 13.7 years
Therefore, it will take approximately 13.7 years for the population to double.
To know more about population visit :
https://brainly.com/question/19169926
#SPJ11
Please help meeee !!!!!

Answers
Answer:
you can buy more bananas
Step-by-step explanation:
7 divided by 0.40 is 17.5 so you can buy 17 pounds
7 divided by 0.56 is 12.5 so you can buy 12 pounds
A store sells grape jelly in 15-ounce jars for $13.59. Find the price per ounce
Answers
Answer:
91 cents per ounce
Step-by-step explanation:
Divide 13.59 by 15
Answer: $0.91
Step-by-step explanation:
Take $13.59 divided by 15 = $0.91 per ounce
If you can, please give me a Brainliest, I need 2 more to get to the highest rank, thank you!
Evaluate (y - 10) - 8x
when x = 4 and y = 16
Answers
Answer:
-26 :)
Step-by-step explanation:
Fill in y and x with the subs
do parentheses which is 6
6-8(4) - do 8 times 4 which is 32
then 6-32 which is -26
(16 - 10) - 8*4
(6) - 32
6 - 32
-26
CAN ANYONE PLS PLS PLS HELP ME THIS IS A DISASTER AND I WIL GET PUNISHED IF NO DONE.

Answers
(Written in alphabetical order, watch your negatives, some things to keep in mind are - x - = + and -/- = + and - - = +)
What Do You Want To Learn About In Math This Year
Answers
Answer:
math
Step-by-step explanation:
a- What are the different logs used to obtain porosity? Explain the principles of measurement, quantity measured and its relation to porosity. Illustrate with equations and/or sketches.
Answers
Different logs measure different physical properties of the formation that are related to porosity, and the relationship between the log measurement and porosity can be expressed through equations.
The different logs used to obtain porosity and their principles of measurement, quantities measured, and relation to porosity.
There are three main types of logs used to obtain porosity: neutron logs, density logs, and sonic logs.
Neutron logs: These logs measure the hydrogen content in a formation, which is directly related to porosity. The tool emits neutrons that interact with hydrogen atoms, and the resulting gamma rays are measured. The count rate decreases with increasing porosity. The neutron log can be expressed as:
Porosity = (neutron log reading - matrix reading) / (fluid reading - matrix reading)
Density logs: Density logs measure the bulk density of a formation, which can be related to porosity. The tool emits gamma rays that interact with the formation, and the scattered gamma rays are measured. The bulk density decreases with increasing porosity. The density log can be expressed as:
Porosity = (matrix density - bulk density) / (matrix density - fluid density)
Sonic logs: Sonic logs measure the travel time of sound waves through a formation, which can be related to porosity. The tool sends an acoustic wave and measures the time it takes to travel a specific distance. The travel time increases with increasing porosity. The sonic log can be expressed using Wyllie's time-average equation:
Porosity = (sonic log reading - matrix reading) / (fluid reading - matrix reading)
In summary, neutron logs measure hydrogen content, density logs measure bulk density, and sonic logs measure travel time of sound waves. These measurements can be used to calculate porosity using the respective equations provided.
for more questions on porosity
https://brainly.com/question/3107253
#SPJ11
Sierra is building a skateboard ramp.
She wants the ramp to have a height, h, of 15 inches and a base length, l, of 54 inches.
Which linear function models the situation?
Α. y = 5/18x
B. y = 18/5x
C. y = 5x + 18
D. y=18x + 5
Answers
Answer: Here is a step by step
Step-by-step explanation:
We can use the formula for the slope-intercept form of a linear function, which is:
y = mx + b
where m is the slope and b is the y-intercept.
In this situation, the ramp has a height, h, of 15 inches when the base length, l, is 54 inches. We can use this information to find the slope of the linear function as follows:
m = rise / run = h / l = 15 / 54
To simplify this fraction, we can divide both the numerator and denominator by 3:
m = 5 / 18
So the slope of the linear function is 5/18.
Now we need to find the y-intercept, b. We know that when the base length, l, is 0, the height, y, is also 0 (since there is no ramp). This gives us:
y = mx + b
0 = (5/18)(0) + b
b = 0
Therefore, the linear function that models this situation is:
y = (5/18)x
So the answer is option A: y = 5/18x.
About 2 out of every 15 people are left-handed. how many of the 650 seniors in our school would you expect to be left-handed? set up the proportion and round to the nearest whole number.
Answers
You would expect approximately 87 seniors in your school to be left-handed.
To determine the number of left-handed seniors in your school, you can set up a proportion using the given information.
The proportion can be set up as follows:
(left-handed seniors) / (total number of seniors) = (left-handed people in the population) / (total population)
From the given information, we know that about 2 out of every 15 people are left-handed. This can be expressed as:
2/15 = (left-handed seniors) / (total number of seniors)
We are given that there are 650 seniors in your school. Let's denote the number of left-handed seniors as 'x'.
Substituting the known values into the proportion, we get:
2/15 = x / 650
To solve for 'x', we can cross-multiply:
2 * 650 = 15 * x
1300 = 15x
Dividing both sides of the equation by 15:
1300 / 15 = x
x ≈ 86.67
Rounding to the nearest whole number, we find that you would expect approximately 87 seniors in your school to be left-handed.
The proportion allows us to determine the expected number of left-handed seniors based on the known proportion of left-handed people in the population. By setting up and solving the proportion, we can estimate the number of left-handed seniors in your school to be approximately 87.
To learn more about proportion, click here: brainly.com/question/870035
#SPJ11
Mr. Garcia has 11 boys and 14 girls in his math class. He selects two students at random to demonstrate how they solved the day’s challenge assignment. What is the probability that both students chosen in a row are girls? (Put your answer in percent form.)
Answers
The probability that both students are girls is 3/10 or 0.30 if Mr. Garcia has 11 boys and 14 girls in his math class. He selects two students at random to demonstrate how they solved the day’s challenge assignment.
What is probability?It is defined as the ratio of the number of favorable outcomes to the total number of outcomes in other words the probability is the number that shows the happening of the event.
We have:
Total number of boys in Garcia's math class = 11
Total number of girls in Garcia's math class = 14
Total number of students = 11+14 ⇒ 25
Total number of outcomes = \(_{25}C_{2}\)
Total number of favorable outcomes = \(_{14}C_2\)
Now, the probability,
\(=\frac{_{14}C_2}{_{25}C_2}\)
The value of \(_{25}C_{2}=300\)
The value of \(_{14}C_2=91\)
\(=\frac{91}{300}\)
= 3/10 or 0.30
Thus, the probability that both students are girls is 3/10 or 0.30 if Mr. Garcia has 11 boys and 14 girls in his math class. He selects two students at random to demonstrate how they solved the day’s challenge assignment.
Learn more about the probability here:
https://brainly.com/question/11234923
a machine is designed to fill toothpaste tubes with 5.8 ounces of toothpaste. the manufacturer does not want any underfilling or overfilling. the correct hypotheses to be tested are
Answers
claim:- on average the machine is filled toothpaste tube is not equal to 5.8 ounces.
Hypothesis:-
\(H_{0}\): ∪= 5.8 vs \(H_{1}\) :∪≠5.8
\(H_{0}\): The machine fills toothpaste with an average of 5.8 ounces.
\(H_{1}\): The machine fills toothpaste with an average of not 5.8 ounces.
An explanation for a phenomenon is offered in the form of a hypothesis, or hypotheses. According to the scientific method, a hypothesis must be testable in order to qualify as a scientific hypothesis. Typically, scientists base their hypotheses on prior observations that leave room for doubt on the validity of the existing body of knowledge. A scientific theory is different from a scientific hypothesis, even though the terms "hypothesis" and "theory" are frequently used interchangeably. An educated guess or a notion is the starting point for a working hypothesis, which is an acknowledged hypothesis that is offered for further study.
In formal logic, the term "hypothesis" is used in a distinct sense to refer to the antecedent of a proposition; in the statement "If P, then Q," P refers to the hypothesis (or antecedent), and Q can be thought of as the consequent. In a (potentially counterfactual) What If inquiry, P is the underlying presumption.
To learn more about hypotheses visit here:
brainly.com/question/18064632
#SPJ4
Assume that a sample is used to estimate a population proportion p. Find the 99.9% confidence interval for a sample of size 176 with 118 successes. Enter your answer as an open-interval (i.e., parentheses) using decimals (not percents) accurate to three decimal places. 99.9% C.1. =
Answers
The 99.9% confidence interval for a sample of size 176 with 118 successes is (0.558, 0.778).
The formula for finding the confidence interval for a sample proportion is given as follows:
Confidence interval = sample proportion ± zα/2 * √(sample proportion * (1 - sample proportion) / n)
Where,
zα/2 is the z-value for the level of confidence α/2,
n is the sample size,
sample proportion = successes / n
Here, level of confidence, α = 99.9%, so α/2 = 0.4995. The value of zα/2 for 0.4995 can be found from the z-table or calculator and it comes out to be 3.291.
Putting all the values in the formula, we get:
Confidence interval = 0.670 ± 3.291 * √(0.670 * 0.330 / 176)
= (0.558, 0.778) (rounded to three decimal places and put in parentheses)
Thus, the 99.9% confidence interval for a sample of size 176 with 118 successes is (0.558, 0.778).
Know more about confidence interval here:
https://brainly.com/question/32546207
#SPJ11
What is the measure of the base of the rectangle if the area of the triangle is 28ft^2 ?
PLSSS ANSWER ASAP
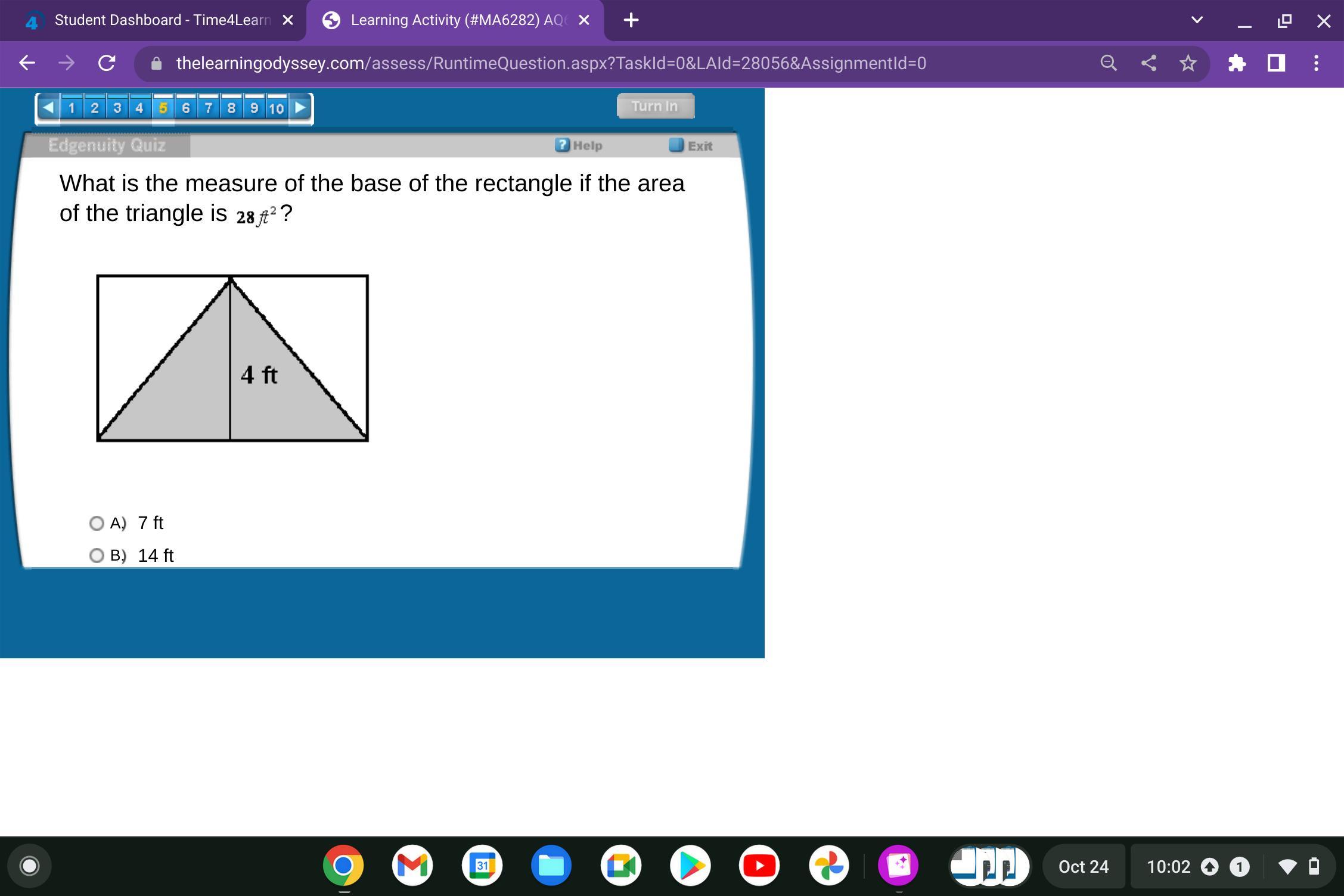
Answers
The measure of the base of the triangle is 14 ft.
How to find the base of a triangle?A triangle is a polygon with three sides. The sum of angles in a triangle is 180 degrees.
Therefore, the area of a triangle can be calculated as follows:
area of a triangle = 1 / 2 bh
where
b = baseh = heightTherefore,
area of the triangle = 28 ft²
height of the triangle = 4 ft
base of the triangle = ?
Hence,
area of the triangle = 1 / 2 × b × 4
28 = 1 / 2 × b × 4
28 = 4b / 2
28 = 2b
divide both sides by 2
b = 28 / 2
b = 14
Therefore,
base of the triangle = 14 ft
learn more on triangle here: https://brainly.com/question/117317
#SPJ1
The measure of the base of the triangle would be; 14 ft.
What is the triangle?A triangle is a polygon with three sides. The sum of angles in a triangle is 180 degrees.
Therefore, the area of a triangle can be calculated by the formula;
The area of a triangle = 1 / 2 b h
where
b = base
h = height
Thus,
The area of the triangle is 28 ft²
The height of the triangle = 4 ft
The base of the triangle =?
Hence,
Area = 1 / 2 × b × 4
28 = 1 / 2 × b × 4
28 = 4b / 2
28 = 2b
Then divide both sides by 2
b = 28 / 2
b = 14
Therefore, the base of the triangle is 14 ft
learn more about triangle here:
brainly.com/question/117317
#SPJ1
Find a function f such that f '(x) = 3x^3 and the line 81x + y = 0 is tangent to the graph of f.f(x) = 3x^4/4 + 32285040.75
Answers
The function f(x) = 3x^4/4 - 32285040.75 and the line 81x + y = 0 is tangent to the graph of f at the point (-3,0)
To find a function f such that f '(x) = 3x^3 and the line 81x + y = 0 is tangent to the graph of f, we can use the fact that the slope of the tangent line to a function f(x) at a point x = a is given by f'(a).
Since the line 81x + y = 0 has a slope of -81, we know that f'(a) = -81. So, we can set f'(a) = -81 and solve for a:
f'(a) = 3a^3 = -81
a^3 = -27
a = -3
Now we can use the point-slope form of a line to find the equation of the tangent line to the graph of f at x = -3:
y - f(-3) = f'(-3)(x + 3)
y - f(-3) = -81(x + 3)
We also know that the point (-3,0) lies on the graph of f and on the line 81x + y = 0. We can use this information to find the value of f(-3):
0 = -81(-3) + f(-3)
f(-3) = 243
So the equation of the tangent line is:
y - 243 = -81(x + 3)
y = -81x - 222
To find f(x) we can use the fact that f(x) = the antiderivative of f'(x)
∫f'(x)dx = f(x) + C
∫3x^3 dx = f(x) + C
3x^4/4 + C = f(x)
We can find the value of the constant C by using the point (x,y) = (-3,0) on the function f(x)
0 = 3(-3)^4/4 + C
C = -3^4*3/4 - 32285040.75
Therefore the function f(x) = 3x^4/4 - 32285040.75
And the line 81x + y = 0 is tangent to the graph of f at the point (-3,0)
Learn more about Calculus here: brainly.com/question/22810844
#SPJ4
\(\sqrt{2,25}.1,46-2,25.0,02\\\)
Answers
Answer:
i dont understand what your asking
Step-by-step explanation:
For the system [x = x(x+y-2) y' = y(3-x-3y) List all equilibria. the lines defined by x' = 0 or y' = 0
Answers
The equilibria for the system are (0, 0) and (3, 1).
To find the equilibria of the given system, we need to solve the equations x' = 0 and y' = 0 simultaneously. Let's start with x' = 0:
x(x + y - 2) = 0
This equation can be true if either x = 0 or x + y - 2 = 0.
Case 1: x = 0
Substituting x = 0 into the second equation, we get y' = y(3 - y). To find the equilibrium, we set y' = 0:
y(3 - y) = 0
This equation is true when either y = 0 or y = 3.
Case 2: x + y - 2 = 0
Substituting x + y - 2 = 0 into the second equation, we have y' = y(3 - (x + y - 2)). Simplifying further:
y' = y(3 - x - y + 2)
= y(5 - x - y)
To find the equilibrium, we set y' = 0:
y(5 - x - y) = 0
This equation is true when y = 0, y = 5 - x, or y = 0 and 5 - x = 0.
Combining the equilibria from both cases, we obtain the following equilibrium points: (0, 0) and (3, 1).
Learn more about: Equilibria
brainly.com/question/17408072
#SPJ11
A flower bed is in the shape of a triangle with one side twice the length of the shortest side and the third side is 18 feet more than the length of the shortest side. Find the dimensions if the perimeter is 186 feet.
Answers
Answer:
42 ft, 60 ft, 84 ft
Step-by-step explanation:
let x be the shortest side , then the other 2 sides are 2x and x + 18, so
x + 2x + x + 18 = 186 , that is
4x + 18 = 186 ( subtract 18 from both sides )
4x = 168 ( divide both sides by 4 )
x = 42
Then lengths of sides are
x = 42 ft
2x = 2 × 42 = 84 ft
x + 18 = 42 + 18 = 60 ft
what is 10x 10 x 10 x 10
Answers
Answer:
10^4 or 10000
Step-by-step explanation:
The multiplication of 10x 10 x 10 x 10 is 10000.
We have given that the
10x 10 x 10 x 10
We have to find the multiplication of the above 10.
What is multiplication?Multiplication is one of the four elementary mathematical operations of arithmetic, with the other ones being addition, subtraction, and division.
here we have
10 x10=100
10x100=1000
10x1000=10000
Therefore the multiplication of 10x 10 x 10 x 10 is 10000.
To learn more about the multiplication visit:
https://brainly.com/question/10873737
#SPJ2
choose ASA, SAA, or neither to describe this figure

Answers
ASA
Because the side between two equal angle is equal here. Because "The sides opposite to equal angles of a triangle are equal."
The graphs below have the same shape. What is the equation of the blue
graph?
f(x) = x²
5
g(x) = ?
g(x) =
A. g(x) = (x + 4)2 - 1
O B. g(x) = (x-4)2-1
ASAP plzzzz help

Answers
Answer:
The answer is g(x)=(x-2)^2+1
Step-by-step explanation:
The equation of the blue graph is y = (x - 4)² + 1.
Option D is the correct answer.
What is an equation?An equation contains one or more terms with variables connected by an equal sign.
Example:
2x + 4y = 9 is an equation.
2x = 8 is an equation.
We have,
The graphs of y = x² and y = (x - 4)² + 1 are both parabolas.
The graph of y = x² is a simple upward-facing parabola that passes through the origin.
As x increases or decreases, the value of y increases but at an increasingly slower rate until it reaches a minimum at x = 0, where it turns back upwards and continues to increase at an increasingly faster rate.
The graph of y = (x - 4)² + 1 is also a parabola, but it is shifted 4 units to the right and 1 unit up compared to y = x².
Thus,
The equation of the blue graph is y = (x - 4)² + 1.
Learn more about equations here:
https://brainly.com/question/17194269
#SPJ1
an estimate of 3 out of 25 people are left handed
Answers
find the minimum cost of a rectangular box of volume 170 cm3 whose top and bottom cost 8 cents per cm2 and whose sides cost 9 cents per cm2.
Answers
Therefore the minimum cost of a rectangular box of volume is x = 4.641588834 cents.
What is the volume of cuboid?A cuboid is a six-faced solid known as a hexahedron in geometry. Four-sided quadrilaterals make up its faces. A cuboid is a hexahedron, a solid with six faces, and the word "cuboid" means "like a cube" in the sense that a cuboid can become a cube by changing the length of its edges or the angle between its faces and edges. Four-sided quadrilaterals make up its faces. A cuboid can be made to look more like a cube by varying the lengths of its edges or the angles between its faces and edges. A cuboid's volume is equal to the sum of its length, breadth, and height. The opposite faces of a cuboid on a rectangular cuboid are equal, and all the angles are at right angles.
To know more about volume ,visit:
brainly.com/question/28770143
#SPJ4
Could Someone Help Me with this rewrite dis equation into y=mx+b
y< -3/4x+2
Answers
The equation in the form of y=mx+b will be y= -3/4x+2
What is a linear equation?It is defined as the relation between two variables, if we plot the graph of the linear equation we will get a straight line.
If in the linear equation, one variable is present, then the equation is known as the linear equation in one variable.
It is given that the inequality is y< -3/4x+2,
We have to write the inequality in the form of y=mx+b
A linear equation has the form y = mx + b in the slope-intercept format. X and Y are the variables in the equation. The values m and b represent the line's slope (m) and the value of y when x is 0.
\(\rm m =\dfrac{y_2-y_1}{x_2-x_1}\)
Thus, the equation in the form of y=mx+b will be y= -3/4x+2
Learn more about the linear equation here:
brainly.com/question/11897796
#SPJ1
A square has sides of length 6/12 inches. Area of length times width.
What is the area of the square in square inches?
Answers
The area of the square is 1/4inches² in square inches
What is area of squareThe area of a square is calculated by multiplying its two sides, that is area = s × s, where, 's' is one side of the square.
The square has side of length = 6/12
this can be simplified as 1/2
so
area of the square = (1/2 × 1/2) inches ²
area of the square = 1/4inches²
Thus, the area of the square is calculated using area = s × s, as 1/4inches²
Know about area of square here: https://brainly.com/question/24487155
#SPJ1
sin(20) = cos(2u) = tan(24) = 3. [-/5 Points] Use the given conditions to find the exact values of sin(2u), cos(2u), and tan(2u) using the double-angle formulas. sin(u) = -3/5, 3m/2
Answers
Using the given conditions that sin(20) = cos(2u) = tan(24) = 3, we can find the exact values of sin(2u), cos(2u), and tan(2u) using the double-angle formulas. By substituting the known values into the formulas, we can determine the exact values of these trigonometric functions.
Given sin(20) = 3, we can use the double-angle formula for sine to find sin(2u).
The double-angle formula for sine is sin(2u) = 2sin(u)cos(u). We know that sin(u) = -3/5, so we can substitute this value into the formula to calculate sin(2u).
Therefore, sin(2u) = 2(-3/5)(cos(u)).
Given cos(2u) = 3, we can use the double-angle formula for cosine to find cos(2u).
The double-angle formula for cosine is cos(2u) = cos^2(u) - sin^2(u). Since we already know sin(u) = -3/5 and cos(u) can be calculated using the Pythagorean identity (cos^2(u) = 1 - sin^2(u)), we can substitute these values into the formula to determine cos(2u).
Finally, given tan(24) = 3, we can use the double-angle formula for tangent to find tan(2u).
The double-angle formula for tangent is tan(2u) = (2tan(u))/(1 - tan^2(u)). By substituting the known value of tan(24) = 3 into the formula, we can calculate the exact value of tan(2u).
To learn more about trigonometric functions visit:
brainly.com/question/25618616
#SPJ11
Select all the statements that describe the distribution of the data

Answers
Add the expressions (5x2y3z)and(-7x2y3z).
A.-12x2y3z
B.-2x2y3z
C.2x2y3z
D.12x2y3z
Answers
Answer:
B
Step-by-step explanation:
A number when divided by 5 gives 0 as remainder, that number when divided by 7 gives 0 as remainder also. What will be the remainder when that number is divided by 35?
Answers
Answer:
zero
Step-by-step explanation:
The computation of the remainder when the number would be divided by 35
Let the number be x
So if x is divided by 5 gives the remainder 0
x = 0
And, if x is divided by 7 gives the remainder 0
x = 0
So if x is divided by 35 so the remainder is also zero
the ratio of men to women working for a company is to . if there are men working for the company, what is the total number of employees?
Answers
A ratio displays the multiplicity of two numbers, the total number of employees is 187.
Describe ratio.The ratio can be defined as the number that can be used to represent one quantity as a percentage of another. Only when the two numbers in a ratio have the same unit can they be compared.
How is ratio calculated?Ratios contrast two figures by ordinarily dividing them. A/B would be your formula if you were going to compare one data point (A) to another data point (B). This indicates that you are multiplying data A by data B.
So the ratios are-
men : women
4:7
x:119
Now, relationship between 7and 119 is -
men : women
4:7
7*17
x:119
Now,
men : women
4:7
4*17 :7*17
68:119
Total number
68+119= 187
To know more about ratio visit:
https://brainly.com/question/25184743
#SPJ4