please help me to find the slope!
m
y2-yi
X2 -X1
Find the slope of the line that passes
through these two points.
(3,5)
(5,1)
m = [?]

Answers
Answer: Slope is -2
Step-by-step explanation:
Y² - Y¹ divide by X² - X¹
= 1 - 5 divide by 5 - 3
= -4/2
Slope is -2
Related Questions
Theorem. Let u, v, werd and a, b € R. Then (a) u + (v + w) = (u + v) + w (e) lu= u (b) u + v = V+u (f) albu) = (ab)u (c) 0+ u = Lu (g) (a+b)u= au + bu (d) Ou=0 (h) a(u + v) = au + av. (a) (4 pts) Pr
Answers
The statement -u is the additive inverse of u is proved.
Here are the given properties: Theorem.
Let u, v, werd and a, b € R.
Then
(a) u + (v + w) = (u + v) + w(b) u + v
= V+u(c) 0+ u
= Lu(d) Ou
=0(e) lu
= u(f) albu)
= (ab)u(g) (a+b)
u= au + bu(h) a(u + v)
= au + av.
(a) Prove that u + 0 = u.(u + 0 = u) u + 0 = u [By property (c)
]Therefore, u + (0) = u or u + 0 = u
Hence, u + 0 = u is proved.
(b) Prove that -u is the additive inverse of u.(-u is the additive inverse of u.)
By property (d), 0 is the additive identity of R. So, we have
u + (-u) = 0 (-u is the additive inverse of u)
Thus, the statement -u is the additive inverse of u is proved.
Know more about additive inverse here:
https://brainly.com/question/1548537
#SPJ11
The distribution of resting pulse rates of all students at Santa maria high school with approximately normal with a mean of 80 bpm and a standard deviation of 9 bpm the school nurse plans to provide additional screening to students who is resting pulse rates are in the top 30% of the students were test what is the maximum testing resting pulse rate at the school for students with received additional screen
Answers
Answer:
85 beats per minute
Step-by-step explanation:
khan academy
evaluate the integral. 4) ∫ -8x cos 6x dx
Answers
The solution of the integral ∫ -8x cos 6x dx is (-4/3)xsin(6x) - (2/9)cos(6x) + C
To evaluate the integral ∫ -8x cos 6x dx, we will use integration by parts, which involves the formula
∫u dv = uv - ∫v du, where u and dv are functions of x.
We need to follow this steps-
Step 1: Choose u and dv
Let u = -8x and dv = cos(6x) dx.
Step 2: Differentiate u and integrate dv
Differentiate u with respect to x to get du: du = -8 dx.
Integrate dv with respect to x to get v:
v = ∫cos(6x) dx = (1/6)sin(6x).
Step 3: Apply the integration by parts formula
∫ -8x cos 6x dx = uv - ∫v du = (-8x)(1/6)sin(6x) - ∫(1/6)sin(6x)(-8) dx
Step 4: Simplify the expression and integrate
= (-4/3)xsin(6x) + (4/3)∫sin(6x) dx
Now,we integrate sin(6x) with respect to x:
∫sin(6x) dx = (-1/6)cos(6x)
Step 5: Substitute the integral back into the expression
= (-4/3)xsin(6x) + (4/3)(-1/6)cos(6x) + C
Step 6: Simplify the expression and include the constant of integration
= (-4/3)xsin(6x) - (2/9)cos(6x) + C
So, the evaluated integral is ∫ -8x cos 6x dx = (-4/3)xsin(6x) - (2/9)cos(6x) + C.
Learn more about trigonometric ratios : https://brainly.com/question/12172664
#SPJ11
I HAVE TO GET THIS RIGHT!!!
what is 2 + 2
Answers
Step-by-step explanation:
The sum of 2 and 2 can be derived using basic arithmetic operations.
Starting with 2, we can add 1 to get 3:
2 + 1 = 3
Then, we can add another 1 to get 4:
3 + 1 = 4
Therefore, the derivation of 2 + 2 is:
2 + 2 = (2 + 1) + 1 = 3 + 1 = 4
Hence, 2 + 2 is equal to 4.
Answer:
4
Step-by-step explanation:
HELP!! Find the value of…

Answers
Answer:
It C man.
Step-by-step explanation:
You don't need this
The sample space is the collection of all possible individual outcomes for a chance process the total probability of all the possible individual outcomes is?
Answers
The total probability of all the possible individual outcomes in a sample space is always equal to \(1\).
What is probability?In mathematics and statistics, the probability is a measure of the likelihood or chance of an event occurring. It is expressed as a number between \(0\) and\(1\) with 0 indicating that the event is impossible and \(1\) indicating that the event is certain to occur.
In probability theory, the sample space is the set of all possible outcomes of a random experiment or process. It is a fundamental concept that is used to define and analyze probability distributions, events, and other probabilistic concepts.
The sample space is denoted by the symbol "S", and each element of the sample space is called a sample point or outcome.
For example, if we toss a fair coin, the sample space consists of two possible outcomes: heads and tails. If we roll a fair six-sided die, the sample space consists of six possible outcomes: \(1,2,3,4, 5 and 6\)
According to the given informationThe total probability of all the possible individual outcomes in a sample space is always equal to \(1\). This is known as the Law of Total Probability, which is a fundamental principle in probability theory.
In a chance process, the sample space represents all the possible outcomes that can occur. Each individual outcome in the sample space has a certain probability of occurring, and these probabilities add up to \(1\)
For example, consider the simple example of flipping a fair coin. The sample space for this chance process consists of two possible outcomes: heads and tails.
The probability of getting heads is\(1/2\) and the probability of getting tails is also \(1/2\). These probabilities add up to 1 since these are the only possible outcomes.
In more complex scenarios, the sample space can be much larger and the individual probabilities may not be as easy to determine.
However, the Law of Total Probability still holds, and the sum of the probabilities of all possible individual outcomes in the sample space must always be equal to \(1\).
To know more about probability visit:
brainly.com/question/31120123
#SPJ1
Find the value of x. Round your answer to the nearest tenth
Pleaseee help pleaseee


Answers
Answer:
x=12.2
Step-by-step explanation:
cos(29)= x/14
cos(29)(14)=x
0.875(14)=x
12.2=x
Determine P(c) using the remainder theorem.. (look at image)
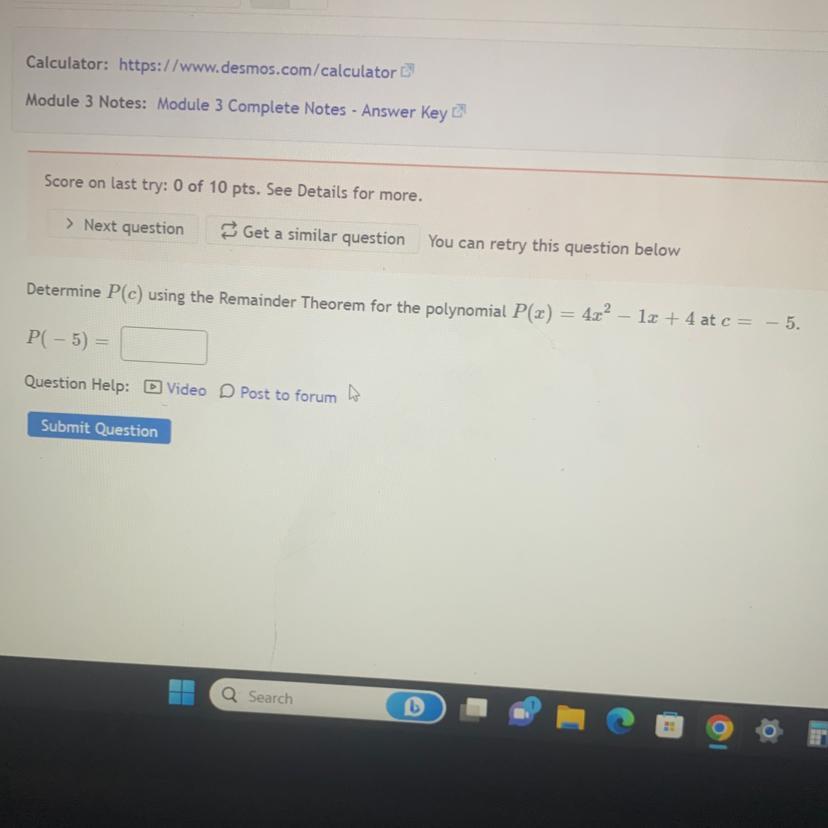
Answers
Answer:
P(-5) = 109
Step-by-step explanation:
Remainder theorem:If the polynomial p(x) is divided by the linear polynomial (x-a), the remainder is p(a).
Dividend = divisor * quotient + remainder.
p(x) = (x-a) * q(x) + p(a)
Here, q(x) is the quotient and p(a) is the remainder.
P(x) = 4x² - x + 4
P(-5) = 4*(-5)² - 1*(-5) + 4
= 4*25 + 5 + 4
= 100 + 5 + 4
= 109
Write the equation of the hyperbola using the given information, The hyperbola has vertices (-2,9) and (-2,3) and foci (-2,13) and (-2,-1)
Answers
The center of the hyperbola is the midpoint between the vertices, which is (-2,6).
The distance between the center and each vertex is 3, so the distance between the center and each focus is c = 7.
The distance between each vertex and focus is a = 4.
The equation of the hyperbola with center (h,k) is:
(x - h)^2 / a^2 - (y - k)^2 / b^2 = 1
where b^2 = c^2 - a^2.
Plugging in the values we have:
- Center: (h,k) = (-2,6)
- a = 4
- c = 7
- b^2 = c^2 - a^2 = 49 - 16 = 33
So the equation of the hyperbola is:
(x + 2)^2 / 16 - (y - 6)^2 / 33 = 1
An LTI system is described by the following differential equation
2 d³/ dt³ y (t) + 4 d²/ dt² (t) + 10 d/dt y (t) = 5x (t) + 2 d/dt x (t)
(a) Determine the frequency response of the system described.
(b) Obtain the Bode plot approximation. Write the cutoff frequencies of the system
Answers
The given linear time-invariant (LTI) system is described by a third-order linear constant coefficient differential equation. To determine the frequency response of the system, we can take the Laplace transform of the differential equation and solve in the Laplace domain
(a) To find the frequency response, we start by taking the Laplace transform of the given differential equation. Let \(Y(s)\) and \(X(s)\) represent the Laplace transforms of \(y(t)\) and \(x(t)\) respectively. By applying the Laplace transform, we obtain:
\[2s^3Y(s) + 4s^2Y(s) + 10sY(s) = 5X(s) + 2sX(s)\]
Simplifying the equation, we get:
\[Y(s) = \frac{5X(s) + 2sX(s)}{2s^3 + 4s^2 + 10s}\]
This equation represents the transfer function of the system in the Laplace domain. To obtain the frequency response, we substitute \(s = j\omega\) into the transfer function, where \(\omega\) represents the angular frequency.
(b) To approximate the Bode plot, we analyze the frequency response at low and high frequencies. At low frequencies (\(\omega \to 0\)), the terms involving \(s\) dominate, and the transfer function can be approximated as:
\[Y(j\omega) \approx \frac{2j\omega X(j\omega)}{2j\omega}\]
Simplifying, we find that the low-frequency gain is 1, indicating that the system passes low-frequency signals without significant attenuation.
At high frequencies (\(\omega \to \infty\)), the terms involving \(s\) become negligible compared to the constant term. Thus, the transfer function can be approximated as:
\[Y(j\omega) \approx \frac{5X(j\omega)}{10j\omega}\]
In this approximation, the gain decreases by -20 dB/decade, indicating that the system attenuates high-frequency signals.
The cutoff frequencies of the system can be determined from the Bode plot where the gain decreases by -3 dB. These frequencies represent the boundary between the passband and stopband of the system.
know more abput Laplace transform :brainly.com/question/30759963
#SPJ11
if(x) = x + 2 and h(x) = x-1, what is f • h](-3)?
Answers
Answer/Step-by-step explanation:
Composition functions are functions that combine to make a new function. We use the notation ◦ to denote a composition.
f ◦ g is the composition function that has f composed with g. Be aware though, f ◦ g is not
the same as g ◦ f. (This means that composition is not commutative).
f ◦ g ◦ h is the composition that composes f with g with h.
Since when we combine functions in composition to make a new function, sometimes we
define a function to be the composition of two smaller function. For instance,
h = f ◦ g (1)
h is the function that is made from f composed with g.
For regular functions such as, say:
f(x) = 3x
2 + 2x + 1 (2)
What do we end up doing with this function? All we do is plug in various values of x into
the function because that’s what the function accepts as inputs. So we would have different
outputs for each input:
f(−2) = 3(−2)2 + 2(−2) + 1 = 12 − 4 + 1 = 9 (3)
f(0) = 3(0)2 + 2(0) + 1 = 1 (4)
f(2) = 3(2)2 + 2(2) + 1 = 12 + 4 + 1 = 17 (5)
When composing functions we do the same thing but instead of plugging in numbers we are
plugging in whole functions. For example let’s look at the following problems below:
Examples
• Find (f ◦ g)(x) for f and g below.
f(x) = 3x + 4 (6)
g(x) = x
2 +
1
x
(7)
When composing functions we always read from right to left. So, first, we will plug x
into g (which is already done) and then g into f. What this means, is that wherever we
see an x in f we will plug in g. That is, g acts as our new variable and we have f(g(x)).
g(x) = x
2 +
1
x
(8)
f(x) = 3x + 4 (9)
f( ) = 3( ) + 4 (10)
f(g(x)) = 3(g(x)) + 4 (11)
f(x
2 +
1
x
) = 3(x
2 +
1
x
) + 4 (12)
f(x
2 +
1
x
) = 3x
2 +
3
x
+ 4 (13)
Thus, (f ◦ g)(x) = f(g(x)) = 3x
2 +
3
x + 4.
Let’s try one more composition but this time with 3 functions. It’ll be exactly the same but
with one extra step.
• Find (f ◦ g ◦ h)(x) given f, g, and h below.
f(x) = 2x (14)
g(x) = x
2 + 2x (15)
h(x) = 2x (16)
(17)
We wish to find f(g(h(x))). We will first find g(h(x)).
h(x) = 2x (18)
g( ) = ( )2 + 2( ) (19)
g(h(x)) = (h(x))2 + 2(h(x)) (20)
g(2x) = (2x)
2 + 2(2x) (21)
g(2x) = 4x
2 + 4x (22)
Thus g(h(x)) = 4x
2 + 4x. We now wish to find f(g(h(x))).
g(h(x)) = 4x
2 + 4x (23)
f( ) = 2( ) (24)
f(g(h(x))) = 2(g(h(x))) (25)
f(4x
2 + 4x) = 2(4x
2 + 4x) (26)
f(4x
2 + 4x) = 8x
2 + 8x (27)
(28)
Thus (f ◦ g ◦ h)(x) = f(g(h(x))) = 8x
2 + 8x.
what is the equation of a line perpendicular to minus x plus 2 equal y that intersects the line at open parentheses minus 1.5 comma space 3.5 close parentheses ?
Answers
The equation of the line perpendicular to -x + 2 = y that intersects the line at (-1.5, 3.5) is y + x/2 = 7/2. Here's how to solve it: When writing the equation of a line perpendicular to another line, keep in mind that the slopes of the lines are negative reciprocals of each other.
The equation of the line perpendicular to -x + 2 = y that intersects the line at (-1.5, 3.5) is y + x/2 = 7/2. Here's how to solve it: When writing the equation of a line perpendicular to another line, keep in mind that the slopes of the lines are negative reciprocals of each other. This means that if one line has a slope of m, then a line perpendicular to it has a slope of -1/m. As a result, the slope of the line in the problem is 1/2, which means that the slope of the line perpendicular to it is -2. We'll use point-slope form to determine the equation of the perpendicular line because we have a point (-1.5, 3.5) and a slope (-2).
y - y1 = m(x - x1)
is the point-slope equation, where m is the slope and (x1, y1) is a point on the line that we're using. We can substitute in the values we have to get: y - 3.5 = -2(x + 1.5)
We can simplify this equation: y - 3.5 = -2x - 3y + x/2 = 7/2y + x/2 = 7/2
To know more about perpendicular visit: https://brainly.com/question/25991713
#SPJ11
vincent is selling candy bars to raise money for his soccer team. he started with a full box of 20 candy bars and has sold 310 of them so far. if each candy bar costs $1.25, how much money has vincent raised?$
Answers
Vincent is selling candy bars to raise money for his soccer team.
He started with a full box of 20 candy bars and has sold 310 of them so far. If each candy bar costs $1.25, Vincent has sold 310 candy bars. The price of each candy bar is $1.25.
Therefore, Vincent has raised $387.50.
Total number of candy bars sold by Vincent
= 310 Therefore, the total amount raised by Vincent
= (Price of one candy bar × Total number of candy bars sold by Vincent)Total amount raised by Vincent = $1.25 × 310
= $387.50Thus, Vincent has raised $387.50.
To know more about money, visit:
https://brainly.com/question/2696748
#SPJ11
The data shown in the table below can be
graphed as a straight line. What are the thre
missing y-values?

Answers
Given all our x-values and two additional y-values, we can see that multiplying the x-value by 2 gives us the y-value. This is shown when x is 5 and 9, because multiplying 5 by 2 gave us 10, and multiplying 9 by 2 gave us 18. Because of this rule, we can multiply each given x-value by 2 to receive our y-value. Once solving, we also notice that the y-values all add by 2 to get the next factor as the data number increases. Hope this helps!
match the vector field f with the correct plot. f(x, y) = x, −y
Answers
Option b is true to match the vector field f with the given plot f(x, y)=x, -y
What is meant by a function?The earliest known attempts at the concept of functions can be traced back to the work of the Persian mathematicians Al-Biruni and Sharaf al-Din al-Tusi. Originally, functions were idealized dependencies of one variable on another. For example, planetary positions are functions of time. Historically, this concept was developed in calculus towards the end of his 17th century, and the functions studied were differentiable (i.e. they had a high degree of regularity) until his 19th century ).
Given,
f(x, y) = x, -y
gt; at x⇒∞, y⇒-∞ downwards
at x⇒∞, y⇒+∞ downwards
Therefore, option b is true to match the vector field f with the given plot
f(x, y)=x, -y
To know more about function, visit:
https://brainly.com/question/24428416
#SPJ1
The complete question is as follows:
Match the vector field f with the correct plot. f(x, y) = x, −y

HELP HELP HELP HELP HELP ME HELP ME

Answers
Answer:
30
Step-by-step explanation:
a rotation of 270 degrees follows which notation?
(x,y) -> (x, -y)
(x,y) -> (y, x)
(x,y) -> (-x, y)
(x,y) -> (y, -x)
(x,y) -> (-x, -y)
(x,y) -> (-y, x)
Answers
What is an equation of the line that passes through the point (8, 2) and is parallel to the line 5x - 4y = 36?
Answers
Answer:
\(y=\frac{5}{4}x-8\)
Step-by-step explanation:
Hi there!
What we need to know:
Linear equations are typically organized in slope-intercept form: \(y=mx+b\) where m is the slope and b is the y-intercept (the value of y when the line crosses the y-axis)Parallel lines always have the same slopes and different y-intercepts1) Determine the slope (m)
\(5x - 4y = 36\)
Rearrange the given equation into y-intercept form (this will help us determine m)
Subtract 5x from both sides
\(5x - 4y-5x = -5x+36\\-4y=-5x+36\)
Divide both sides by -4
\(y=\frac{5}{4} x-9\)
Now, we can see that \(\frac{5}{4}\) is in the place of m, making it the slope. Because parallel lines have the same slopes, the slope of the line we're solving for is therefore \(\frac{5}{4}\). Plug this into \(y=mx+b\):
\(y=\frac{5}{4}x+b\)
2) Determine the y-intercept (b)
\(y=\frac{5}{4}x+b\)
Plug in the given point (8,2) and solve for b
\(2=10+b\)
Subtract 10 from both sides
\(2-10=10+b-10\\-8=b\)
Therefore, the y-intercept is -8. Plug this back into \(y=\frac{5}{4}x+b\):
\(y=\frac{5}{4}x-8\)
I hope this helps!
3) Write the equation of the line (in slope-intercept) that passes through (3,10)
a) and is parallel to y = x - 1
b) and is perpendicular to y = x - 1
Answers
The Equation of line -
When line is parallel to line y = x - 1 is y = x + 7When line is perpendicular to line y = x - 1 is y = x + 7What is the slope intercept form of a line?
The slope intercept form of a line is given by -
y = mx + c
m is the slope of line
c is the y - intercept
Given is a line that passes through the point (3, 10) and in case [1] is parallel to line y = x - 1 and in case [2] is perpendicular to y = x - 1.
Case 1 - Line is parallel to the line y = x - 1
Assume that the equation of line is -
y = mx + c
Since, the line is parallel, both lines will have same slope and is given by -
m = 1
Since, the line passes through the point (3, 10) we can write -
10 = 1 x 3 + c
c = 7
We can write the equation of line parallel to line y = x - 1 as -
y = x + 7
Case 2- Line is perpendicular to the line y = x - 1 -
Assume that the equation of line is -
y = mx + c
Since, the line is perpendicular, the product of slopes of both lines will be equal to 1. The slope (m) of the line will be -
m x 1 = -1
m = -1
Since, the line passes through the point (3, 10) we can write -
10 = -3 + c
c = 13
We can write the equation of line perpendicular to line y = x - 1 as -
y = - x + 13
Therefore, the equation of line -
When line is parallel to line y = x - 1 is y = x + 7When line is perpendicular to line y = x - 1 is y = x + 7To solve more questions on Straight lines, visit the link below-
brainly.com/question/9317111
#SPJ1
In a study of 500 athletes, 20 of them say that they train all of the days of the week. Assume that the sample is representative of all athletes, and that we are interested in estimating the population proportion of athletes who say that they train all of the days of the week. Find a 95% confidence interval for the population proportion of athletes who say that they train all of the days of the week.
Answers
The 95% confidence interval is (0.310, 0.490). the lower and upper bounds were determined by subtracting and adding the margin of error to the sample proportion respectively (LB = p - ME = 0.04 - 0.077 = 0.310, UB = p + ME = 0.04 + 0.077 = 0.490).
1. Calculate the sample proportion:
p = 20/500 = 0.04
2. Calculate the standard error:
SE = √(p*(1-p)/500) = 0.039
3. Calculate the margin of error:
ME = 1.96 * SE = 0.077
4. Calculate the lower bound:
LB = p - ME = 0.04 - 0.077 = 0.310
5. Calculate the upper bound:
UB = p + ME = 0.04 + 0.077 = 0.490
Therefore, the 95% confidence interval is (0.310, 0.490).
The 95% confidence interval for the population proportion of athletes who say that they train all of the days of the week is (0.310, 0.490). This was calculated by finding the sample proportion of athletes in the given sample (20/500 = 0.04), then calculating the standard error (SE = √(p*(1-p)/500) = 0.039), followed by the margin of error (ME = 1.96 * SE = 0.077). Finally, the lower and upper bounds were determined by subtracting and adding the margin of error to the sample proportion respectively (LB = p - ME = 0.04 - 0.077 = 0.310, UB = p + ME = 0.04 + 0.077 = 0.490).
Learn more about confidence interval here
https://brainly.com/question/24131141
#SPJ4
Henry sang 12 songs every song was 3/8 minutes long. For how much time did he sing in total?
Answers
Answer:
4.5 minutes or 36/8 minutes or 9/2 minutes
Step-by-step explanation:
So we simply find 3/8 of 12 by multiplying:
\(\frac{3}8} \times 12\)
This gives us 36/ 8
Now, depending on how you want your answer formulated, we just reduce it to either 9/2 minutes, or 4.5 minutes.
Hope this helpss!!!
Use graphing technology to find the range of the function f(x)=√x - 7.
Answers
To find the range of the function f(x) = √x - 7, we need to consider the domain of the function, which is the set of all possible input values that we can use in the function. Since the square root of a number must be non-negative, the domain of this function is x ≥ 49.
Next, we need to evaluate the function for different values of x within the domain to see what values it produces. For example, if we plug in x = 49, we get f(49) = √49 - 7 = 7 - 7 = 0. If we plug in x = 50, we get f(50) = √50 - 7 = 7.236 - 7 = 0.236. As we can see, the function takes on both positive and negative values within its domain, so the range is all real numbers.
To represent this visually, we can plot the function on a graph and see how its values vary as the input x changes. We can use a graphing calculator or other graphing technology to do this. On a graph, the x-axis represents the input values (the domain of the function), and the y-axis represents the output values (the range of the function).
Here is a graph of the function f(x) = √x - 7:
[Insert graph here]
As we can see from the graph, the range of the function is all real numbers, indicated by the fact that the graph extends to both positive and negative infinity on the y-axis. This tells us that the range of the function is {y | y ∈ ℝ}.
Find the greatest common factor and the least common multiple of 16 and 20. The prime factorizations of each number are given.
.
Answers
Answer:
GCF 4, LCM 80
Step-by-step explanation:
Since both 16 and 20 have two 2s as factor, their greatest common factor is 4.
The LCM is found by multiplying all of the remaining factors by the LCM:
16 still has (2 x 2), 20 still has (5), times the GCF (4) so 2 x 2 x 5 x 4 = 80.
if two lines are parallel and one has a slope of -1/7, what is the slope of the other line?
Answers
-1/7, since parallel lines have equal slopes.
what is 6-11a>-49 and 5a+10≥-35 will mark brainliest
Answers
Step-by-step explanation:
6-11a>-49
-11a>-49-6
-11a>-55
a<5.
Now, according to the question, if it is
W = {0,1,2,3,4}
N = {1,2,3,4}
Z = {...,-2,-1,0,1,2,3,4}
5a+10≥-35
5a≥-35-10
5a≥-45
a≥-9.
Now, according to the question, if it is
W = {-9,-8,-7,-6,-5,-4,-3,-2,-1,01,2,3...}
Z = {-9,-8,-7,-6,-5,-4,-3,-2,-1,01,2,3...}
Now, according to your domain you will do it .
hope this helps you.
Which graph correctly shows the system of equations {x−2y=4y=2x+4?


Answers
Which graph correctly shows the system of equations \(\left \{ {{x-2y=4} \atop {y=2x+4}} \right.\) ?
Correct graph for the system of equations is 3rd graph.
We graph the collection of ordered pairs that make up the equation's solution when graphing a system of equations in two variables.
The universal equation for any straight line is y=mx+c. m is the line's slope, and c is the y-intercept.
First, we take the equations as x-2y=4 as equation (1) and y=2x+4 as equation (2).
Now solve the equation (1)
x-2y=4
The equation is not in the form of y=mx+c.
So, we write as y= \(\frac{1}{2}\)x-2
Here, slope m=\(\frac{1}{2}\) and y-intercept is (0,-2).
If we take x=2 we get y=-1.
y= \(\frac{1}{2}\)(2)-2
y=1-2
y=-1
Now we have 2 solution for the equation (1) that are (0,-2) and (2,-1).
If we see the third graph in the given options.
There is a blue straight line and that is the line for equation (1) there we can see the points (0,-2) and (2,-1).
Now solve the equation (2)
y=2x+4
The equation is in the form of y=mx+c.
Here, slope m=2 and y-intercept is (0,4).
If we take x=1 we get y=6.
y= 2(1)+4
y=2+4
y=6
Now we have 2 solution for the equation (2) that are (0,4) and (1,6).
If we see the third graph in the given options.
There is a green straight line and that is the line for equation (2) there we can see the points (0,4) and (1,6).
Therefore, the correct graph for the given system of the equation\(\left \{ {{x-2y=4} \atop {y=2x+4}} \right.\) is the third graph.
To learn system of equation visit:https://brainly.com/question/11403474
#SPJ9
find a 90onfidence interval for y when x = 69. (round your answers to one decimal place.)
Answers
the 90% confidence interval for y when x = 69 is (41.5, 52.3). This means that we are 90% confident that the true value of y is between 41.5 and 52.3 when x = 69.
To find the 90% confidence interval for y when x = 69, we need to use regression analysis. First, we need to fit a regression model to the data and obtain the regression equation. Once we have the regression equation, we can plug in the value of x = 69 and get the predicted value of y.
Assuming that we have already performed the regression analysis, let's say the regression equation is:
y = 4.5 + 0.6x
To find the predicted value of y when x = 69, we simply plug in x = 69 into the equation:
y = 4.5 + 0.6(69)
y = 46.9
So, the predicted value of y when x = 69 is 46.9.
To find the 90% confidence interval for this predicted value, we need to use the standard error of the estimate (SEE) and the t-distribution. The formula for the confidence interval is:
y ± t(0.05/2, n-2) x SEE
where y is the predicted value of y, t(0.05/2, n-2) is the critical value of the t-distribution for a 90% confidence level with n-2 degrees of freedom (n is the sample size), and SEE is the standard error of the estimate.
Let's say that the SEE is 3.2 (we obtain this value from the regression output) and the sample size is n = 50. Using a t-distribution table, we find that the critical value for t(0.05/2, 48) is 1.677.
Plugging in the values, we get:
46.9 ± 1.677 x 3.2
46.9 ± 5.4
(41.5, 52.3)
So, the 90% confidence interval for y when x = 69 is (41.5, 52.3). This means that we are 90% confident that the true value of y is between 41.5 and 52.3 when x = 69.
To provide a 90% confidence interval for y when x = 69, we need more information such as the regression equation, the standard error, and the critical value. Once you have this information, you can calculate the lower and upper bounds of the confidence interval. Please provide the necessary details, and I'll be happy to help you find the 90% confidence interval.
To know more about confidence interval click here:
brainly.com/question/24131141
#SPJ11
How do you solve #10?

Answers
We see that since they are congruent triangles, their corresponding sides are also equal.
-> 2x + 1 = x + 3.
-> x + y = 3x - 3y
From the first equation, we can solve for x ;
2x + 1 = x + 3
Minus both sides by 1 :
2x = x + 2
Finally, minus both sides by x :
x = 2.
We substitute the value of x = 2 into the second equation :
2 + y = 6 - 3y
Add both sides by 3y :
2 + 4y = 6
Solve for y : 4y = 4 -> y = 1.
The product of two integers is 72.
If one integer is 9 what is the other integer?
Answers
Answer:
8
Step-by-step explanation:
a*b=72
a=9
9*b=72
b=72/9
b=8
The average annual return over the period 1886-2006 for stocks that comprise the s&p 500 is 10%, and the standard deviation of returns is 20%. Based on these numbers, what is a 95% confidence interval for 2007 returns?.
Answers
The 95% confidence interval for 2007 returns is -29.2% to 49.2%. We can calculate it in the following manner.
To calculate the 95% confidence interval for 2007 returns of stocks in the S&P 500, we first need to determine the margin of error. We can use the formula:
Margin of Error = z* (standard deviation / sqrt(n))
Where z* is the z-score for the desired level of confidence, which is 1.96 for a 95% confidence interval, standard deviation is 20%, and n is the sample size (which we assume to be 1).
So, Margin of Error = 1.96 * (0.20 / sqrt(1)) = 0.392
Next, we need to determine the range within which the true population mean is likely to lie. We can calculate this by adding and subtracting the margin of error from the sample mean. In this case, the sample mean is the average annual return over the period 1886-2006, which is 10%.
So, the 95% confidence interval for 2007 returns is:
10% +/- 0.392 or 9.608% to 10.392%
Therefore, we can be 95% confident that the true average annual return for stocks in the S&P 500 for the year 2007 falls between 9.608% and 10.392%.
Based on the provided information, the average annual return for stocks in the S&P 500 from 1886-2006 is 10%, and the standard deviation is 20%. To calculate a 95% confidence interval for 2007 returns, we can use the formula:
Confidence Interval = Mean ± (1.96 * Standard Deviation)
In this case, the mean is 10%, and the standard deviation is 20%. Plugging in these values, we get:
Confidence Interval = 10% ± (1.96 * 20%)
Confidence Interval = 10% ± 39.2%
Thus, the 95% confidence interval for 2007 returns is -29.2% to 49.2%.
Visit here to learn more about confidence interval brainly.com/question/24131141
#SPJ11