< 2 and <3 are ____
angles. (1 point)
A.complementary
B.Overtical
C.right
D.supplementary

Answers
Answer:
D. l hope this helps
Step-by-step explanation:
Answer:
d. supplementary angles
Step-by-step explanation:
Supplementary angles are angles that add up to 180. Both <2 and <3 combined show a straight 180 line, so this is the best option. Let me know if that helps! :)
Related Questions
Kate can read 12 pages in 8 minutes. How many minutes will it take her to read 30 pages? Write a proportion to this problem.
Answers
Answer:
12/8 = 30/x ... x =20minutes
Step-by-step explanation:
Which statement about equations with an infinite number of solutions is TRUE? No matter what number you choose to substitute for the variable, the result will be a true statement. No matter what number you choose to substitute for the variable, the result will always be 0=1 . No matter what number you choose to substitute for the variable, the result will always be u=0 . No matter what number you choose to substitute for the variable, the result will be a false statement.
Answers
Correct Option is No matter what number you choose to substitute for the variable, the result will be a true statement.
What are Infinite Solutions?The number of solutions of an equation based on the total number of variables contained in it. As a result, the equation system consists of two or more equations with two or more variables.
It can be any combination such as
2 equations in 3 variables5 equations in 3 variables, etcThere are three different forms of equation solutions, depending on the quantity of variables and equations. They are
Unique Solution (One solution)No solutionInfinite Solutions (Many solutions)The term “infinite” represents limitless or unboundedness.
For the infinite number of solution of the equation No matter what number you choose to substitute for the variable, the result will be a true statement.
To know more about variable, visit:
https://brainly.com/question/15078630
#SPJ1
1) Which is greater, 75 % of 85 or 85% of 75? Explain and show how you know.
7
Answers
Answer:
They are both equal!
Step-by-step explanation:
To find the answers, do the following
For 75% of 85 multiply 0.75 by 85:
(0.75)(85) = 63.75
For 85% of 75 multiply 0.85 by 75:
(0.85)(75) = 63.75
So, both of them are equal to each other.
HOWEVER, there is thing with percents that the opposites equal each other. So, you see how the percent and the whole number is changed with the equations? Like 85 turns to 85% and whatnot. If the percent/whole number of something is reversed it will equal the same thing.
So, you don't even need to do all the math to infer that they would be the same number. Changing the percent to the other number will yield the same result.
I hope that makes sense, ask questions if you need!!
the following are the populations for the top twelve largest cities in the u. s. New York city, NY: 8,336,817 Los Angeles ca: 979.576 Chicago, il:2,693,976 Houston, Tx: 2,320,268 phoenix Az: 1,680,992 Philadelphia pa: 1,584,064 San Antonio Tx: 1, 547,253 San Diego ca: 1,423,851 Dallas Tx: 1,343,573 San Jose ca: 1,021,795 Austyn tx:978,908 Jacksonville Fl: 911,507. what is the mean population?, what is the median population?, what is the mode?, what is range?, what is the standard deviation?I need the standard deviation, I already did mode, mean, range, take the value of the population of each city, subtract the mode raised to 2, it does not give me the result
Answers
The mean is 2,071,798.33
The Median is 1,485,552
The range is 7,425,310
The standard deviation is given as 1961105.68
The mean population can be computed as:Mean = (Sum of all populations) / (Number of cities)
= (8,336,817 + 979,576 + 2,693,976 + 2,320,268 + 1,680,992 + 1,584,064 + 1,547,253 + 1,423,851 + 1,343,573 + 1,021,795 + 978,908 + 911,507) / 12
= 24,861,580 / 12
= 2,071,798.33
To find the median, we need to arrange the populations in increasing order and find the middle value. If we arrange these values, we get:
911,507, 978,908, 979,576, 1,021,795, 1,343,573, 1,423,851, 1,547,253, 1,584,064, 1,680,992, 2,320,268, 2,693,976, 8,336,817
Since there are 12 cities (an even number), the median would be the average of the 6th and 7th values:
Median = (1,423,851 + 1,547,253) / 2
= 1,485,552
The mode isn't applicable here as no city population repeats.
The range is the highest population minus the lowest population:
Range = 8,336,817 - 911,507
= 7,425,310
Standard deviation = (911507- 2068548.3333333)² + (978908 - 2068548.3333333)² + (979576 - 2068548.3333333)² + ( 1021795 - 2068548.3333333)² + (1343573- 2068548.3333333)² +( 1423851- 2068548.3333333)² + (1547253- 2068548.3333333)² +( 1584064- 2068548.3333333)² + (1680992- 2068548.3333333)² + ( 2320268- 2068548.3333333)² +(2693976- 2068548.3333333)² +( 8336817 - 2068548.3333333)² / 12
= 46151226311869 / 12
= 3845935525989.1
\(standard deviation = \sqrt{ 3845935525989.1}\)
= 1961105.6896529
Read more on mean , mode and median here https://brainly.com/question/14532771
#SPJ1
What is the product of Three-fourths and Negative StartFraction 6 over 7 EndFraction?
Answers
Answer:
-9/14
Step-by-step explanation:
product of Three-fourths and Negative StartFraction 6 over 7 EndFraction
\( = \frac{3}{4} \times \bigg( - \frac{6}{7} \bigg) \\ \\ = \frac{3 \times ( - 6)}{4 \times 7} \\ \\ = - \frac{18}{28} \\ \\ = - \frac{9}{14} \\ \)
Answer:
-9/14 so the answer is B
Step-by-step explanation:
Hope this helps
the circumference of a circular patio is 53 2/7 ft. what is the area of the patio. use 22/7 for pi. round to the nearest hundredth
Answers
Answer:
I think it is A≈225.77
Step-by-step explanation:
Five more than the product of a number and six is equal to nine
Answers
Answer:
Think about the PEMDAS rules for order of operations.
Multiplication comes before addition or subtraction, so first we have "the product of a number and 5" - that's 5x. Then "six more" than that would be 5x + 6. "Equals" means the "=" sign, so the final answer is 5x + 6 = 8.
What is the value of x?
07
0 7√2
O
O 14
O 14√/2
45°
7√2

Answers
The value of the side length x is equal to 14 using the trigonometric ratio of sine.
What is trigonometric ratios?The trigonometric ratios is concerned with the relationship of an angle of a right-angled triangle to ratios of two side lengths.
The basic trigonometric ratios includes;
sine, cosine and tangent.
sin45 = 7√2/x {opposite/hypotenuse}
√2/2 = 7√2/x {sin45 = √2/2}
x = 7√2 × 2/√2 {cross multiplication}
x = 7 × 2
x = 14
Therefore, the value of the side length x is equal to 14 using the trigonometric ratio of sine.
Read more about trigonometric ratios here: https://brainly.com/question/3457795
#SPJ1
2. How much interest is earned by investing $2100 for three years at 4.5% compounded
monthly?
Answers
Answer:
The final balance is $2,402.92.
The total compound interest is $302.92.
Step-by-step explanation:
A = P(1 + r/n)^{nt}
A = final amount
P = initial principal balance
r = interest rate
n = number of times interest applied per time period
t = number of time periods elapsed
A=2100(1+.045/12)^(3*12)
A=2100(1.00375)^(36)
A=2100*1.442478322
A=2402.92
gain of 302.92
What is the shape of each cut surface? What are its dimensions?
Answers
Answer:
1. The shape of each cut surface is the same shape as the base it is parallel to: a rectangle. It is an 8" by 10" rectangle
Step-by-step explanation:
An item is regularly priced at $31. Teresa bought it at a discount of 85% off the regular price. Use the ALEKS calculator to find how much Teresa paid.

Answers
which describes the correct order of steps for constructing an angle bisector of ABC using only a straightedge and compass
Answers
Constructing an angle bisector using only a straightedge and compass involves drawing the Angle, creating two arcs that intersect the angle, and using those arcs to create a line segment that bisects the angle.
To construct an angle bisector using a straightedge and compass, there are several steps that you need to follow. The correct order of steps for constructing an angle bisector of ABC using only a straightedge and compass are listed below:
Step 1: Draw the angle ABC with a straightedge.
Step 2: Place the point of the compass on point B and swing an arc that intersects both lines that make up the angle. Label the two points where the arc intersects the angle as points D and E.
Step 3: Without changing the compass width, place the point of the compass on point D and swing an arc that intersects the ray that extends from point B. Label the point of intersection as point F.
Step 4: Without changing the compass width, place the point of the compass on point E and swing an arc that intersects the ray that extends from point B. Label the point of intersection as point G.
Step 5: Draw the line segment FG with a straightedge. This line segment is the angle bisector of angle ABC.
In summary, constructing an angle bisector using only a straightedge and compass involves drawing the angle, creating two arcs that intersect the angle, and using those arcs to create a line segment that bisects the angle. Following these steps will allow you to construct the angle bisector of ABC accurately.
For more questions on Angle.
https://brainly.com/question/31615777
#SPJ8
rounded to the nearest whole, what is the radius length if minor arcYZ = 12 and angleYXZ is one-third of a full circle? (i guessed it idk if it’s right)

Answers
Answer:
Option (1)
Step-by-step explanation:
Since the length of arc YZ = 12 units
m∠YXZ = one third of the full circle = \(\frac{360}{3}\) = 120°
From the formula of arc length,
Length of arc = \(\frac{\theta}{360}(2\pi r)\)
Where θ = Central angle subtended by the arc
r = radius of the circle
By substituting these values in the formula,
12 = \(\frac{120}{360}(2\pi r)\)
12 = \(\frac{2}{3}\pi r\)
\(18=\pi r\)
r = \(\frac{18}{\pi }\)
r = 5.73
r ≈ 6 units
Therefore, Option (1) will be the answer.
Which is the graph of f(x) = 2 Square root negative X
Answers
Answer:
the graph is (0,2)
Step-by-step explanation:
A closed rectangular tank has a length of 8.5 feet, a width of 3.2 feet, and a height of 4.8 feet. Find the surface area of the tank.
Answers
Answer: 166.72 \(ft^{2}\)
Step-by-step explanation: math
Step-by-step explanation:
All you have to do here is use the surface area, or SA, equation.
Sa=2lw+2lh+2hw
sa=2(8.5)(3.2)+2(8.5)(4.8)+2(4.8)(3.2)
Sa=54.4+81.6+30.72
Sa=166.72
Hope this helped!
Which of the following effects of a BAC of .2 percent does NOT relate to one’s driving ability?
A. lack of coordination
B. Breathalyzer test results indicating a high BAC
C. Impaired gross motor skills such as walking or gestures
D. Impaired reactions
It is either between B or C.
Answers
The effect that does NOT relate to one's driving ability is breathalyzer test results indicating a high BAC. Option B.
Breathalyzer testWhile the breathalyzer test measures the blood alcohol concentration (BAC), it is a method used to determine the level of alcohol in a person's system and is commonly used in assessing impairment for driving under the influence.
Therefore, it directly relates to one's driving ability and is not excluded from the effects on driving ability caused by a BAC of .2 percent.
On the other hand, options, lack of coordination, impaired gross motor skills, and impaired reactions all directly relate to one's driving ability as they affect the physical and cognitive abilities necessary for safe and efficient driving.
More on breathalyzers can be found here: https://brainly.com/question/26086547
#SPJ1
replace the letters a,b, and c with the numbers 3, 4, and 5 to make a true statement, 2a+2a=bc
Answers
Answer:
FALSEStep-by-step explanation:
In this problem, we are expected to provide an outcome to a true statement that the left-hand side of the equation is the same as the right-hand side
2a+2a=bc
Given that
a= 3,
b= 4,
c= 5.
We can substitute these values for a, b, and c in the given expression to see if the LHS and the RHS of the given expression are equal
2(3)+2(3)=4*5
6+6=20
12=20
The answer is FALSE because 12 is not equal to 20
The ages of three siblings are all consecutive integers. The sum of their ages is 39. How old is the youngest sibling equation: Solution: years old. equation
Answers
Answer:
12,13,14
Step-by-step explanation:
Consecutive means something like 1,2,3 or 43,44,45.
So we know the siblings age is something like that.
We start from the youngest, and let's say the youngest's age is x.
Remember consecutive? So the three siblings' age would be x, x+1, x+2 respectively. We can prove this is consecutive by replacing x with a random number, say, 2. That would make x, x+1, x+2 become 2, 2+1, 2+2, which is 2,3,4.
Sum of their age is 39:
Adding x, x+1, x+2 together is 39, so x + x+1 + x+2 = 39
We can further simplify x + x+1 + x+2 = 39 into
3x + 3 = 39
3x = 39-3
3x= 36
x=36÷3
x=12
Remember that x is the youngest age? So naturally the three siblings' ages are 12, 13 and 14 respectively.
Explain how to rewrite the function shown in order to determine the transformation of the parent function. Then, describe the transformation of the graph compared to the parent function. y = ^3√-8x_4
Answers
The parent function is y = ∛x and the sequence of transformation is
Shift the function up by 4 unitsReflect across the y-axisHorizontal stretch by 1/8How to rewrite the equation of the functionFrom the question, we have the following parameters that can be used in our computation:
y = ^3√-8x_4
Rewrite the above equation properly
So, we have the following representation
y = ∛-8x - 4
Start by shifting the function up by 4 units
So, we have the following representation
y = ∛-8x - 4 + 4
Evaluate the sum
y = ∛-8x
Reflect across the y-axis
So, we have
y = ∛8x
Horizontal stretch by 1/8
This is represented as
y = ∛1/8 * 8x
So, we have
y = ∛x
Hence, the parent function is y = ∛x
Read more about transformation at
https://brainly.com/question/27224272
#SPJ1
Answer:Rewrite the equation by factoring –8 from the radicand and taking the cube root to get –2 in front of the radical symbol.
The graph is reflected over the x-axis.
The graph is also reflected over the y-axis.
The graph is vertically stretched by a factor of 2.
The graph is translated ½ unit to the left.
Step-by-step explanation:
Angle ADB and CD are straight lines. angle ADC = 5 x angle CDB Work out the size of angle ADC.

Answers
Answer:
Step-by-step explanation:

Find the average rate of change of the function below over the interval (-4,-1).

Answers
Answer:
-2
Step-by-step explanation:
\(\frac{f(b)-f(a)}{b-a}\\ \\=\frac{f(-1)-f(-4)}{-1-(-4)}\\ \\=\frac{-4-2}{-1+4}\\ \\=\frac{-6}{3}\\ \\=-2\)
Therefore, the average rate of change over the interval [-4,-1] is -2.
Jack wants to abopt all kittens that weigh more than 4 ounces how many kittens will he abopt
Answers
Adopt that many kittens
someone please help me
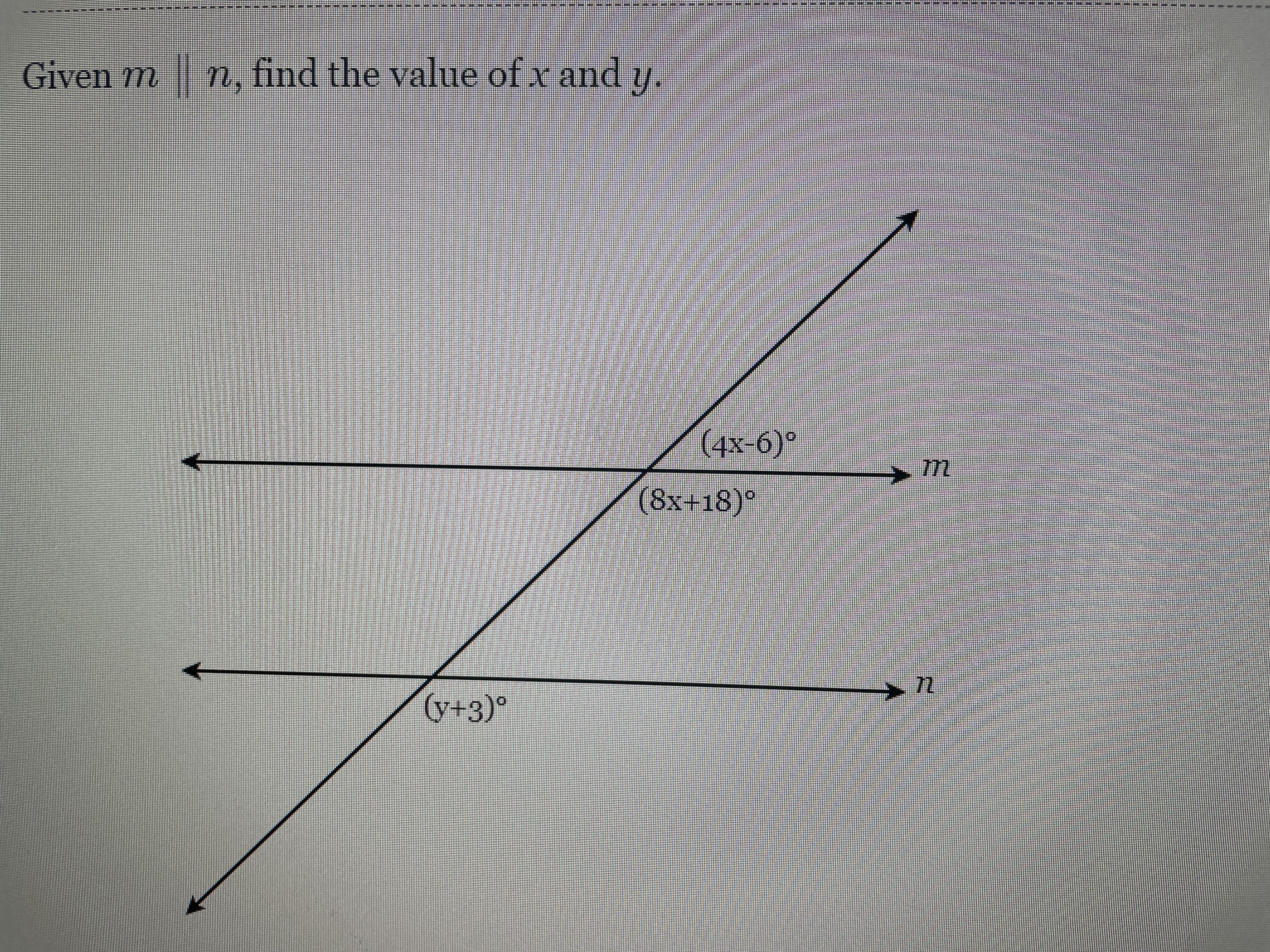
Answers
Answer:
x = 14°, y = 127°
Step-by-step explanation:
Definitions:Parallel lines: Two lines are considered as parallel if, and only if, they are coplanar and do not intersect each other.
Linear pair: Two adjacent angles whose opposite rays comprise of their noncommon side. These angles are also supplements; hence, the sum of their measures equal 180°.
Corresponding angles: Two angles with corresponding or relative positions (same side along the transversal that are non-adjacent).
Corresponding Angles Theorem: if there are two lines cut by a transversal, then their corresponding angles are congruent.
Solution:Given that m ║n, and that we are asked to find the value of x and y:
Solve for x:As defined in the previous section, a linear pair are two adjacent angles that are supplements. Therefore, ∠(4x - 6)° and ∠(8x + 18)° are linear pair.
We can establish the following equation to solve for the value of x (by definition of linear pair ):
⇒ ∠(4x - 6)° + ∠(8x + 18)° = 180°
4x° - 6° + 8x° + 18° = 180°
Combine like terms and constants:
12x° + 12° = 180°
Subtract 12° from both sides:
12x° + 12° - 12° = 180° - 12°
12x° = 168°
Divide both sides by 12 to solve for x:
\(\displaystyle\mathsf{\frac{12x^{\circ}}{12^{\circ}}\:=\:\frac{168^{\circ}}{12^{\circ}} }\)
x = 14°
Therefore, the value of x = 14°.
Solve for y:As defined in the previous section of this post, corresponding angles are located in relative positions in between the intersection of the parallel lines and transversal. Moreover, according to the Corresponding Angles Theorem, corresponding angles are congruent.
⇒ Corresponding angles: ∠(8x + 18)° ≅ ∠(y + 3)°
Since these two angles are congruent, we can set up the following equality statement: ∠(8x + 18)° = ∠(y + 3)°.
Substitute the value of x = 14° into the equality statement to solve for y:
∠(8x + 18)° = ∠(y + 3)°
8(14)° + 18° = y° + 3°
112° + 18° = y° + 3°
130° = y° + 3°
Subtract 3° from both sides of the equation:
130° - 3° = y° + 3° - 3°
127° = y
Double-check Solution:Linear pair: ∠(4x - 6)° + ∠(8x + 18)° = 180°
4(14)° - 6° + 8(14)° + 18° = 180°
56° - 6° + 112° + 18° = 180°
180° = 180° (True statement).
Corresponding Angles: ∠(8x + 18)° = ∠(y + 3)°
8(14)° + 18° = 127° + 3°
112° + 18° = 130°
130° = 130° (True statement).
Therefore, x = 14° and y = 127°.
_________________________
Keywords:
Linear pair
Corresponding Angles
Supplements
Parallel lines
Transversal
_____________________________
Learn more about this topic here:
https://brainly.com/question/26167358
2.) A shop sells oranges at 6 for N100. A trader sells the same kind of oranges at 8 for N120.
Which price is cheaper, by how much per orange?
Answers
The cheaper price among this set of orange sales is the one done by trader B, who sells 8 for N120.
How is the cheaper price determined?The cheaper price can be computed by finding out the price per unit.
Data and Calculations:Price of Set Unit Price
Sale of 6 oranges N100 N16.67 (N100/6)
Sale of 8 oranges N120 N15.00 (N120/8)
Thus, selling 8 oranges for N120 is cheaper than selling 6 oranges for N100, and N1.67.
Learn more about establishing prices at https://brainly.com/question/1153322
#SPJ1
I’m in AP Calculus AB. Can you help me solve this showing steps?I’m not exactly sure how to do this.

Answers
We need to find the derivative of a function, but if we look at the function is a quotient. Then we can use the rule for the derivative of a quotient:
\(\begin{gathered} f(x)=\frac{g(x)}{h(x)} \\ \text{Then,} \\ \frac{df}{dx}=\frac{g^{\prime}h-h^{\prime}g}{h^2} \end{gathered}\)In this case, we have:
\(f(x)=\frac{\csc (x)}{x^2}\)We can call:
\(\begin{gathered} g(x)=\csc (x) \\ h(x)=x^2 \end{gathered}\)Now we apply the rule:
\(\frac{df}{dx}=\frac{\frac{dg}{dx}h-\frac{dh}{dx}g}{h^2}\)We know that the derivative of h(x) = x^2 is 2x, and the derivative of g(x) = csc(x) is [-cot(x)csc(x)]
Replacing the values:
\(\frac{df}{dx}=\frac{-\cot (x)\csc (x)\cdot x^2-2x\cdot\csc (x)}{(x^2)^2}\)Simplifying:
We can factor out csc(x)*x in the numerator and solve the square in the denominator
\(\frac{df}{dx}=\frac{x\csc (x)(-x\cot (x)-2)}{x^4}\)Finally, we can factor the (-1) inside the parentheses and cancel out the x in the numerator:
\(\frac{df}{dx}=-\frac{\csc (x)(x\cot (x)+2)}{x^3}\)And this is the final answer.
What is the meaning of "\( \varphi (x,y)\) be \( y\wedge \phi (x)\) "?
![What is the meaning of "[tex] \varphi (x,y)[/tex] be [tex] y\wedge \phi (x)[/tex] "?](https://i5t5.c14.e2-1.dev/h-images-qa/contents/attachments/qBCO5ypO9m13MpdV66LMvZB6FUp8SIQM.png)
Answers
The reasoning presented lacks explicit explanations and logical connections between the steps, making it difficult to fully understand the intended proof strategy.
The given proof aims to show that the Separation Axioms can be derived from the Replacement Schema using a particular construction involving a formula p(x, y). Let's analyze the proof step by step:
Define the formula p(x, y) as x = yo(x).
This formula states that for each x, y pair, x is equal to the unique object y such that y is obtained by applying the operation o to x.
Define the set F as {(x, x) (x)}.
This set F contains pairs (x, x) where x is the unique object obtained by applying the operation (x) to x.
Claim: F(X) = {y (x = X)p(x, y)} = {y: (x = X)x = y^o(x)} = {x: (3x € X)o(x)} = {x X: (x)}.
This claim asserts that F(X) is equivalent to {y (x = X)p(x, y)}, which is further equivalent to {y: (x = X)x = y^o(x)}, and so on.
The proof states that since (x, y) satisfies the functional formula VaVyVz(p(x, y)^(x, z) y = z), it follows that (x, y) is a functional formula.This step emphasizes that the formula p(x, y) satisfies certain properties that make it a functional formula, which is relevant for the subsequent deductions.
Finally, the proof concludes that the Separation Axioms follow from the Replacement Schema, based on the previous steps.
For more such questions on explicit
https://brainly.com/question/30394385
#SPJ8
The symbol ⊕ is used to denote exclusive or, so p ⊕ q ≡ (p v q) ^ ~ (p ^ q).
a) simplify the statement p ⊕ p.
b) Show that p ⊕ q ≡ ~ (p ↔ q) without using a truth table.
So how would this be done?
Answers
I'll use text forms of the logical symbols because copying/pasting on mobile is annoying.
⊕ = XOR
∨ = OR
∧ = AND
~ = NOT
↔ = IFF (meaning "if and only if")
→ = IMP (short for "implies")
and for logical equivalence I'll use a regular equal sign.
Then
p XOR q = (p OR q) AND NOT (p AND q)
(a) By definition of XOR, we have
p XOR p = (p OR p) AND NOT (p AND p)
= p AND NOT p
and this is tautologically FALSE.
(b) To rewrite XOR, first distribute the NOT over the AND using DeMorgan's law:
NOT (p AND q) = NOT p OR NOT q
Then we can interchange the AND and ORs using their respective distributive properties. That is,
p XOR q = (p OR q) AND (NOT p OR NOT q)
= (p AND (NOT p OR NOT q)) OR (q AND (NOT p OR NOT q))
= ((p AND NOT p) OR (p AND NOT q)) OR ((q AND NOT p) OR (q AND NOT q))
Both (p AND NOT p) and (q AND NOT q) are tautologically FALSE, so the truth value of either OR depends only on the other statements, so we have
p XOR q = (p AND NOT q) OR (q AND NOT p)
Recall the definition of IFF:
p IFF q = (p IMP q) AND (q IMP p)
Also recall that IMP is logically equivalent to
p IMP q = p OR NOT q
Then we have
p IFF q = (p OR NOT q) AND (q OR NOT p)
so that its negation is
NOT (p IFF q) = NOT ((p OR NOT q) AND (q OR NOT p))
= NOT (p OR NOT q) OR NOT (q OR NOT p)
= (NOT p AND q) OR (NOT q AND p)
The statements in bold match, so p XOR q is indeed equivalent to NOT (p IFF q).
A triangular-shaped section of grass is shown.
8 yards
9 yards
Enter the area of the section of grass, in square yards.

Answers
9 x 8 = 72
72 divide by 2 = 36
area = 36 square yards
Answer:
36 square yards
Step-by-step explanation:
The area of the triangle can be figured with the expression 1/2(b * h).
1/2(9 * 8)
Multiply 9 by 8 (operation in parentheses) to get 72.
1/2(72)
Then multiply 72 by 1/2 (which is the same as 72 divided by 2).
36 square yards
The area of this section of grass is 36 square yards.
Find the common ratio of the geometric sequence 9, -18, 36, ...
Answers
Answer:
-2
Step-by-step explanation:
Each term is being multiplied by -2.
9(-2) → -18
-18(-2) → 36
A circle is represented by the equation ( x − 1 ) 2 + ( y − 5 ) 2 = 6 . Which of the following shows the location of the center of the circle and its radius?
Answers
Answer:
The center is (1,5) and the radius is sqrt(6)
Step-by-step explanation:
( x − 1 )^ 2 + ( y − 5 )^ 2 = 6
This equation of a circle is in the form
( x − h) ^2 + ( y − k )^ 2 = r^2 where (h,k) is the center and r is the radius
The center is (1,5) and the radius is sqrt(6)
Answer:
Center of a circle ⇒ ( 1 , 5 )
Radius of a circle ⇒ √6
Step-by-step explanation:
We know that the equation of a circle is:
( x - a )² + ( y - b )² = r²
Center of a circle ⇒ ( a , b )
Radius of a circle ⇒ r
Accordingly, let us solve this problem.
( x - 1 )² + ( y - 5 )² = 6
∴ Center of a circle ⇒ ( 1 , 5 )
Radius of a circle ⇒ √6