Find the some 2/3 +1/5=
Answers
Answer:
13/15
Step-by-step explanation:
Related Questions
53 grams equals how many ounces?
Answers
Answer:
1.86952 ounces
6x2 + 14 - 10x
What is the value of the expression when x = 5?
A.
170
B.
24
C.
114
D.
864
Answers
\(\lsrge\text{Hey there!}\)
\(\mathsf{6x^2 + 14 - 10x}\\\mathsf{= 6(5)^2 + 14 - 10(5)}\\\mathsf{= 6(\bold{25}) + 14 - 10(5)}\\\mathsf{= \bold{150} + 14 - 10(5)}\\\mathsf{= \bold{164} - 10(5)}\\\mathsf{= 164 - \bf 50}\\\mathsf{= \bf 114}\\\\\large\text{Therefore, your answer is: \huge\boxed{\mathsf{Option\ C. 114}}}\huge\checkmark\)
\(\large\text{Good luck on assignment \& enjoy your day!}\)
~\(\frak{Amphitrite1040:)}\)
To force floating-point output to show the decimal point and trailing zeros, use the ________ manipulator.
Answers
To force the output to show the decimal point and trailing zeros of a decimal number use the showpoint, manipulator.
The showpoint function is a manipulator that sets the show point flag, which instructs an output stream to write a decimal point for floating-point output, even if the point is superfluous. This is done by setting the showpoint flag, which is done by setting the show point flag (only zeros appear after the decimal point). More specifically, the calls to the functions.
The distinction between a whole number and the fractional component of a number is denoted by the use of a symbol known as a "decimal point," which can be either a point or a dot. A different name for it is the Decimal Mark. The representation of the decimal point is the (.). In addition to using whole numbers, we frequently work with decimals.
Want to know more about the showpoint, manipulator visit the link which is given below;
https://brainly.com/question/14774591
#SPJ4
h(r) = 11r² - 6
g(r) = r² + 8r+ 9
Find h(-2) + g(-2)
Answers
According to the solving the function the value of h(-2) + g(-2) is 45.
What exactly is function?function is a mathematical phrase, rule, or law that establishes the relationship between an independent variable and a dependent variable (the dependent variable). Functions are used frequently in mathematics and are crucial for constructing physical links in the sciences.
According to the given information:To find h(-2) + g(-2), we need to evaluate h(r) and g(r) when r = -2, and then add the results:
h(-2) = 11(-2)² - 6 = 44
g(-2) = (-2)² + 8(-2) + 9 = 1
So, h(-2) + g(-2) = 44 + 1 = 45.
Therefore, h(-2) + g(-2) = 45.
To know more about function visit:
https://brainly.com/question/12431044
#SPJ1
Find the equation in slope-intercept form of the line with slope 2 which passes through the point (2, 8)
Answers
The equation of the line in slope-intercept form is y = 2x + 4
What is slope how to solve slope equation ?
Slope is a measure of the steepness of a line. It is defined as the ratio of the change in y-coordinates to the change in x-coordinates between any two points on the line. Slope is denoted by the letter m.
The slope formula is:
m = (y2 - y1) / (x2 - x1)
where (x1, y1) and (x2, y2) are any two points on the line.
To solve for the slope of a line given two points, you can simply plug in the coordinates into the slope formula and simplify.
The slope-intercept form of a line is y = mx + b, where m is the slope and b is the y-intercept.
We are given that the slope is 2, so we have:
y = 2x + b
To find the value of b, we can use the fact that the line passes through the point (2, 8). Substituting these values into the equation, we get:
8 = 2(2) + b
Simplifying the right-hand side, we get:
8 = 4 + b
Subtracting 4 from both sides, we get:
b = 4
So the equation of the line in slope-intercept form is:
y = 2x + 4
To know more about Slope visit :-
https://brainly.com/question/16949303
#SPJ1
what is the answer ???

Answers
Step by step
She reads 5 1/2 novels per week
We know a week is 7 days
Divide 5 1/2 by 7
Change both numbers to improper fractions
11/2 divided by 7/1
When dividing fractions you flip the divisor and multiply
11/2 x 1/7
Multiply across 11 x 1 and 2 x 7
11/14 is your answer
Problem solved!
YALL I NEED HELP PLEASE HELPP ME

Answers
Answer:
y = -2x-1
Step-by-step explanation:
hope this helps :)
Answer:
y=-2x-1
Step-by-step explanation:
so we need to find y=mx+3
y=intercept
mx=gradient
c is the y intercept where it crosses the line the y and m axis is the gradient which means the slope of the graph to find c but you need to find m so the gradient has a formula and you need to use any of the coordinates
so the formula will be y2-y1 /x2-x1 this means you do the second y from the first y from the first coordinates do the same for x and then divide them y2-y1/x2-x1=-5--3/2-1=-5+3/1 so m=-2 to look for c we do -7=-2(3)+c =-7= -6 +c
=-7+6=c =-1=c
y=mx+c
ans= y=-2x-1
ik it long lol
Robert can complete 10 inspections in 8 hours. Which represents the unit rate for the number of inspections he completes per hour?

Answers
Answer: 5 inspections in 4 hours, because if its 8 hours it would be 10 inspections
Step-by-step explanation:
Answer:
1.25
Step-by-step explanation:
10 ÷ 8 = 1.25
Which statement is true about the graphed function

Answers
i need help with this ^

Answers
Answer:
i think 13
Step-by-step explanation:
60+57=117
117/9=13
what is the value of the expression 4 2/5(-2.5)
Answers
Answer:
-0.64
Step-by-step explanation:
Given:
4[2/5(-2.5)]
Find:
Value
Computation:
⇒ 4[2/5(-2.5)]
⇒ 8 / [-12.5]
⇒ -0.64
What is the value of pi?
Answers
Answer:
3.141592653589793
Step-by-step explanation:
Normally, you only need to memorize the digits up to 3.1415926. Of course, there are more digits of pi, but you don't need to know that much digits of pi.
Answer:
3.14159265359
Step-by-step explanation:
Find the perioa
equation.
llowing
y = 2 cos(5x + 3) - 6
77
Period = [2]T
Give your answer in simplest form.
![Find the perioaequation.llowingy = 2 cos(5x + 3) - 677Period = [2]TGive your answer in simplest form.](https://i5t5.c14.e2-1.dev/h-images-qa/contents/attachments/LQPTncYwbgWAlBk9oUI1EamQ5J0oFRZg.png)
Answers
Answer:
In the equation y = 2 cos(5x + 3) - 6, we can ignore the coefficients 2 and -6 for the purposes of calculating the period because they do not change the period. They only change the amplitude (2) and vertical shift (-6) of the function.
The coefficient 5 in front of x inside the cosine function affects the period of the function. It is a horizontal compression/stretch of the graph of the function.
The period of the basic cosine function, y = cos(x), is 2π. When there is a coefficient (let's call it b) in front of x, such as y = cos(bx), the period becomes 2π/b.
So, in your case, b = 5, so the period T of the function y = 2 cos(5x + 3) - 6 is:
T = 2π / 5
This is the simplest form for the period of the given function.
eometry Part 4 - Lesson 1 Assessment - EDCP.MA004.D
T
1
2 3 4 5
1
of 5
Save
What is the area of a sector of a circle with a central angle of measure 180° and whose radius is 5
cm? Leave your answer in terms of I.
○
o
ле?
o com
35
O 2 cm
Answers
Answer: it would be 34
Step-by-step explanation:
The standard deviation of returns averages28 percent. The options mature in 5 years and the risk-freerate is 2.36 percent. What is the value of e−Rt?
Answers
The value of e⁻ᴿᵗ is 0.8886 for the given the standard deviation of returns averages 28 percent with the options mature in 5 years and the risk-free rate is 2.36 percent.
So, the correct option is option ( C).
Exponential function is characterized by rapid increase (as in size or extent) and an exponential growth rate.
We have given that,
standard deviation of returns averages = 28%
options mature, t = 5 years and
Risk-free rate , R = 2.36 percent.
we have to calculate the value of e−Rt.
Exp(-Rt) = e⁻⁽²·³⁶⁾⁵
use exp(value) function to find the value :
=exp(-5 × 2.36%)
= exp(- 0.118 )
=0.8886
Hence, the the value of e⁻ᴿᵗ is 0.8886.
To learn more about Exponential function, refer:
https://brainly.com/question/9330192?
#SPJ4
Complete question:
I. M. Greedy has been granted options on 500,000 shares. The stock is currently trading at $48 a share and the options are at the money. The standard deviation of returns averages 28 percent. The options mature in 5 years and the risk-free rate is 2.36 percent. What is the value of e-rt?
a) .7087
b) .8476
c) .8886
d) .6087
e) .7952
Find all values of m for which the equation has two real solutions.
3x² + 7x - (m + 1) = 0
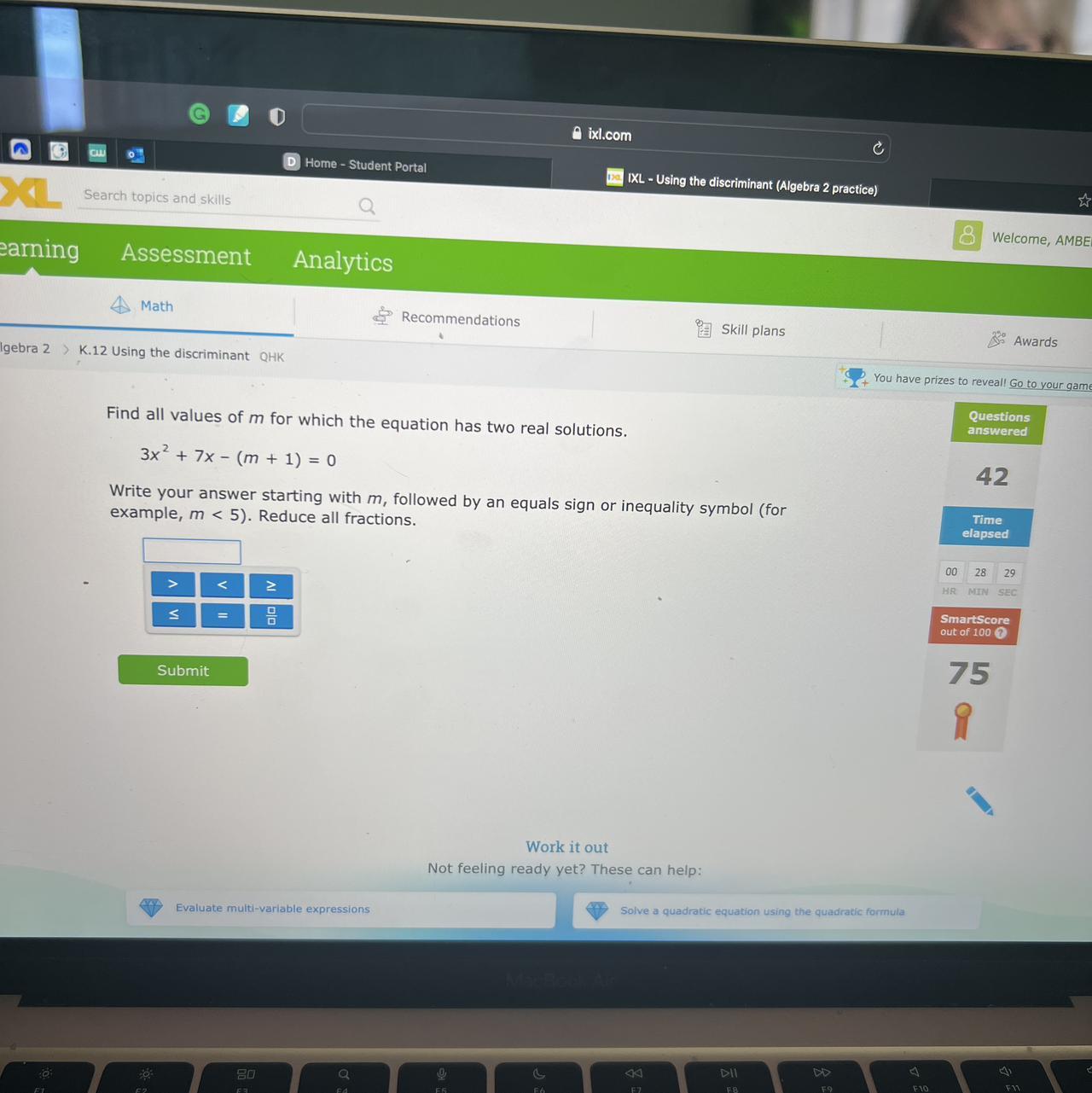
Answers

Answer:
m > - 5 1/12----------------------
Given is the quadratic equation.
A quadratic equation has two real solutions if the discriminant is positive.
Set inequality and solve for m:D = b² - 4ac, where a = 3, b = 7, c = - (m + 1)D = 7² + 4*3*(m + 1) 7² + 4*3*(m + 1) > 049 + 12m + 12 > 012m + 61 > 012m > - 61m > - 61/12 m > - 5 1/12PLEASE HELP FAST !!!!!!!!
Identify the equation that represents a quadratic relationship

Answers
Answer:
Step-by-step explanation:
Quadratic means squared equation so
the first one is your answer.
y= 4x²
PLEASE HELP ILL GIVE THANKS, 5.0, AND BRAINLIEST TO WHOEVER ANSWERS CORRECTLY PLEASE HELP AND ILL GIVE A HIGH POINT COUNT
Sophie bikes at a speed of s miles per hour. Her friend Rana is slower, her biking
speed is only r miles per hour.
Tomorrow both girls are planning to spend 3 hours biking. How much longer will be
Sophie's route?
Answers
Answer:
FOR THIS PROBLEM I GOT..... (20/r)-(20/s)Step-by-step explanation:
pls brainlest mee
currently needing to slove this problem

Answers
Check the picture below.
so it has a center at (2 , -5) and it passes through (4 , -4), now we could either the distance formula to get the "red" radius or just the pythagorean theorem, hmmm let's use the pythagorean theorem
\(\begin{array}{llll} \textit{using the pythagorean theorem} \\\\ c^2=a^2+o^2\implies c=\sqrt{a^2 + o^2} \end{array} \qquad \begin{cases} c=hypotenuse\\ a=\stackrel{adjacent}{2}\\ o=\stackrel{opposite}{1} \end{cases} \\\\\\ c=\sqrt{ 2^2 + 1^2}\implies c=\sqrt{ 4 + 1 } \implies c=\sqrt{ 5 } \\\\[-0.35em] \rule{34em}{0.25pt}\)
\(\textit{equation of a circle}\\\\ (x- h)^2+(y- k)^2= r^2 \hspace{5em}\stackrel{center}{(\underset{2}{h}~~,~~\underset{-5}{k})}\qquad \stackrel{radius}{\underset{\sqrt{5}}{r}} \\\\[-0.35em] ~\dotfill\\\\ ( ~~ x - 2 ~~ )^2 ~~ + ~~ ( ~~ y-(-5) ~~ )^2~~ = ~~(\sqrt{5})^2\implies (x-2)^2 + (y+5)^2 = 5\)

Which graph represents the function f(x)=4x?
Responses
Answers
Answer:
slope = 4
points on graph = (0,0), (1,4)
Step-by-step explanation:
line going through quadrants 1 and 3. Straight line.
Hey can anyone help pls and thank you

Answers
Answer:
5x+28=7x-4
5x+32=7x
32=2x
x=16
Hope This Helps!!!
Answer:
x = 16
Step-by-step explanation:
(7x - 4) and (5x + 28) are alternate exterior angles and are congruent, so
7x - 4 = 5x + 28 ( subtract 5x from both sides )
2x - 4 = 28 ( add 4 to both sides )
2x = 32 ( divide both sides by 2 )
x = 16
What percentage of the data in a standard normal distribution lies between x = .09 and x = 1.2?
Answers
Answer:
The percentage is \(P(0.09 < \mu < 1.2 ) = 34.9\%\)
Step-by-step explanation:
From the question we are told that
The random number is \(x_1 = 0.09 \ and \ x_2 = 1.2\)
Generally the mean of standard normal distribution is \(\mu = 0\)
The standard deviation of a standard normal distribution is \(\sigma = 1\)
The percentage of the data in a standard normal distribution lies between
\(x_1 = 0.09 \ and \ x_2 = 1.2\) is mathematically represented as
\(P(x_1 < \mu < x_2 ) = P(\frac{x_1 - \mu}{\sigma } <\frac{X - \mu}{\sigma } < \frac{x_2 - \mu}{\sigma } )\)
\(P(0.09 < \mu < 1.2 ) = P(\frac{0.09 - 0}{1 } <\frac{X - \mu}{\sigma } < \frac{1.2 - 0}{1 } )\)
\(P(0.09 < \mu < 1.2 ) = P(0.09 <\frac{X - \mu}{\sigma } < 1.2 )\)
The \(\frac{X - \mu}{\sigma } = Z(The \ standardized \ value \ of \ X )\)
\(P(0.09 < \mu < 1.2 ) = P(0.09 <Z < 1.2 )\)
\(P(0.09 < \mu < 1.2 ) = P (Z < 1.2 )- P( Z<0.09)\)
From the z-table
\(P (Z < 1.2 )= 0.88493\)
\(P( Z<0.09) = 0.53586\)
So
\(P(0.09 < \mu < 1.2 ) = 0.88493 - 0.53586\)
\(P(0.09 < \mu < 1.2 ) = 0.349\)
Hence the percentage is
\(P(0.09 < \mu < 1.2 ) = 34.9\%\)
From a group of 8 people 5 will each win $1000.How many different winning groups are possible?
Answers
Answer:
6720 ways different
Step-by-step explanation:
In this case, we must calculate the different ways using the permutation formula:
nPr = n! / (n - r)!
where n is the total number of people and r would come being the group of person that you want to put together the groups, therefore n = 8 and r = 5
replacing:
8P5 = 8! / (8 - 5)!
8P5 = 6720
That is to say that there are 6720 ways different winning groups are possible
Which of the following is the equation of a line with a slope of -5/9
Answers
Answer:
Please help me important question in image
Please help me important question in image
Please help me important question in image
Step-by-step explanation:
Please help me important question Please help me important question in image
in image
Please help me iPlease help me important question in image
Please help me important question in image
mportant question in image
Please help me important question in image
A simple random sample of 25 is drawn from a population that is normally distributed. The sample mean is found to be 108 with a sample standard deviation, s is 10 ( population standard deviation is unknown). Construct the 95% confidence interval.?
Answers
A simple random population that is normally distributed then the 95% confidence interval is (104.02, 111.98)
In mathematics and statistics, the mean refers to the average of a set of values. The mean can be computed in a number of ways, including the simple arithmetic mean (add up the numbers and divide the total by the number of observations), the geometric mean, and the harmonic mean.
Given that,
Sample Mean = 108,
Standard deviation = 10,
Sample n = 25,
Degree of freedom = n-1
n - 1 = 25 - 1
n - 1 = 24.
t-value corresponding to 24 degrees of freedom and 95% confidence level is 1.990
Confidence Interval = Mean + or - Error margin
Error margin (E) = (t×sd)/√n
E = (1.990×10)/√25
E = 19.9/5
E = 3.98
Lower bound = mean - E
= 108 - 3.98
= 104.02
Upper bound = mean + E
= 108 + 3.98
= 111.98
Therefore,
A simple random population that is normally distributed then the 95% confidence interval is (104.02, 111.98)
To learn more about Confidence interval visit :
brainly.com/question/13646086
#SPJ4
Select the correct answer. If the parent function is f(x) = x2 and its transformed function is g(x) = 2(x − 1)2 + 2, how is the graph of g(x) transformed from the graph of its parent function? A. stretch, shift along the y–axis, and shift along the x–axis B. shift along the x–axis and the y–axis C. reflection only D. stretch along the y–axis and reflection through the origin
Answers
Answer:
A) stretch, shift along the y–axis, and shift along the x–axis.
Step-by-step explanation:
Given the parent quadratic function, f(x) = x², and the transformed function in vertex form, g(x) = 2(x - 1)² + 2:
In the vertex form, g(x) = a(x - h)² + k
where:
(h, k) = vertex
a = makes the parent function wider (0 < a < 1) or narrower (a > 1).
h = determines the horizontal translation of the parent graph:
→ Horizontal translation of h units to the right: g(x) = f(x - h), where h > 0
→ Horizontal translation of |h| units to the left: g(x) = f(x - h), where h < 0.
k = determines the vertical translation of the parent graph.
Given these definitions, it is evident that the transformed function is narrower (vertical stretch by a factor of a = 2); shifted along the y-axis (with k = 2), and horizontally shifted along the x-axis given h = 1.
Therefore, the correct answer is Option A): stretch, shift along the y–axis, and shift along the x–axis.
A - on the po boyds at a emase the foot, 1, of building. He. Observes an obje- et on the top, P of the building at an angle of ele- building of 66 Aviation of 66 Hemows directly backwards to new point C and observes the same object at an angle of elevation of 53° · 1P) |MT|= 50m point m Iame horizontal level I, a a
Answers
Answer:
53\(x_{123}\) == 134 cf
Step-by-step explanation:
A - on the po boyds at a emase the foot, 1, of building. He. Observes an obje- et on the top, P of the building at an angle of ele- building of 66 Aviation of 66 Hemows directly backwards to new point C and observes the same object at an angle of elevation of 53° · 1P) |MT|= 50m point m Iame horizontal level I, a a
The height of the building is approximately 78.63 meters.
The following is a step-by-step explanation of how to solve the problem. We'll need to use some trigonometric concepts and formulas to find the solution.
Draw a diagram of the situation described in the problem to get a better understanding of the problem. The diagram would have a right-angled triangle with angle of elevation of 66° at the bottom left vertex and another angle of elevation of 53° at the bottom right vertex. The object on top of the building is at the vertex of the triangle. Point M and I on the diagram are points on the horizontal line of sight and on the ground respectively. We can label the diagram with the following values:Angle of elevation from point A = 66°Angle of elevation from point P = 53° Length of line segment AM = h Length of line segment MP = x Length of line segment IP = y Length of line segment MT = 50m. We'll use these values to calculate the length of h, which is the height of the building.Use the tangent ratio to find x:tan 66° = h / x => x = h / tan 66°. Use the tangent ratio to find y:tan 53° = h / y => y = h / tan 53°.We know that x + y = 50, so substituting the expressions for x and y from step 3 gives:h / tan 66° + h / tan 53° = 50h = 50 tan 66° tan 53° / (tan 53° + tan 66°) ≈ 78.63 m.Therefore, the height of the building is approximately 78.63 meters.
For more such questions on height, click on:
https://brainly.com/question/28122539
#SPJ8
what is the range of this function (4, –12)
(16, –18)
(12, 18)
(1, 14
Answers
Answer:
Range = {-18; -12; 14; 18}Step-by-step explanation:
(x, y)
x in the domain
y in the range
(4, -12); (16, -18); (12, 18); (1, 14)
Domain = {1; 4; 12; 16}
Range = {-18; -12; 14; 18}
Mariam runs 7.5 miles in one hour How much distance will she cover in 3 hours
Answers
Debbie has 340 dimes in three boxes. she says that there are 4 times as many dimes in the first box as in the second and 3 times as many in the third box as in the first how much money in dollars does she have in each box
Answers
The amount of money in the first box is 8 dollars
The amount of money in the second box is 2 dollars
The amount of money in the third box is 24 dollars
Solving equationsFrom the question, we are to determine how much money there are in each box
From the given information,
"Debbie has 340 dimes in three boxes"
Let x represents the number of dimes in the first box
y represent the number of dimes in the second box
and z represent the number of dimes in the third box
Thus,
x + y + z = 340
Also, from the given information
"she says that there are 4 times as many dimes in the first box as in the second"
x = 4y
and
"3 times as many in the third box as in the first"
z = 3x
Substitute the second and third equations into the first equation
x + y + z = 340
4y + y + 3x = 340
4y + y + 3(4y) = 340
4y + y + 12y = 340
17y = 340
y = 340/17
y = 20
Substitute the value of y into the second equation
x = 4y
x = 4(20)
x = 80
Substitute the value of x into the third equation
z = 3x
z = 3(80)
z = 240
Thus,
There are 80 dimes in the first box
80 dimes = 8 dollars
There are 20 dimes in the second box
20 dimes = 2 dollars
There are 240 dimes in the third box
240 dimes = 24 dollars
Hence,
The amount of money in the first box is 8 dollars
The amount of money in the second box is 2 dollars
The amount of money in the third box is 24 dollars
Learn more on Solving equations here: https://brainly.com/question/9553406
#SPJ1