conuevert 12 min 15 sec to sec​

the first one and the last one.

The probability that the three randomly selected students will have blood types A, B and AB is given as follows:

0.00164 = 0.164%.

The parameters that are needed to calculate a probability are listed as follows:

Then the probability is calculated as the division of the number of desired outcomes by the number of total outcomes.

Hence the probability for this problem is calculated as follows:

41/100 x 1/10 x 1/25 = 0.00164 = 0.164%.

Learn more about the concept of probability at https://brainly.com/question/24756209

#SPJ1

Answer:

(n + 1)(n + 2): Number of circles that make the center group of circles

2n: Number of circles on both sides of the center group

Step-by-step explanation:

It makes sense

It was right for me

Answer:

yes

Step-by-step explanation:

12+3=5x-3+3

15=5x

15=5(3)

15=15

Find the volume of a pyramid with a square base, where the side length of the base is

19.3 ft and the height of the pyramid is 16.2 ft. Round your answer to the nearest

tenth of a cubic foo

Remember that

the volume of the pyramid is equal to

where

B is the area of the base

h is the height

step 1

Find out the area of the base

B=19.3^2

B=372.49 ft2

h=16.2 ft

substitute the given values in the formula

Answer:

8) x + 20

9) 20x

10) 20/x

11) x-20

Step-by-step explanation:

8) x + 20

9) 20x

10) 20/x

11) x-20

Answer:

\(\frac{1}{729} c^{27}\)

Step-by-step explanation:

\(\left(9c^{-9}\right)^{-3}=\frac{1}{\left(9c^{-9}\right)^3}\)

\(\frac{1}{9^3\left(c^{-9}\right)^3}\)

\(\frac{1}{729c^{-9\times 3}}\)

\(\frac{1}{729c^{-27}}\)

\(\frac{c^{27}}{729}=\frac{1}{729} c^{27}\)

The equation of a circle that is congruent to circle C and is centered at point P is (x - 5)² + (y - 1)² = 5².

In Geometry, the standard form of the equation of a circle is modeled by this mathematical equation;

(x - h)² + (y - k)² = r²

Where:

Based on the information provided, we have the following parameters for the equation of this circle:

By substituting the given parameters, we have:

(x - h)² + (y - k)² = r²

(x - 5)² + (y - 1)² = 5²

Read more on equation of a circle here: brainly.com/question/15626679

#SPJ1

Answer: Shade 6 pieces

Step-by-step explanation:

Because 2/5 of 15 is 6

Answer:

7x10 ^-10

Step-by-step explanation:

Answer:

x = 47

Step-by-step explanation:

x + 121 = 4x - 20

-3x + 121 = - 20

-3x = -141

x = 47

So, the answer is x = 47

Answer:

See below

Step-by-step explanation:

2x = 10

Divide by 2.

x = 5

x has to equal 5 to make the first statement true.

Proof:

2x = 10

2(5) = 10

10 = 10

-3x = 21

Divide by -3.

x = -7

x has to equal -7 to make the second statement true.

Proof:

-3x = 21

-3(-7) = 21

21 = 21

1/3(x) = 6

Multiply by 3 to cancel the fraction.

x = 18

x has to equal 18 to make the third statement true.

Proof:

1/3(x) = 6

1/3(18) = 6

6 = 6

-1/2(x) = -7

Multiply by -2 to cancel the fraction.

x = 14

x has to equal 14 to make the fourth statement true.

Proof:

-1/2(x) = -7

-1/2(14) = -7

-7 = -7

Answer:

Y = 4

Step-by-step explanation:

5x-y=54

50-y=54

circle y and subtract 50 from itself then subtract 50 from 54.

after subtracting you should have y=4

Answer:

-15

Step-by-step explanation:

4b - 3(6 + 2b) = 12

4b - 18 - 6b = 12

4b - 6b = 12 + 18

-2b = 30

b = 30/-2

b = -15

Answer:

Step-by-step explanation:

Refer to attached

Sum of frequencies = 20

Sum of frequency x midpoint product = 610

Mean height

Based on the given data set, the mean height of a plant is 30.5 centimeters.

Given information:

The table shows the height of plant in centimeters and the number of plant that fall under each range.

The midpoints of ranges of height will be,

5, 15, 25, 35, 45, and 55.

The respective frequency of plants is,

1, 4, ,7, 2, 3, and 3.

Now, the mean height of the plants will be calculated as,

\(\bar x=\dfrac{5\times1+15\times4+25\times7+35\times2+45\times3+55\times3}{1+4+7+2+3+3}\\\bar x=\dfrac{610}{20}\\\bar x=30.5\)

Thus, we conclude that  the mean height of the plants is 30.5.

for more details, refer to the link:

https://brainly.com/question/25744307

Answer:

For $1.00 you can get 8 pencils

Step-by-step explanation: 24/3= 8

Can you give me Brainliest?

the answer is 10 lol  

Answer:

10

Step-by-step explanation:

The absolute value of -9 is 9

The absolute value of -1 is

9+1=10

Answer:

c

Step-by-step explanation:

Answer:

We kindly invite you to see the result in the image attached below.

The number in polar form is \(z = 3\sqrt{5}\cdot e^{i\cdot 0.352\pi}\).

Step-by-step explanation:

A complex number is represented by elements of the form \(a + i\,b\), for all \(a\), \(b\) \(\in \mathbb{R}\). The first part of the sum is the real component of the complex number, whereas the second part of the sum is the imaginary component of the complex number. The real component is located on the horizontal axis and the imaginary component on the vertical axis.

Now we proceed to present the point on the graph: (\(a = 6\), \(b = 3\))  We kindly invite you to see the result in the image attached below.

The polar form of the complex number is defined by:

\(z = r\cdot e^{i\cdot \theta}\) (1)

Where:

\(r\) - Magnitude of the complex number, dimensionless.

\(\theta\) - Direction of the complex number, measured in radians.

The magnitude and the direction of the complex number are defined by the following formulas:

Magnitude

\(r =\sqrt{a^{2}+b^{2}}\) (2)

Direction

\(\theta = \tan^{-1} \frac{b}{a}\) (3)

If we know that \(a = 6\) and \(b = 3\), then the polar form of the number is:

\(r =\sqrt{6^{2}+3^{2}}\)

\(r = 3\sqrt{5}\)

\(\theta = \tan^{-1} \frac{6}{3}\)

\(\theta \approx 0.352\pi \, rad\)

\(z = 3\sqrt{5}\cdot e^{i\cdot 0.352\pi}\)

The number in polar form is \(z = 3\sqrt{5}\cdot e^{i\cdot 0.352\pi}\).

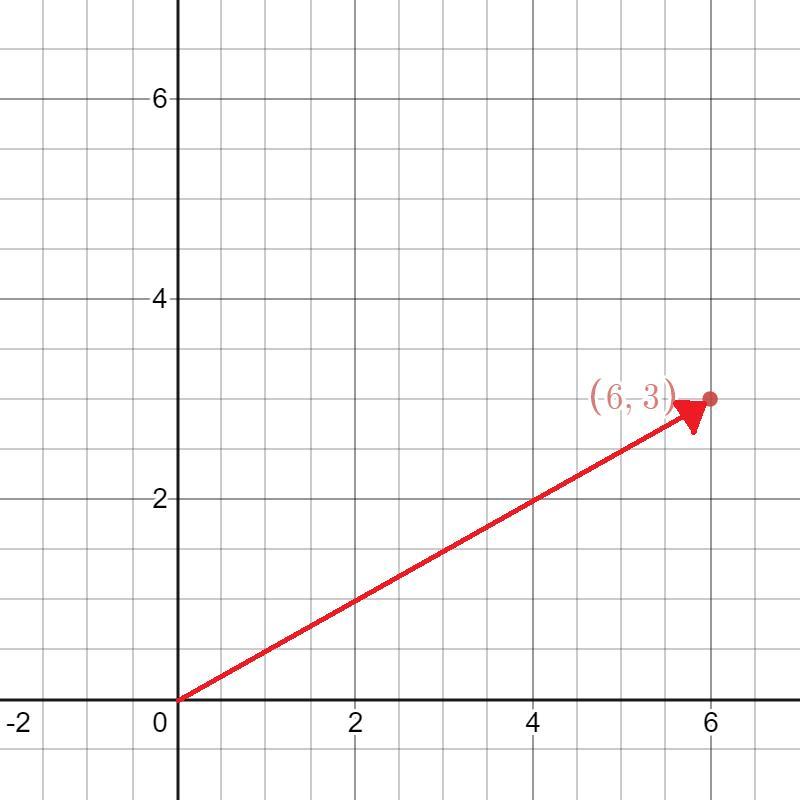
A triangle with coordinates A(1, 1), B(6, 2), C(3, 5) is translated five units left and three units down. The new coordinates are

A'' (-4, -2)

B'' (1, -1)

C'' (-2, 2

The new coordinates of the transformations solved by applying the transformation rule adequately

Translation 5 units left is subtracting 5 units to the x coordinates

A (1, 1) = A' (-4, 1)

B (6, 2) = B' (1, 2)

C (3, 5) = C' (-2, 5)

Translation 3 units down is subtracting 3 units to the y coordinates

A' (-4, 1) = A'' (-4, -2)

B' (1, 2) = B'' (1, -1)

C' (-2, 5) = C'' (-2, 2

the coordinates are the ones with double primes ''

Learn more about translation at:

https://brainly.com/question/12861087

#SPJ1

Answer:

good the idea work

the common multiple are you find the for work

The complete values are:

1. Scale factor = 1/2

DE = 17.69

AC = 6.5 unit

AB = 8.845 unit

2.  Scale factor = 2/3

UT = 11.34 unit

YZ =  12 unit

XY =  17 unit

1. In ΔACB and ΔDFE

Using Pythagoras

DE = √13² + 12²

DE = √169 + 144

DE = √313

DE = 17.69

AC = 6.5 unit

AB = 8.845 unit

and, Scale factor = 6/12 = 1/2

2. In ΔTUV and ΔXYZ

Scale factor = 8/12 = 2/3

Using Pythagoras

UT= √64 + 64

UT = 11.34 unit

So, YZ = 8  x 3/2 = 12

and, XY = 11.34 x 3/2 = 17

Learn more about Scale factor here:

https://brainly.com/question/29464385

#SPJ1

Answer:

942 square millimeters.

Step-by-step explanation:

The formula for the surface area of a cylinder is:

S = 2πr^2 + 2πrh

where S is the surface area, r is the radius, and h is the height.

Substituting the given values, we get:

S = 2 x 3.14 x 10^2 + 2 x 3.14 x 10 x 5

S = 628 + 314

S = 942

Therefore, the surface area of the cylinder is 942 square millimeters.

Answer:

Step-by-step explanation:

Given relation

Its range is the set of second values in the pairs

In the ascending order

Answer:

{-4, -2, 2}

Step-by-step explanation:

The relation is {(3, -2), (1, 2), (-1, -4),(-1, 2)}

The range is all possible y values apart from any duplicates.

y = -2, 2, -4, 2

Removing all duplicates we get.

y = -2, 2, -4

Put them in order.

y = -4, -2, 2

Best of Luck!

To find the limit of a convergent sequence, we can simply take the limit as n approaches infinity. Let's calculate the limits for each of the given sequences:

A. \(\sf\:\lim_{n \to \infty} \frac{5n}{n+7} \\\)

To find the limit, we divide the leading terms by n:

\(\sf\:\lim_{n \to \infty} \frac{5n/n}{(n+7)/n} = \frac{5}{1} = 5 \\\)

Therefore, the limit of the sequence A is 5.

B. \(\sf\:\lim_{n \to \infty} \frac{4-7n}{8+n} \\\)

Again, divide the leading terms by n:

\(\sf\:\lim_{n \to \infty} \frac{4/n - 7}{8/n + 1} = \frac{0 - 7}{0 + 1} = -7 \\\)

So, the limit of sequence B is -7.

C. \(\sf:\lim_{n \to \infty} \frac{8n - 500\sqrt{n}}{2n + 800\sqrt{n}} \\\)

Divide the leading terms by n:

\(\sf\:\lim_{n \to \infty} \frac{8 - 500\sqrt{n}/n}{2 + 800\sqrt{n}/n} \\\)

As n approaches infinity, the terms involving \(\sf\:\sqrt{n}/n \\\) tend to 0:

\(\sf\:\lim_{n \to \infty} \frac{8 - 500(0)}{2 + 800(0)} = \frac{8}{2} = 4 \\\)

Therefore, the limit of sequence C is 4.

To summarize:

A. The limit of sequence A is 5.

B. The limit of sequence B is -7.

C. The limit of sequence C is 4.

As per the given data, the number of integers in R ∩ S is 2, and the correct answer is (B) Two.

In mathematics, the intersection of two sets is a set containing all elements that are members of both sets.

In symbols, the intersection of two sets A and B is denoted by A ∩ B, and it contains all elements that belong to both A and B.

The intersection of two sets contains only the elements that are present in both sets. In this case,

R ∩ S = {x | x > 0 and x < 3}

The integers that are present in this set are 1 and 2.

Therefore, the number of integers in R ∩ S is 2, and the correct answer is (B) Two.

For more details regarding intersection, visit:

https://brainly.com/question/30722656

#SPJ1

Answer:

2:15 pm

Step-by-step explanation: