Answers
If AB is x+8 and BC is 4x-4 then he measure of AC is 24
The point where two lines meet is known as an angle.
From the given diagram;
AB + BC = 180
Given the following
AB = x + 8
BC = 4x - 4
Since the midpoint of AC is B, then: AB = BC
x + 8 = 4x - 4
x - 4x = -4 - 8
-3x = -12
x = 12/3
x = 4
Get the value of AC
AC = AB + BC
AC = x + 8 + 4x - 4
AC = 5x + 4
AC = 5(4) + 4
AC = 24
Hence the measure of AC is 24
Learn more here: https://brainly.com/question/22883501
Related Questions
Yogurt cups have a regular selling price of $0.55 each. The grocery store is
having a sale and all yogurt cups are 3 for $1. What is the markdown on a
single cup if you take advantage of the sale?
Answers
Answer:0.65$
Step-by-step explanation:
If there was no discount, the yogurt would be 1.65$
At a sale, suits were sold for $116 each. This price was 58% of a suit's original price. How much did a suit originally cost?
Answers
Answer:
The suit originally cost $200.
Step-by-step explanation:
In order to calculate the original price of the suit, you can use a rule of three considering that $116 represents 58% of the original price:
116 → 58%
x ← 100%
x=(116*100)/58
x=200
According to this, the answer is that the suit originally cost $200.
Write the negation of each of the following statements (hint: you may have to apply DeMorgan’s Law multiple times)
(a) ∼ p∧ ∼ q
(b) (p ∧ q) → r
Answers
a) Negation of ∼ p∧ ∼ q is (p V q). The original statement "∼ p∧ ∼ q" has a negation of "p V q" using DeMorgan's law of negation that states: The negation of a conjunction is a disjunction in which each negated conjunct is asserted.
b) Negation of (p ∧ q) → r is (p ∧ q) ∧ ∼r. The original statement "(p ∧ q) → r" has a negation of "(p ∧ q) ∧ ∼r" using DeMorgan's law of negation that states: The negation of a conditional is a conjunction of the antecedent and the negation of the consequent.
DeMorgan's law of negation is applied to get the negation of the given statements as shown below:(a) ∼ p∧ ∼ qNegation of the above statement is(p V q)DeMorgan's law of negation is used to get the negation of the statement(b) (p ∧ q) → rNegation of the above statement is(p ∧ q) ∧ ∼r DeMorgan's law of negation is used to get the negation of the statement.
The given statement (a) is ∼ p∧ ∼ q. The negation of the statement is obtained by applying DeMorgan's law of negation. The law states that the negation of a conjunction is a disjunction in which each negated conjunct is asserted. Hence, the negation of ∼ p∧ ∼ q is (p V q).
For the given statement (b) which is (p ∧ q) → r, the negation is obtained using DeMorgan's law of negation. The law states that the negation of a conditional is a conjunction of the antecedent and the negation of the consequent. Hence, the negation of (p ∧ q) → r is (p ∧ q) ∧ ∼r.
DeMorgan's law of negation is a fundamental tool in logic that is used to obtain the negation of a given statement. The law is applied to negate a conjunction, disjunction, or conditional statement. To obtain the negation of a statement, the law is applied as many times as required until the desired negation is obtained.
To know more about DeMorgan's law :
brainly.com/question/33354694
#SPJ11
If x, y ∈ Cn are both eigenvectors of A ∈ Mn associated with the eigenvalue λ, show that any nonzero linear combination of x and y is also right eigenvectors associated with λ. Conclude that the set of all eigenvectors associated with a
particular λ ∈ σ(A), together with the zero vector, is a subspace of Cn.
Answers
Az = λz, which means that any nonzero linear combination of x and y (such as z) is also a right eigenvector associated with the eigenvalue λ.
to show that any nonzero linear combination of x and y is also a right eigenvector associated with the eigenvalue λ, we can start by considering a nonzero scalar α. let z = αx + βy, where α and β are scalars. now, let's evaluate az:
az = a(αx + βy) = αax + βay.since x and y are eigenvectors of a associated with the eigenvalue λ, we have:
ax = λx,ay = λy.substituting these equations into the expression for az, we get:
az = α(λx) + β(λy) = λ(αx + βy) = λz. to conclude that the set of all eigenvectors associated with a particular λ, together with the zero vector, forms a subspace of cn, we need to show that this set is closed under addition and scalar multiplication.1. closure under addition:
let z1 and z2 be nonzero linear combinations of x and y, associated with λ. we can express them as z1 = α1x + β1y and z2 = α2x + β2y, where α1, α2, β1, β2 are scalars. now, let's consider the sum of z1 and z2:z1 + z2 = (α1x + β1y) + (α2x + β2y) = (α1 + α2)x + (β1 + β2)y.
since α1 + α2 and β1 + β2 are also scalars, we can see that the sum of z1 and z2 is a nonzero linear combination of x and y, associated with λ.2. closure under scalar multiplication:
let z be a nonzero linear combination of x and y, associated with λ. we can express it as z = αx + βy, where α and β are scalars.now, let's consider the scalar multiplication of z by a scalar c:cz = c(αx + βy) = (cα)x + (cβ)y.
since cα and cβ are also scalars, we can see that cz is a nonzero linear combination of x and y, associated with λ.additionally, it's clear that the zero vector, which can be represented as a linear combination with α = β = 0, is also associated with λ.
Learn more about linear here:
https://brainly.com/question/31510530
#SPJ11
I need help please

Answers
Answer: SAS
Explanation: There are two sides that are congruent and there is one vertical angle between them which are always congruent.
Anyone know what the answer to \(\sqrt{45854547452436}\) is?
Answers
Change to slope-intercept form: 3x + 12y = -60
Answers
Answer:
y=-1/4x-5
Step-by-step explanation:
Answer:
y = -1/4x - 5
Step-by-step explanation:
12y = -3x- 60
divide 12 on each side
y = - 1/4x -5
make me brainliest if answer is correct
What trig do you use cos, sin, tan and how
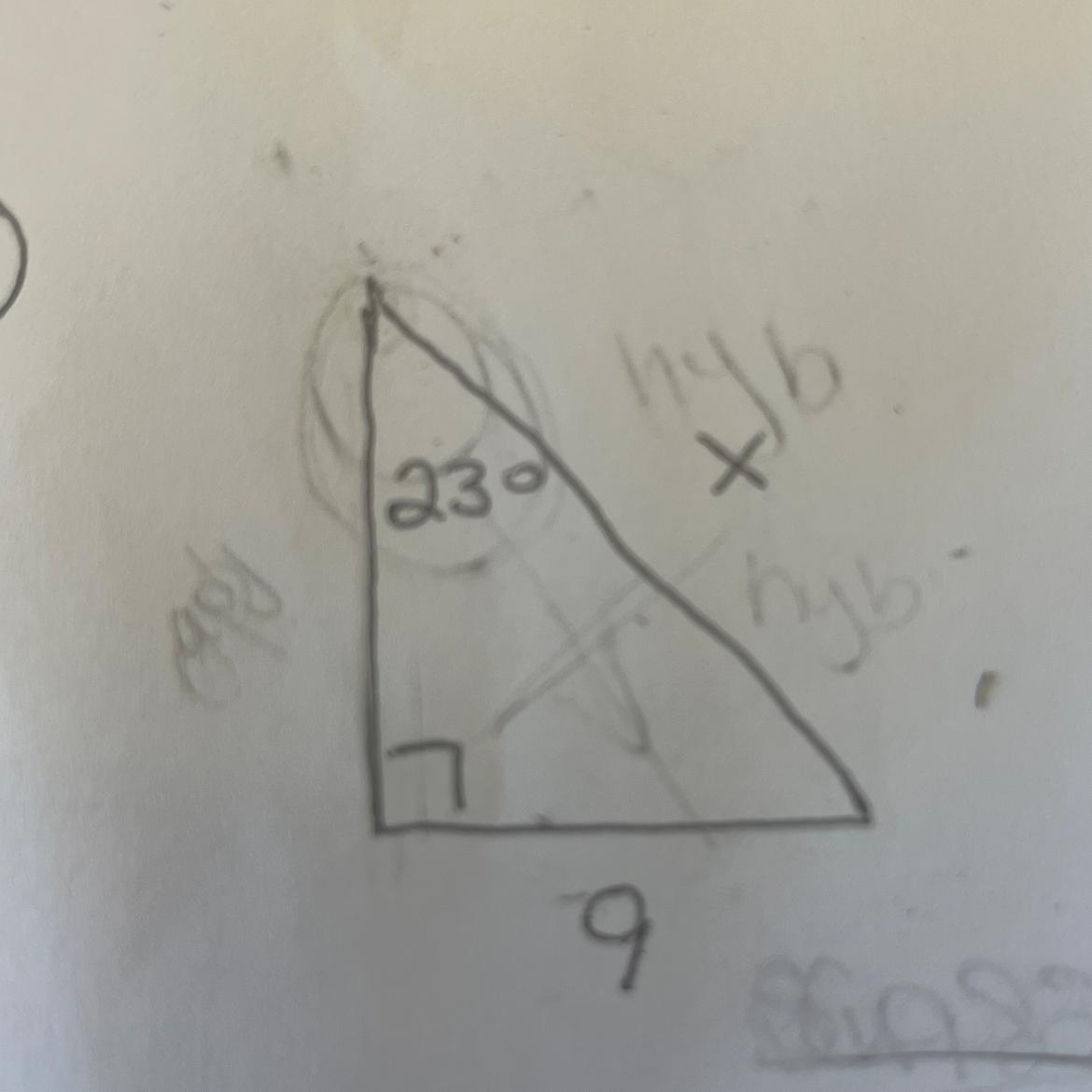
Answers
By using a right-angled triangle as a reference, the trigonometric functions and identities are derived:
sin θ = Opposite Side/Hypotenuse
cos θ = Adjacent Side/Hypotenuse
tan θ = Opposite Side/Adjacent Side
sec θ = Hypotenuse/Adjacent Side
cosec θ = Hypotenuse/Opposite Side
cot θ = Adjacent Side/Opposite Side
Please help me ASAP! Thank you! 15 points
Simon and his friends have 27 pieces of candy. They split them up evenly and each person get 9 pieces. How many people are there? Select the correct equation and solve for p.
A. 27 = 9p; p = 3
B. 9 + p = 27; p = 18
C. p/27 = 9; p = 3
D. 9 = 27 - p; p = 18
Answers
Answer:
\( \sf \: a) \: 27 = 9p \: ; p = 3\)
Step-by-step explanation:
Given information,
→ Simon have 27 pieces of candy.
→ Each person will get 9 pieces.
Now we have to,
→ Find the required equation.
The equation will be,
→ 27 = 9p
→ 9p = 27
=> As each person (p) gets 9 pieces.
Then the value of p will be,
→ 9p = 27
→ p = 27 ÷ 9
→ [ p = 3 ]
Hence, option (a) is correct.
The following figures are not drawn to scale but AB and CD are straight lines.
Find x.

Answers
x = 30
Step-by-step explanation:
2x + 90° + x = 180°
3x + 90° = 180°
3x = 90°
x = 90/3
x = 30
Answer:
30
Step-by-step explanation:
90° + x + x+ x = 180 {Straight line angles}
90 + 3x = 180
Subtract 90from both sides
3x = 180- 90
3x = 90
Divide both sides by 3
x = 90/3
x = 30
True or false. If the graphs of f an g intersect midway between x=a and x=b, then the integral from a to b [f(x)-g(x)]dx= 0. Explain
Answers
The given statement "If the graphs of f an g intersect midway between x=a and x=b, then the integral from a to b [f(x)-g(x)]dx= 0" is a FALSE statement.
The given statement "If the graphs of f an g intersect midway between x=a and x=b, then the integral from a to b [f(x)-g(x)]dx= 0" is a FALSE statement. What is meant by the word intersect? The point where two lines cross each other is known as the intersection point. We know that in mathematics, two lines can intersect at a single point or may never intersect at all.
Let us consider two different functions f(x) and g(x) and assume that they intersect midway between x=a and x=b as shown in the below figure.Then, we can conclude that at the point of intersection, both the function has the same value. That is, f(c) = g(c).Where c is the value of the point of intersection. To check whether the given statement is true or false, let us find the integral from a to b of [f(x) - g(x)] dx.The area of the shaded region in the above graph is equal to the integral of [f(x) - g(x)] from a to b. Since the graphs of f and g intersect at c, then both the function has the same value at x = c.
This implies that f(c) = g(c).From the graph, it is clear that f(x) > g(x) when x is between a and c and g(x) > f(x) when x is between c and b. That is, [f(x) - g(x)] > 0 for x in [a, c) and [g(x) - f(x)] > 0 for x in (c, b]. Therefore, the integral from a to b of [f(x) - g(x)] dx is not equal to zero. Thus, the given statement "If the graphs of f an g intersect midway between x=a and x=b, then the integral from a to b [f(x)-g(x)]dx= 0" is a FALSE statement.
Learn more about Integral
brainly.com/question/18125359
#SPJ11
A rectangle has an area of 126 square yards and a width of 7 yards.
What is the length of the rectangle?
Answers
The equation for the area of a rectangle is width * length = area. You can revers that to get area / width = length. 126 / 7 = 18. You can double check by multiplying 7 by 18 and getting 126.
Josh drew the rectangle below and then reflected it over C D to form Rectangle A'B'C'D'. He argues that the image of the rectangle will be in the same location as the original rectangle was. His classmate, Raquel, thinks that it wil not be. Who is correct?
Answers
Answer: Raquel
Step-by-step explanation: If you’re reflecting then obviously it’s going to be in a new spot because it flips to somewhere new
What are the advantages of graphing calculators?.
Answers
The main advantage of using a graphic calculator is that it speeds up the process of solving more difficult arithmetic problems for students.
What is a Graphing Calculator?A graphing calculator, often known as a graphics calculator or graphic display calculator, is a portable computer that can plot graphs, solve many equations at once, and carry out other variable-related activities. The most common graphing calculators are programmable calculators, which let the user design unique programs, generally for scientific, technical, or educational purposes. They feature big screens that show computations and several lines of text.
What is the laboratory usage of such calculators?Numerous graphing calculators may be connected to sensors such as accelerometers, electronic thermometers, pH meters, weather stations, decibels, light meters, and other devices to operate as data recorders and as monitoring, polling, and communication tools with teachers. The use of such data in student laboratory tasks improves their understanding of math, particularly statistics and mechanics.
To know more about calculators click the following link
https://brainly.com/question/17054946
#SPJ4
How many times is it necessary to fold repeatedly in half one sheet of paper of thickness
0.1 mm to reach the thickness of the layer at least 1 m?
1) 60
2) 140
3) 16
(4) 14
5) 10000
Answers
There are 780 registered voters in Cloud County. In the last election, 40% of the registered voters actually voted. How many people in Cloud County voted in the last election?
Answers
If a quadrilateral is a rhombus, what must also be true?
O All angles are congruent.
O No angles are congruent.
O Opposite sides are parallel.
0 Opposite sides are not parallel.

Answers
Edge 2022
Step-by-step explanation:
what is the length of this line? khan academy - Distance between two points
Answers
Answer:
D = √[(x2 - x1)² + (y2 - y1)²]
Step-by-step explanation:
Given a right-angled triangle, with sides: Hypotenuse D, and opposites (x2 - x1) and (y2 - y1).
Then by Pythagoras theorem, the square of the hypotenuse is equal to the sum of the squares of the opposite side.
That is,
D² = (x2 - x1)² + (y2 - y1)²
Now, taking square roots of both side, we have the formula for the distance or length D.
D = √[(x2 - x1)² + (y2 - y1)²]
Choose the best equation to represent the problem: Misha recently measured the height of each member of her family. She found out that her dad is 72 inches tall. Her younger brother is exactly half of her dad’s height. How tall is Misha’s younger brother?
72 x 1/2
72 + 1/2
72 x 2
72 - 1/2
Answers
The height of Misha’s younger brother is 72 x 1/2
Equation
An equation is an expression that can be used to show the relationship between two or more numbers and variables.
Given that Misha brother is exactly half of her dad’s height. Hence:
Misha brother height = 72 * 1/2
The height of Misha’s younger brother is 72 x 1/2
Find out more on Equation at: https://brainly.com/question/2972832
Answer:
The height is 72 x 1/2
Step-by-step explanation:
I'm sorry if I did it incorrect, I'm just trying to get points and brainliest :)
as part of video game, the point (5,2) is rotated counterclockwise about the origin through an angle of 5 degrees. find the new coordinates of this point
Answers
The new coordinates of the point (5, 2) after rotating counterclockwise about the origin through an angle of 5 degrees are approximately (4.993, 2.048).
To find the new coordinates of the point (5, 2) after rotating counterclockwise about the origin through an angle of 5 degrees, we can use the rotation formula:
x' = x * cos(theta) - y * sin(theta)
y' = x * sin(theta) + y * cos(theta)
Where (x, y) are the original coordinates, (x', y') are the new coordinates after rotation, and theta is the angle of rotation in radians.
Converting the angle of rotation from degrees to radians:
theta = 5 degrees * (pi/180) ≈ 0.08727 radians
Plugging in the values into the rotation formula:
x' = 5 * cos(0.08727) - 2 * sin(0.08727)
y' = 5 * sin(0.08727) + 2 * cos(0.08727)
Evaluating the trigonometric functions and simplifying:
x' ≈ 4.993
y' ≈ 2.048
KLnow more about coordinates here;
https://brainly.com/question/32836021
#SPJ11
-18 = -3 + x THANK YOU SOOOOOO MCHOOOO
Answers
Answer:
\(\huge\boxed{x=-15}\)
Step-by-step explanation:
\(-18=-3+x\qquad|\text{add 3 to both sides}\\\\-18+3=-3+3+x\\\\-15=x\to x=-15\)
X and y are normal random variables with e(x) = 2, v(x) = 5, e(y) = 6, v(y) = 8 and cov(x,y)=2. determine the following: e(3x 2y) (2 points) v(3x 2y) (4 points) find p(3x 2y>20) (4 points)
Answers
The result for the given normal random variables are as follows;
a. E(3X + 2Y) = 18
b. V(3X + 2Y) = 77
c. P(3X + 2Y < 18) = 0.5
d. P(3X + 2Y < 28) = 0.8729
What is normal random variables?Any normally distributed random variable having mean = 0 and standard deviation = 1 is referred to as a standard normal random variable. The letter Z will always be used to represent it.
Now, according to the question;
The given normal random variables are;
E(X) = 2, V(X) = 5, E(Y) = 6, and V(Y) = 8.
Part a.
Consider E(3X + 2Y)
\(\begin{aligned}E(3 X+2 Y) &=3 E(X)+2 E(Y) \\&=(3) (2)+(2)(6 )\\&=18\end{aligned}\)
Part b.
Consider V(3X + 2Y)
\(\begin{aligned}V(3 X+2 Y) &=3^{2} V(X)+2^{2} V(Y) \\&=(9)(5)+(4)(8) \\&=77\end{aligned}\)
Part c.
Consider P(3X + 2Y < 18)
A normal random variable is also linear combination of two independent normal random variables.
\(3 X+2 Y \sim N(18,77)\)
Thus,
\(P(3 X+2 Y < 18)=0.5\)
Part d.
Consider P(3X + 2Y < 28)
\(Z=\frac{(3 X+2 Y-18)}{\sqrt{77}}\)
\(\begin{aligned} P(3X + 2Y < 28)&=P\left(\frac{3 X+2 Y-18}{\sqrt{77}} < \frac{28-18}{\sqrt{77}}\right) \\&=P(Z < 1.14) \\&=0.8729\end{aligned}\)
Therefore, the values for the given normal random variables are found.
To know more about the normal random variables, here
https://brainly.com/question/23836881
#SPJ4
The correct question is-
X and Y are independent, normal random variables with E(X) = 2, V(X) = 5, E(Y) = 6, and V(Y) = 8. Determine the following:
a. E(3X + 2Y)
b. V(3X + 2Y)
c. P(3X + 2Y < 18)
d. P(3X + 2Y < 28)
the carrying capacity for a population of elk is 52. there are currently 33 elk in the population. the intrinsic growth rate of elk is 0.2 per year. how many elk will be in the herd one year from now? round to a whole number, up or down (we will accept either)
Answers
There will be 38 elk in the herd one year from now, rounded to a whole number.
To solve the given problem, we must use the formula for exponential growth or decay.
We'll use the following formula:
N = N0ert
Where,N is the final population;
N0 is the initial population;
r is the intrinsic growth rate;
e is the base of the natural logarithm; and
t is the time (in years).
According to the problem, the carrying capacity for a population of elk is 52, and there are currently 33 elk in the population.
The intrinsic growth rate of elk is 0.2 per year. We need to find out how many elk will be in the herd one year from now, rounded to a whole number.
Using the formula above, we can write:
N = 33e0.2 × 1= 37.98 ≈ 38
Therefore, there will be 38 elk in the herd one year from now, rounded to a whole number. Answer: 38.
for such more question on whole number
https://brainly.com/question/9879870
#SPJ11
Fine the value of x
a.) 40.2
b.)33.1
c.) 38.4
d.) 36.6
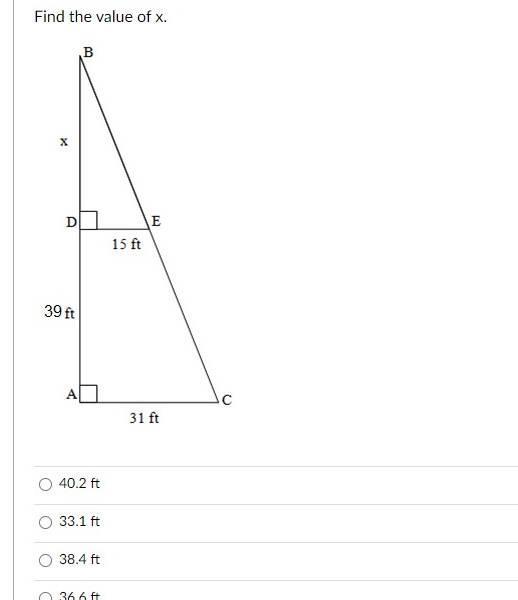
Answers
Answer: The value of x is 38.4
Answer:D
Step-by-step explanation:

the product of two numbers is 5070 and their LCM is 390 find the GCD of two numbers
Answers
\(\\ \sf\Rrightarrow Product=LCM(GCD)\)
\(\\ \sf\Rrightarrow 5070=390GCD\)
\(\\ \sf\Rrightarrow GCD=5070/390\)
\(\\ \sf\Rrightarrow GCD=13\)
Key words:-
LCM=Lowest common multipleGCD=Greatest common divisorWrite the equation of the line that passes through the points (-6,-1) and (-4,2). Put your answer in fully simplified point-slope form, unless it is a vertical or horizontal line.
Answers
\((\stackrel{x_1}{-6}~,~\stackrel{y_1}{-1})\qquad (\stackrel{x_2}{-4}~,~\stackrel{y_2}{2}) ~\hfill \stackrel{slope}{m}\implies \cfrac{\stackrel{rise} {\stackrel{y_2}{2}-\stackrel{y1}{(-1)}}}{\underset{run} {\underset{x_2}{-4}-\underset{x_1}{(-6)}}} \implies \cfrac{2 +1}{-4 +6} \implies \cfrac{ 3 }{ 2 }\)
\(\begin{array}{|c|ll} \cline{1-1} \textit{point-slope form}\\ \cline{1-1} \\ y-y_1=m(x-x_1) \\\\ \cline{1-1} \end{array}\implies y-\stackrel{y_1}{(-1)}=\stackrel{m}{\cfrac{3}{2}}(x-\stackrel{x_1}{(-6)}) \implies {\large \begin{array}{llll} y +1= \cfrac{3}{2} (x +6) \end{array}}\)
Tom has $5.05 in quarters and dimes. How many coins are quarters if the total
number of coins is 28? Try creating a system of equations and solving this by either
substitution or elimination.
quarters
Help due by 8 am tm
Answers
Answer: The answer would be 7.03
Step-by-step explanation: cause you gotta get the 28 in there i hope im right
Answer:
15 quarters
Step-by-step explanation:
Assuming q = quarters and d = dimes...
First, set up the equations. q + d = 28 since we have 28 coins, and the latter is because a quarter is worth $0.25 and a dime is worth $0.1 - totaling $5.05.
q + d = 28
.25q + .10d = 5.05
Try to get one of the variables equal to the equation above. In this case, I tried to get the "q" variables equal to each other.
q + d = 28
4(.25q + .10d) = 4(5.05)
After this, you "subtract" the two equations from each other. Remember that d = 1d, and 1 - .4 = .6
q + d = 28
q + .4d = 20.20
Divide both sides by .6 to get d by itself.
.6d = 7.8
There are 13 dimes.
d = 13
Subtract 13 from the total coins (28) to get the amount of quarters.
28 - 13 = 15
13 dimes, 15 quarters. Hope this helped!
can somebody help with step by step

Answers
Answer:
the range is 18.5
Step-by-step explanation:
14, 14, 15, 15, 15, 16, 16, 16, 17, 17, 17, 17, 17, 18, 18, 18, 18, 18, 18, 19, 19, 19, 19, 20, 20, 20, 20, 20, 21, 21, 22, 22, 22, 23, 24, 25, 26, 27.
Then take 1 off of the back and then take one from the front. (continue this until you find your answer)
If I am wrong feel free to yell at me and report me.
C = c0 + c1YD T = t0 + t1Y YD = Y – T
G and I are both constant. Assume that t1 is between 0 and 1.
Solve for taxes in equilibrium.
Suppose that the government starts with a balanced budget and that there is a drop in c0.
What happens to Y? What happens to taxes?
Suppose that the government cuts spending in order to keep the budget balanced. What will be the effect on Y? Does the cut in spending required to balance the budget counteract or reinforce the effect of the drop in c0 on output? (Don’t do the algebra. Use your intuition and give the answer in words.)
Answers
To solve for taxes in equilibrium, we can start by substituting the given equations into the equation for YD:
YD = Y - T
Since C and T are constant, we can write:
YD = (c0 + c1YD) - (t0 + t1Y)
Now, we can rearrange the equation to isolate YD:
YD = c0 + c1YD - t0 - t1Y
Simplifying further:
YD - c1YD = c0 - t0 - t1Y
Factoring out YD:
YD(1 - c1) = c0 - t0 - t1Y
Dividing both sides by (1 - c1):
YD = (c0 - t0 - t1Y) / (1 - c1)
Now, let's analyze the effects of a drop in c0. If c0 decreases, it implies that consumption decreases. As a result, YD will decrease, leading to a decrease in Y. Taxes will also decrease because they are determined by YD.
If the government cuts spending to balance the budget, it will lead to a decrease in G. This decrease in spending will reduce Y and further decrease YD. However, the impact on Y will depend on the magnitude of the cut in spending.
If the cut in spending is significant, it can counteract the decrease in output caused by the drop in c0. On the other hand, if the cut in spending is small, it may reinforce the effect of the drop in c0 on output. The overall effect on Y will depend on the relative magnitudes of the changes in c0 and G.
A drop in c0 will decrease both Y and taxes in equilibrium. If the government cuts spending to balance the budget, the effect on Y will depend on the magnitude of the cut in spending relative to the decrease in c0.
To know more about equilibrium , visit ;
https://brainly.in/question/7169549
#SPJ11
The cut in spending required to balance the budget counteracts the effect of the drop in c₀ on output (Y).
In equilibrium, taxes can be solved using the given equations:
C = c₀ + c₁YD
T = t₀+ t₁Y
YD = Y – T
To find taxes in equilibrium, we need to substitute the value of YD into the equation for taxes:
T = t₀ + t₁YD
Now, let's analyze the impact of a drop in c₀ on output (Y) and taxes (T).
When c₀ decreases, it means that the intercept of the consumption function (C) decreases.
This results in a decrease in consumption at every level of income. As a result, the aggregate demand decreases, leading to a decrease in output (Y).
The decrease in output (Y) leads to a decrease in income and, consequently, a decrease in disposable income (YD).
Since taxes (T) depend on disposable income, a decrease in YD will lead to a decrease in taxes (T).
Next, let's consider the effect of a government spending cut to balance the budget.
When the government cuts spending to balance the budget, it reduces its expenditure (G) without changing its tax revenue (T).
This reduction in government spending decreases aggregate demand, which in turn reduces output (Y).
However, since the government is keeping the budget balanced, the decrease in government spending is offset by the decrease in taxes (T) that occurs as a result of the decrease in output (Y).
Therefore, the cut in spending required to balance the budget counteracts the effect of the drop in c₀ on output (Y).
Learn more about tax revenue click;
https://brainly.com/question/33488480
#SPJ4
please help me. I think I have it figured out but I just wanted to double check.

Answers
Let's consider triangle ABC
Length AB can be obtained using Pythagoras
\(\begin{gathered} AB^2=x^2+y^2 \\ AB\text{ = }\sqrt[]{x^2+y^2} \\ \end{gathered}\)Similarly, we can consider triangle ACD, so that length AD will be obtained through Pythagoras
\(\begin{gathered} AD^2=x^2+z^2 \\ AD\text{ = }\sqrt[]{x^2+z^2} \end{gathered}\)Considering triangle ABD, with BD being the hypotenuse
\(\begin{gathered} BD^2=AD^2+AB^2 \\ (y+z)^{2\text{ }}=(x^2+z^2)+(x^2+y^2\text{)} \end{gathered}\)Expanding the parentheses
\(\begin{gathered} y^2+2yz+z^2=x^2+z^2+x^2+y^2 \\ \\ y^2-y^2+z^2-z^2+2yz=2x^2 \\ 2yz=2x^2 \\ \end{gathered}\)Divide both sides by 2
\(\begin{gathered} \frac{2yz}{2}=\text{ }\frac{2x^2}{2} \\ yz=x^2 \\ \\ x^2\text{ =yz} \\ \\ x\text{ = }\sqrt[]{yz} \end{gathered}\)Option A is correct

Answer:
Let's consider triangle ABCLength AB can be obtained using PythagorasSimilarly, we can consider triangle ACD, so that length AD will be obtained through PythagorasConsidering triangle ABD, with BD being the hypotenuseExpanding the parentheses Divide both sides by 2Option A is correct
Step-by-step explanation:
